Application of Mean, Median and Mode [In Real Life] [Uses in Real Life]
Summary
TLDRThis video explores the appropriate use of mean, median, and mode in data analysis. It explains these measures of central tendency and their applications in real-life scenarios, using examples to illustrate when each is most effective. The video emphasizes the importance of data distribution and the presence of outliers in choosing the right statistical measure, offering practical tips for personal and professional decision-making.
Takeaways
- 📊 Mean, median, and mode are summary statistics used to analyze and summarize data sets.
- 🔢 The mean is the arithmetic average of all values, but it can be skewed by outliers.
- 📈 Median is the middle value in a data set and is less affected by extreme values.
- 🍦 Mode is the most frequently occurring value in a data set, useful for categorical data.
- 📚 It's important to choose the right measure of central tendency based on the data's distribution and characteristics.
- 📉 Outliers can significantly affect the mean, making it less representative of the overall data set.
- 🌾 In skewed data sets, like exam scores or agricultural output affected by drought, the median can provide a better summary.
- 🍹 For evenly distributed data, such as monthly sugar usage in a manufacturing process, the mean can be a reliable summary statistic.
- 👠 Retailers can use mode to identify the most popular shoe sizes to stock based on sales data.
- 🏠 Mode can also be applied in everyday life, such as organizing household items based on frequency of use.
- 📋 The script provides a comparison table to help decide when to use mean, median, or mode based on the data's characteristics.
Q & A
What are the three main summary statistics discussed in the video?
-The three main summary statistics discussed in the video are mean, median, and mode.
Why might the mean not be the most appropriate measure to summarize data?
-The mean might not be the most appropriate measure to summarize data when there are extreme values or outliers, as it can skew the average and provide a misleading representation of the data set.
What is the definition of the median in statistics?
-The median is the middle value in a data set when all the observations are arranged in ascending or descending order. It divides the data into two equal halves.
Can you provide an example from the script where the mean would overestimate the performance of a group?
-An example from the script is the math class where the mean score of 43 overestimates the performance of the class, as most students scored below 40, which is the passing percentage.
What is the mode and how is it useful in certain scenarios?
-The mode is the value that occurs most frequently in a data set. It is useful in scenarios where you need to identify the most common response or the most popular item, such as in consumer surveys or inventory management.
How can the median be a better choice than the mean in certain situations?
-The median can be a better choice than the mean when the data is skewed or has outliers, as it represents the middle value and is not affected by extreme data points.
What thumb rules are suggested in the video for using mean and median?
-The thumb rules suggested are: use the mean when data is evenly distributed without extreme values, when all data points are important, and there are no special circumstances affecting the data. Use the median when data is skewed or has outliers.
In what type of data set can the mode be more useful than the mean or median?
-The mode can be more useful in data sets with categorical variables or when you need to identify the most frequently occurring item or response, as it is not affected by extreme values.
What is a potential application of the mode in a retail setting?
-A potential application of the mode in a retail setting is to determine the most popular shoe size or clothing size to stock more of, based on past sales data.
How can understanding the concepts of mean, median, and mode help in personal finance?
-Understanding these concepts can help in personal finance by allowing you to analyze expenses, set budgets, and monitor spending patterns, such as using the mean to budget for monthly household expenses.
What is the importance of observing the range and distribution of data when choosing a measure of central tendency?
-Observing the range and distribution of data is important because it helps determine the presence of outliers or skewness, which can influence the choice between mean, median, or mode for an accurate representation of the data.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
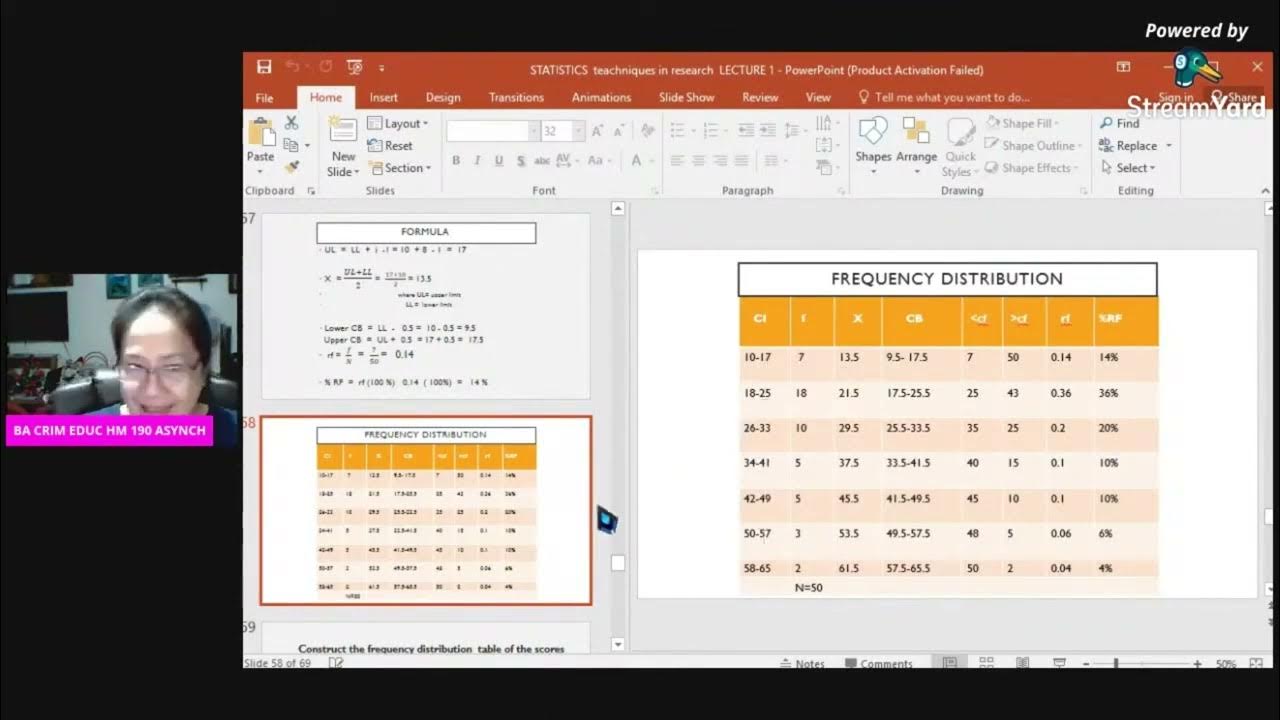
BA CRIM EDUC HM 190 VIDEO 1 2024

Statistics: The average | Descriptive statistics | Probability and Statistics | Khan Academy
Mean, Median and Mode in Statistics | Statistics Tutorial | MarinStatsLectures

Tendensi Sentral: Mean, Median, Modus

Mode, Median, Mean, Range, and Standard Deviation (1.3)

Penjelasan Mean Median dan Modus (Center of Point) Biostatistik
5.0 / 5 (0 votes)