SA04: Truss Analysis: Method of Joints
Summary
TLDRThis video explains the analysis of statically determinate trusses, covering the key concepts and methods involved in determining the forces within a truss structure. It introduces the concept of trusses, their design assumptions, and explains how axial forces are the only forces present in truss members. The Method of Joints is highlighted as the primary technique for analyzing trusses, demonstrating the application of equilibrium equations to solve for unknown forces. Through detailed examples, the script walks through the process of calculating member forces and reactions, offering a comprehensive understanding of how to analyze such structures.
Takeaways
- 😀 A truss structure consists of slender members connected at joints, carrying only axial forces (tensile or compressive), with no shear force or bending moment.
- 😀 Trusses are commonly used in roofs and bridges, and typical configurations include Howe, Warren, Pratt, and K-trusses.
- 😀 Frictionless pin connections between truss members allow rotation but no bending moment transfer at the joints.
- 😀 Applied loads must be placed at the joints, not on the members themselves, to maintain the truss's structural integrity.
- 😀 A truss is statically determinate if the number of unknown forces equals the number of equilibrium equations.
- 😀 The total number of equilibrium equations is two times the number of joints in the truss structure.
- 😀 The total number of unknown forces equals the number of truss members plus the number of support reactions.
- 😀 The Method of Joints is used to analyze statically determinate trusses by applying the principle of static equilibrium to each joint and segment.
- 😀 Free-body diagrams for each truss segment are drawn, where forces are assumed as either tensile or compressive, and equilibrium equations are applied for analysis.
- 😀 For each joint, the sum of forces in the X and Y directions must equal zero, while the moment equation is automatically satisfied due to the nature of joint forces.
- 😀 The analysis process involves solving the system of equations generated for each joint, with common methods like Gaussian elimination or direct solving for joints with fewer unknowns.
Q & A
What is a statically determinate truss?
-A statically determinate truss is one where the number of unknown forces equals the number of equilibrium equations, allowing for the calculation of the forces in each member using static equilibrium equations.
What types of loads can be carried by truss members?
-Truss members are designed to carry axial forces, which can either be compressive or tensile. There are no shear forces or bending moments acting on the truss members.
Why are truss joints assumed to be frictionless in the analysis?
-Truss joints are assumed to be frictionless to simplify the analysis, allowing the truss members to rotate freely at the joints and ensuring that no bending moments are developed at the joints.
What is the significance of the number of joints in determining the number of equilibrium equations?
-The number of equilibrium equations is two times the number of joints in the truss. This is because each joint contributes two equations: one for the sum of forces in the X direction and one for the sum of forces in the Y direction.
How do you calculate the number of unknown forces in a truss?
-The number of unknown forces in a truss is the sum of the number of truss members (each carrying an axial force) and the number of support reactions (one reaction from a roller and two reactions from a pin).
What is the Method of Joints and how is it applied in truss analysis?
-The Method of Joints involves isolating each joint of the truss and writing equilibrium equations for forces in the X and Y directions. By assuming directions for the forces and applying equilibrium, the forces in the truss members can be determined.
Why do we assume each truss member carries an axial force in the Method of Joints?
-In the Method of Joints, it is assumed that each truss member carries only an axial force (either tensile or compressive), as this is the only type of force that acts in the members. This simplifies the analysis by eliminating shear forces and bending moments.
How are the forces at joints determined in the Method of Joints?
-The forces at joints are determined by applying equilibrium equations to the free-body diagram of each joint. The sum of forces in both the X and Y directions must equal zero. If the number of unknowns is equal to the number of equations, the forces can be solved.
What does a negative value for a force indicate in the analysis?
-A negative value for a force indicates that the assumed direction for that force was incorrect. For example, if a member was assumed to be in tension but the result is negative, the member is actually in compression.
How can you simplify the solution process when analyzing a truss using the Method of Joints?
-The solution process can be simplified by looking for joints with fewer unknown forces. For example, a joint with only one or two unknown forces can be solved directly using the equilibrium equations, which reduces the complexity of the system.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

S-10 Pengenalan Truss
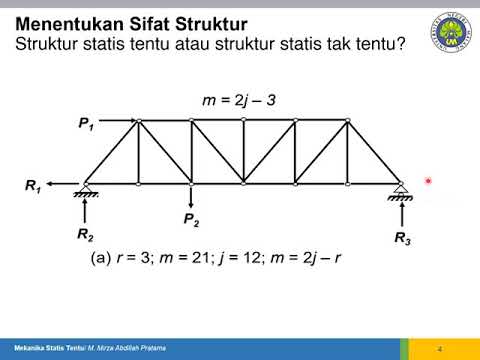
Mekanika Statis Tentu: Struktur Statis Tentu atau Struktur Statis Tak Tentu?
Understanding and Analysing Trusses

Structural Theory 1 Chapter 1 Structural Elements & Types of Structure Part 1 (with Subtitles)

Reza Adhi Fajar Engsel dan Konstruksi Gerber

Contoh Perhitungan Rangka Batang dengan Metode Potongan | Analisa Struktur Rangka Batang
5.0 / 5 (0 votes)