Persamaan Garis Lurus [Part 5] - Persamaan Garis Tegak Lurus
Summary
TLDRIn this video, Pak Beni explains the concept of perpendicular lines in geometry, specifically focusing on how to identify and calculate the equation of lines that are perpendicular to one another. He begins by reviewing the characteristics of parallel and perpendicular lines, emphasizing the 90° intersection and the condition that the product of their gradients equals -1. Through clear examples and tips, Pak Beni simplifies how to determine the gradient of a perpendicular line by flipping the values and changing the sign. The tutorial includes practical steps to find the equation of perpendicular lines, helping viewers understand and apply the concept effectively.
Takeaways
- 😀 Understanding perpendicular lines: Perpendicular lines intersect at a 90° angle, forming a right angle.
- 😀 Key feature of perpendicular lines: The product of their gradients is always -1.
- 😀 Gradients of parallel lines are the same, but gradients of perpendicular lines are inversely related and have opposite signs.
- 😀 To check if two lines are perpendicular, calculate their gradients and multiply them. If the result is -1, they are perpendicular.
- 😀 Gradients are calculated using the formula: m = (y2 - y1) / (x2 - x1). This applies to any two points on a line.
- 😀 When the gradient of one line is m1, the gradient of a line perpendicular to it is the negative reciprocal (-1/m1).
- 😀 The slope (gradient) of a line can easily be found by rearranging the line's equation into the form y = mx + c, where m is the gradient.
- 😀 Tips for finding perpendicular gradients: To find the gradient of a line perpendicular to a given line, just flip the fraction and change its sign.
- 😀 Example problem: Given a line with a gradient of 3/5, the gradient of the perpendicular line will be -5/3.
- 😀 For more complex problems, such as finding the equation of a perpendicular line passing through a specific point, use the point-slope form of the equation: y - y1 = m(x - x1).
Q & A
What is the purpose of watching this video?
-The purpose of watching the video is to help viewers understand perpendicular lines, identify if two lines are perpendicular, and determine the equation of a line that is perpendicular to another.
What is the key difference between parallel and perpendicular lines?
-Parallel lines never intersect and have the same gradient. In contrast, perpendicular lines intersect at a 90° angle, and the product of their gradients equals -1.
What is the significance of the gradient of two perpendicular lines?
-For two lines to be perpendicular, the product of their gradients must equal -1. This is a key characteristic of perpendicular lines.
How do you calculate the gradient of a line using two points?
-The gradient of a line is calculated using the formula: m = (y2 - y1) / (x2 - x1), where (x1, y1) and (x2, y2) are two points on the line.
How do you check if two lines are perpendicular based on their gradients?
-To check if two lines are perpendicular, you multiply their gradients. If the result is -1, then the lines are perpendicular.
How do you determine the gradient of a line perpendicular to a given line?
-To find the gradient of a line that is perpendicular to a given line, you invert the gradient of the original line and change its sign. For example, if the gradient is 3/5, the perpendicular gradient will be -5/3.
What is a simple tip for determining the gradient of a perpendicular line?
-A simple tip is to invert the gradient of the original line and switch its sign. For example, if the original gradient is 3/5, the perpendicular gradient will be -5/3.
What is the general form of a linear equation and how does it relate to the gradient?
-The general form of a linear equation is y = mx + c, where m is the gradient (slope) of the line and c is the y-intercept.
How do you determine the equation of a line perpendicular to a given equation?
-To determine the equation of a line perpendicular to a given equation, first find the gradient of the given line, then invert and change the sign of the gradient to find the perpendicular gradient. Afterward, use the point-slope form to find the equation of the new line.
How can you solve for the equation of a line perpendicular to another line passing through a specific point?
-First, find the gradient of the given line, then use the inverted and signed gradient to determine the perpendicular gradient. Using the point provided, apply the point-slope formula (y - y1 = m(x - x1)) to find the equation of the line.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Bangun Datar [Part 1] - Garis dan Sudut

Persamaan Garis Lurus [Part 4] - Persamaan Garis Sejajar

Bangun Datar [Part 3] - Melukis Garis, Sudut, dan Bangun Datar
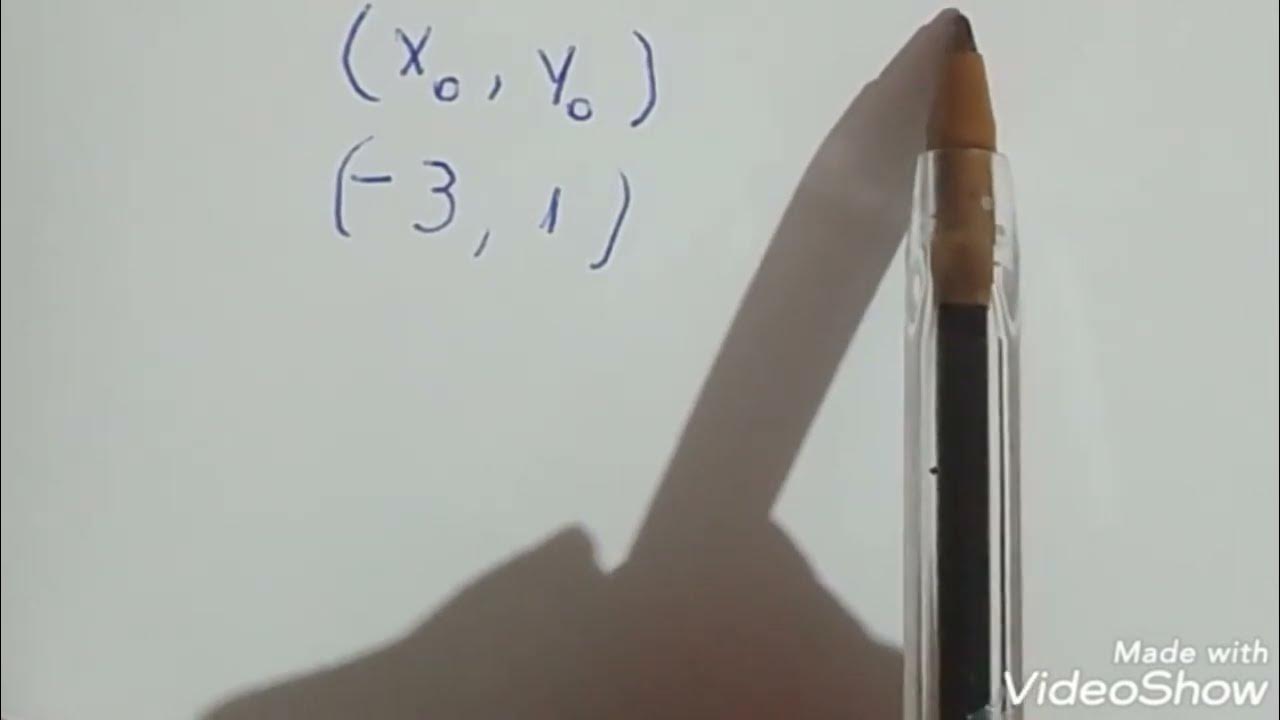
Questão de Geometria Analítica - equação da reta

Persamaan Garis Lurus [Part 2] - Gradien Garis

Proof: perpendicular lines have negative reciprocal slope | High School Math | Khan Academy
5.0 / 5 (0 votes)