Cara Cepat Integral Parsial Aljabar [LENGKAP] - Matematika Peminatan Kelas 12 - Part 5

Summary

This video explains the method of integration by parts, highlighting when it is necessary to use this technique over direct integration or substitution. Through detailed examples, it demonstrates how to solve integrals of various complexities, using the standard formula and an alternative faster approach that is rarely taught in schools. The script includes several examples that guide viewers through the step-by-step process of applying integration by parts, helping learners understand both the theory and practical application of this calculus technique. The video also introduces alternative methods for more complex integrals.

Takeaways

• 😀 Integration by parts is essential for solving integrals that cannot be easily solved using substitution or direct methods.
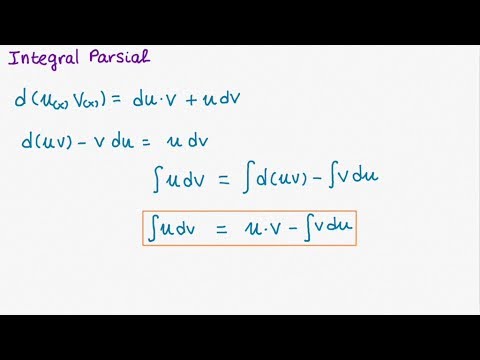
• 😀 The standard formula for integration by parts is: ∫udv = uv - ∫vdu, which helps break down complex integrals.
• 😀 An alternative and faster method to solve integration by parts involves differentiating a function to zero and then integrating it until the derivative reaches zero, alternating signs as you go.
• 😀 For the first example, ∫6x(x+2)^5 dx, the solution involves using integration by parts to break it down into more manageable steps.
• 😀 In the second example, ∫x√(2x-4) dx, integration by parts was used, with proper handling of roots and powers to find the solution.
• 😀 In the third example, ∫x/√(x+1) dx, applying integration by parts requires recognizing the form of the integral and using the proper transformation to simplify it.
• 😀 The fourth example demonstrates an alternative method for more complex integrals that involves transforming the polynomial and applying integration techniques effectively.
• 😀 For the fifth example, ∫8x(1-2x^3) dx, a method called 'tanzalin' (a quick method) is introduced to simplify the problem, using differential tables and alternating signs.
• 😀 Several methods for simplifying integrals, such as the quick differentiation and integration table method, are highlighted as faster alternatives to traditional integration by parts.
• 😀 The script concludes by providing practice problems for viewers to apply what they've learned, encouraging them to ask questions and subscribe for further learning.

Q & A

What is the main method discussed for solving integrals that cannot be solved by substitution?

The main method discussed is 'integration by parts,' which is used when substitution does not work. The formula for integration by parts is: ∫u dv = uv - ∫v du.

How is the integration by parts formula applied to the first example in the video?

In the first example, the integral of 6x(x + 2)^5 dx is solved using the integration by parts formula. We set u = 6x and dv = (x + 2)^5 dx. Then, we compute du and v, and apply the formula: uv - ∫v du.

What is the significance of the 'u' and 'dv' choices in the integration by parts method?

Choosing 'u' and 'dv' correctly is crucial in integration by parts. 'u' is usually chosen to be a function that simplifies upon differentiation, and 'dv' is chosen to be an expression that is easier to integrate. These choices dictate the ease and success of applying the formula.

What alternative approach is introduced in the video for solving integrals more quickly?

An alternative approach introduced is to differentiate a function until it reaches zero while simultaneously integrating another part of the function. This method involves alternating signs and simplifies solving complex integrals by parts.

In the second example, how is the integral of x√(2x - 4) dx handled?

In the second example, the integral of x√(2x - 4) dx is solved using integration by parts. 'u' is set as x, and 'dv' as (2x - 4)^(1/2) dx. We differentiate u and integrate dv to apply the formula successfully.

Why is the second example more complex than the first one?

The second example is more complex because it involves an expression with a square root, which requires careful handling of powers when differentiating and integrating. This introduces additional steps and makes the problem more challenging.

What is the key difference in approach between solving integrals in the video and traditional methods?

The key difference is the introduction of an alternative, faster method for solving integrals by parts, which is not typically taught in schools. This method involves differentiating until zero and integrating alternately, using a sign-alternating pattern.

How do the calculations in the example of the integral ∫x/√(x + 1) dx simplify after applying the integration by parts formula?

After applying the integration by parts formula, the calculations simplify by reducing the powers of x and integrating the remaining terms. This process involves handling the powers and ensuring proper manipulation of constants and signs.

What is the purpose of creating a table for differentiating and integrating in the alternative method?

The table helps organize the process of differentiating and integrating. It tracks the derivatives of 'u' and the integrals of 'dv' step by step, ensuring that all necessary parts are accounted for and that signs alternate correctly.

Why does the video emphasize the importance of understanding the integration by parts technique thoroughly?

The video emphasizes the importance of understanding integration by parts because it is a powerful technique for solving integrals that cannot be handled with substitution. Mastering this technique allows students to tackle a wide variety of integrals, especially those with more complex forms.