Problemas de desigualdades. Metodología y ejemplo
Summary
TLDRThis video introduces a systematic approach to solving problems involving inequalities, focusing on real-world scenarios like budgeting labor costs for assembling electronic devices. It guides viewers through key steps such as interpreting problem data, defining variables, and setting up inequalities. The video provides a clear example where a company needs to assemble 1000 devices within a budget, with different labor costs for daytime and nighttime shifts. By following the outlined steps, viewers learn how to translate a real-world problem into an algebraic inequality and solve it to determine the minimum number of devices to assemble during the day.
Takeaways
- 😀 Solving inequalities is a useful method for addressing real-world problems, especially when dealing with constraints.
- 😀 Always begin by reading the problem carefully to understand the given data and what is being asked.
- 😀 Using drawings or graphs can help clarify the problem and pinpoint important information.
- 😀 The variable selection is critical—sometimes the unknown quantity is the best candidate for the variable.
- 😀 Translating verbal descriptions into algebraic expressions is a key step in forming the inequality.
- 😀 Inequalities often arise in problems that involve limitations, such as cost constraints.
- 😀 After defining the variable, set up the inequality by breaking down the given conditions into mathematical expressions.
- 😀 The process of solving the inequality involves simplifying, grouping terms, and isolating the variable.
- 😀 When solving, always check that the inequality makes sense within the context of the problem (e.g., non-negative quantities).
- 😀 Providing a verbal interpretation of the solution is important for understanding and communicating the results clearly.
- 😀 In the given example, the company must assemble at least 500 devices during the day to meet the budget constraint for labor costs.
Q & A
What is the purpose of the video?
-The video aims to provide a methodology for solving problems involving inequalities, offering recommendations and strategies for approaching such problems.
How does the video suggest you approach solving an inequality problem?
-The video suggests a systematic approach: first, thoroughly understand the problem, then choose the correct variable, establish the inequality, and solve it algebraically.
Why is it important to read and understand the problem before solving it?
-Reading and understanding the problem ensures you identify the correct data and what is being asked, allowing you to form an appropriate strategy and mathematical expressions.
What role do drawings or graphs play in solving inequality problems?
-Drawings or graphs can help clarify situations, visualize relationships, and ensure that all relevant information is considered when translating the problem into an algebraic form.
What is the key to selecting the right variable for an inequality problem?
-The key is to choose a variable that simplifies the expression of the unknown quantities and represents the quantity that the problem is asking about.
How is the inequality established in the given example problem?
-In the example, the inequality is established by translating the cost conditions into algebraic form, using the fact that the total labor cost must be no more than 6,000 units.
What variable is chosen in the example problem, and why?
-The variable chosen is 'x,' representing the number of devices assembled during the day. This is because the problem asks about the daytime assembly and cost associated with it.
What mathematical expression represents the total cost in the example problem?
-The total cost expression is: 5x + 7(1000 - x), where 5x represents the cost for daytime labor and 7(1000 - x) represents the cost for nighttime labor.
How is the inequality solved in the example problem?
-The inequality is solved by first distributing the terms, then isolating the variable 'x,' and finally simplifying the expression to find that x must be greater than or equal to 500.
What is the final solution to the problem, and how is it interpreted?
-The final solution is that at least 500 devices must be assembled during the daytime. This is interpreted as the minimum number of units required to stay within the budget for labor costs.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Materi Berpikir Komputasional

Soal & Pembahasan SPTLDV Sistem Pertidaksamaan Linear Dua Variabel SOAL CERITA
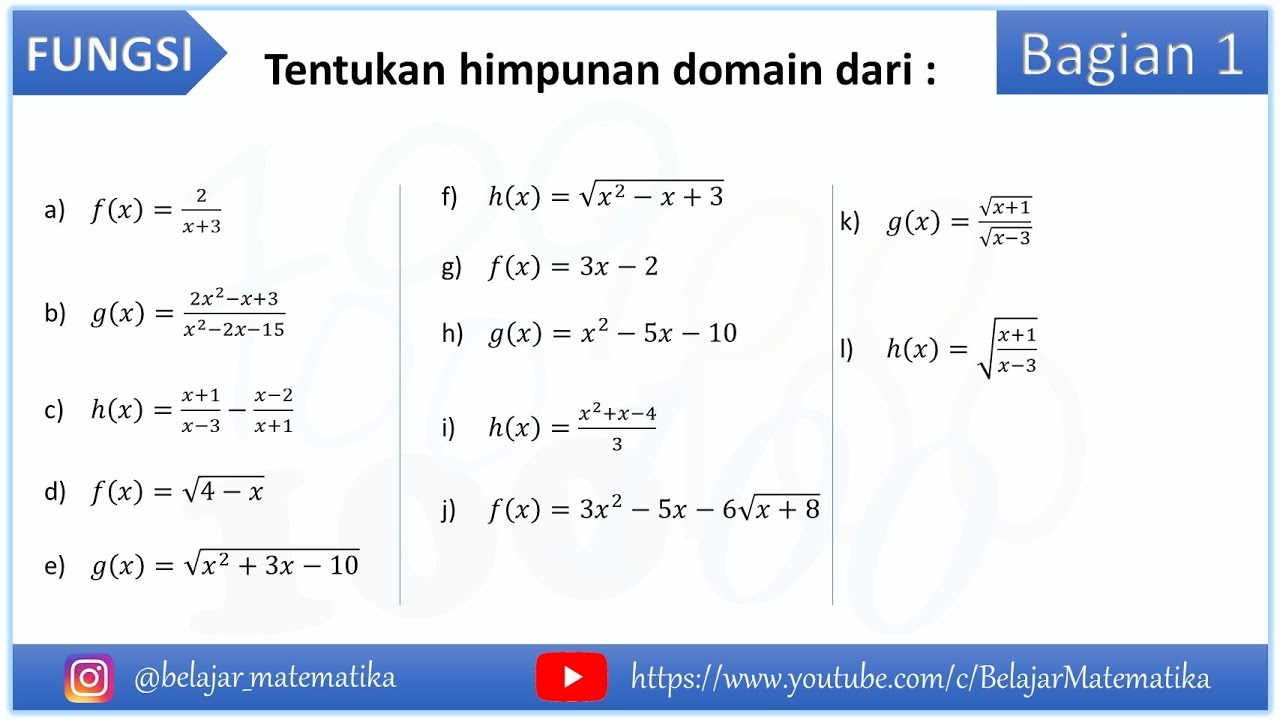
Belajar Matematika: Menentukan Domain Fungsi #1
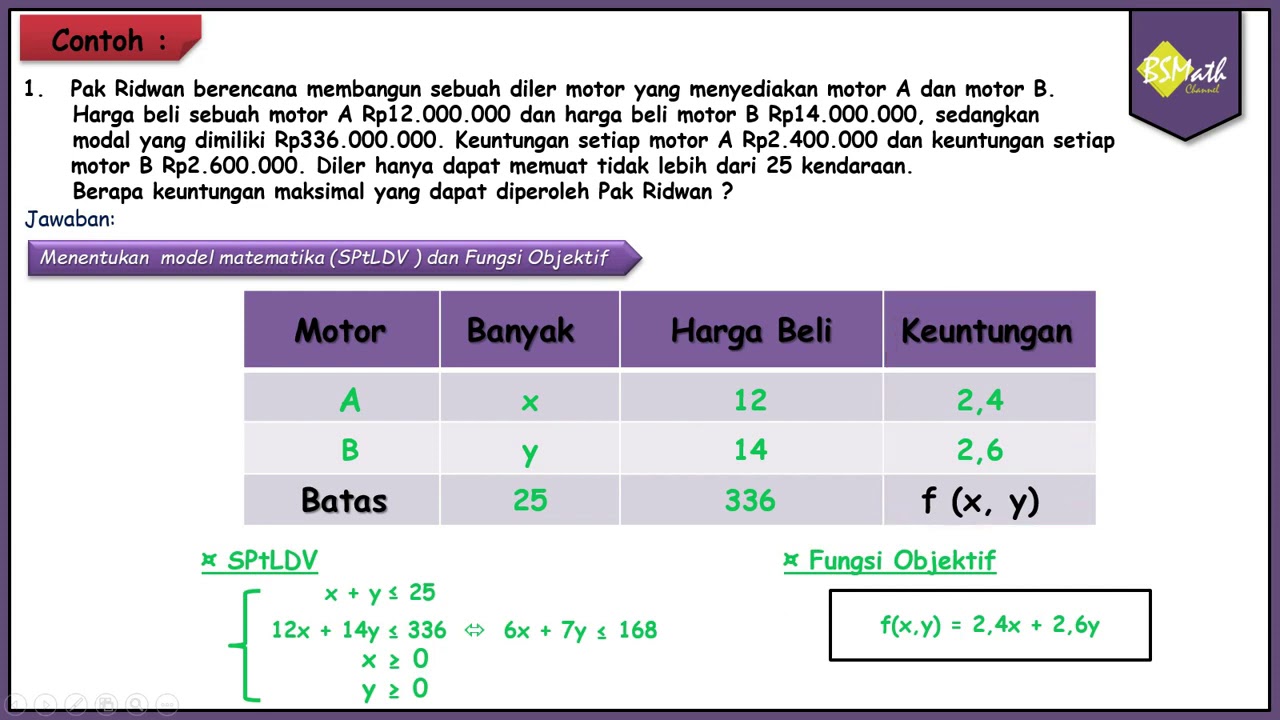
Menyelesaikan Permasalahan Program Linear Menentukan Nilai Optimum dengan Metode Uji Titik Pojok

Materi 6 - Nilai Optimum fungsi Objektif

Menentukan Nilai Optimum Menggunakan Metode Uji Titik Sudut (Uji Titik Pojok)
5.0 / 5 (0 votes)