Limit Fungsi Aljabar

Summary

In this educational video, the presenter explains how to solve algebraic limits by using various methods such as direct substitution and factoring. The video covers simple examples of limits, handling indeterminate forms like 0/0, and simplifying complex expressions involving square roots by multiplying by conjugates. The aim is to make understanding limits in algebra more accessible and break down each step of the process, offering valuable techniques to solve these problems. The video encourages learners to approach mathematical challenges with a step-by-step mindset to master the concepts.

Takeaways

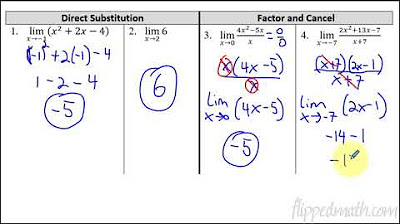
• 😀 Limit calculation involves substituting the value of x into the function when no variable x remains in the expression.
• 😀 In cases with a specific algebraic expression, substitute the limit value of x directly into the function to find the result.
• 😀 If the result is indeterminate (e.g., 0/0), further steps like factoring and simplifying the function are required.
• 😀 The first example shows that when the function doesn't involve x, the limit is simply the constant value, such as 7 in the first example.
• 😀 For a rational function, if the result yields 0/0, the function must be factored to cancel common terms before substitution.
• 😀 Factoring is essential when the form is indeterminate (like 0/0) to simplify the expression and find the correct limit.
• 😀 The transcript emphasizes that indeterminate forms require special techniques like factoring or simplifying to resolve.
• 😀 The importance of factoring is also highlighted in solving quadratic expressions by finding factors that simplify the expression.
• 😀 For limits involving square roots, multiplying by the conjugate (the 'sekan' method) helps simplify and solve the expression.
• 😀 The process concludes by substituting the simplified expression after applying factoring or conjugates to obtain the correct result.

Q & A

What is the first step in calculating the limit of an algebraic function?

The first step is to substitute the value of 'x' that the limit is approaching into the function, provided there are no indeterminate forms.

In the example where the limit approaches 3 from the constant function 7, what is the result?

The result is 7 because the function is constant and does not depend on the variable 'x'.

What happens when you encounter a 0/0 indeterminate form while calculating a limit?

When you encounter a 0/0 indeterminate form, you need to factor the expression, simplify it, and then substitute the value of 'x' again.

How is the limit of the function 2x - 4x^2 as x approaches -1 calculated?

By substituting x = -1 into the function, you get the expression 2(-1) - 4(-1)^2, which simplifies to -2 - 4 = -6.

What technique is used to resolve indeterminate forms like 0/0 in limits?

The technique used is factoring the function, simplifying it, and then substituting the limit value again.

How do you solve limits involving square roots, such as the limit of (4 - x^2) / (3 - √(x^2 + 5)) as x approaches 2?

To solve limits involving square roots, you multiply the numerator and denominator by the conjugate of the denominator to eliminate the square root and simplify the expression.

What is the result when calculating the limit of (x^2 + 4x - 5) / (x - 1) as x approaches 1?

First, you factor the numerator to get (x + 5)(x - 1) / (x - 1). After canceling out the common factor (x - 1), the limit becomes x + 5, and substituting x = 1 gives 6.

What do you do if a limit results in a form like 0/0 or ∞/∞?

If a limit results in forms like 0/0 or ∞/∞, you should simplify the expression, often by factoring or rationalizing, and then recompute the limit.

What is the process for finding the limit of a function with a square root in the denominator?

The process involves multiplying both the numerator and denominator by the conjugate of the denominator to rationalize the expression.

Why is factoring important when solving limits that involve expressions like (x^2 + 4x - 5)?

Factoring is important because it allows you to simplify the expression and cancel out common factors, which can resolve indeterminate forms like 0/0.