Matematika SMA - Matriks (2) - Penjumlahan Matriks, Pengurangan Matriks (A)
Summary
TLDRIn this educational video, the instructor dives into matrix operations, focusing on addition and subtraction. Key concepts such as matrix order, commutative and associative properties are explained clearly. Through various examples, the video demonstrates how matrix operations can only be performed when matrices have the same dimensions. The instructor also guides viewers through solving matrix equations involving variables. By breaking down complex topics into simple steps, the video makes learning matrix operations accessible and engaging for students at all levels.
Takeaways
- 😀 Matrix addition and subtraction are possible when matrices have the same dimensions.
- 😀 Matrix addition follows the commutative property: A + B = B + A.
- 😀 Matrix addition is associative: (A + B) + C = A + (B + C).
- 😀 Matrix subtraction does not follow the commutative or associative properties.
- 😀 Before performing operations on matrices, always check if the matrices have the same order.
- 😀 In matrix addition, you sum the corresponding elements from both matrices.
- 😀 In matrix subtraction, you subtract corresponding elements from one matrix to another.
- 😀 If matrices have different dimensions, addition or subtraction cannot be performed.
- 😀 For solving matrix equations, compare corresponding matrix elements to find unknown values.
- 😀 Use substitution or elimination methods to solve for variables in matrix equations with unknowns.
Q & A
What is the main topic discussed in the video?
-The main topic of the video is about matrices, specifically focusing on matrix addition and subtraction.
What are the conditions for performing matrix addition or subtraction?
-Matrix addition or subtraction can only be performed if the matrices involved have the same order (dimensions), and the corresponding elements of the matrices must align or be positioned similarly.
What is the commutative property in matrix addition?
-The commutative property of matrix addition states that A + B equals B + A, meaning the order in which matrices are added does not change the result.
What is the associative property in matrix addition?
-The associative property in matrix addition means that the grouping of matrices does not affect the result. For example, (A + B) + C equals A + (B + C).
Do the commutative and associative properties apply to matrix subtraction?
-No, the commutative and associative properties do not apply to matrix subtraction. The order of subtraction matters and affects the result.
Can you perform matrix addition between matrices A and B with different dimensions?
-No, matrix addition can only be performed if both matrices have the same dimensions (i.e., the same number of rows and columns).
How do you perform matrix addition with the given matrices A and B?
-To add matrices A and B, you add their corresponding elements. For example, if matrix A has elements 6, -2, 3, and 11, and matrix B has elements -4, 12, 5, and 1, you add 6 + (-4), -2 + 12, and so on.
What is the result of matrix A + matrix B with given values?
-Given matrix A as [6, -2; 3, 11] and matrix B as [-4, 12; 5, 1], the result of A + B is [2, 10; 8, 12].
How do you solve for variables x and y in matrix equations?
-To solve for variables x and y, equate corresponding elements of the matrices and solve the resulting system of equations. For example, solving 3x - 5 = 7 will give x = 4.
What method is used to solve the system of equations involving x and y?
-The video suggests using substitution or elimination methods to solve for x and y. For instance, after simplifying equations, you can substitute the value of one variable into the other equation.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Matriks Matematika Wajib Kelas 11 Bagian 2 - Operasi Matriks

MATEMATIKA DASAR ke - 1 untuk SD, SMP & SMA

x64 Assembly Tutorial 19: FLAGS Register, Conditional Jumps and Moves
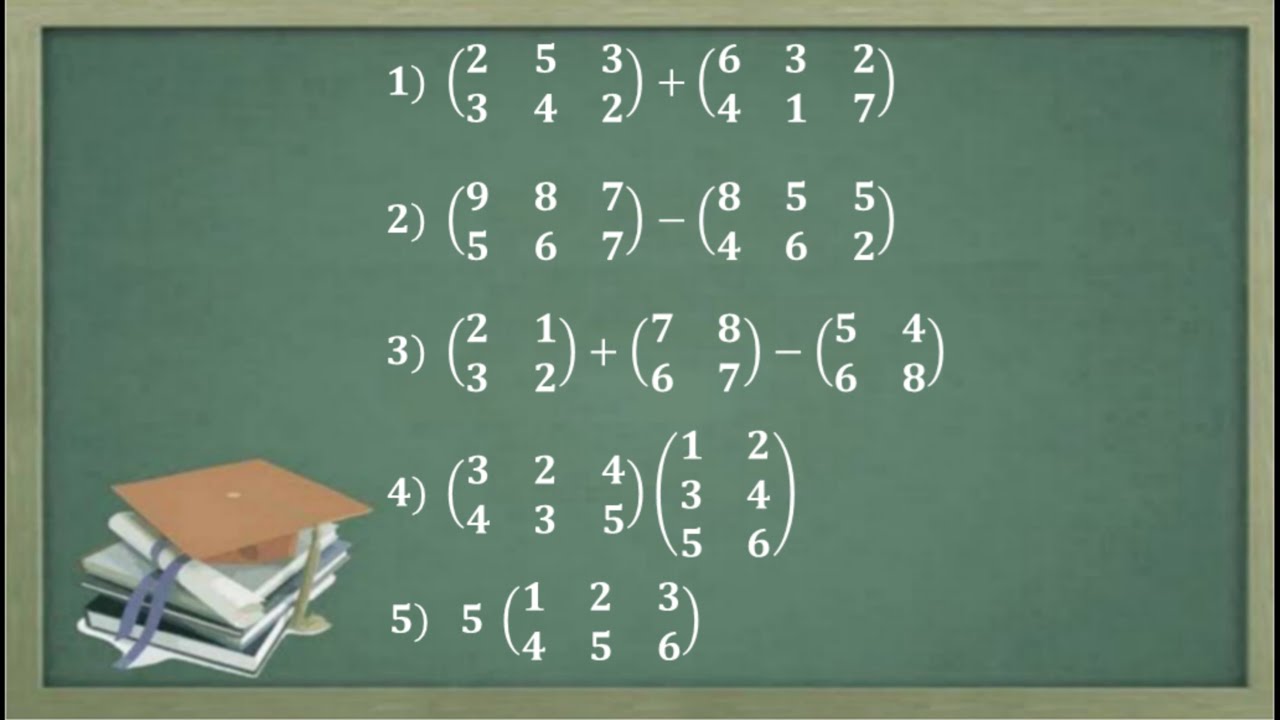
Operasi dasar matriks - Penjumlahan, Pengurangan dan Perkalian Matriks
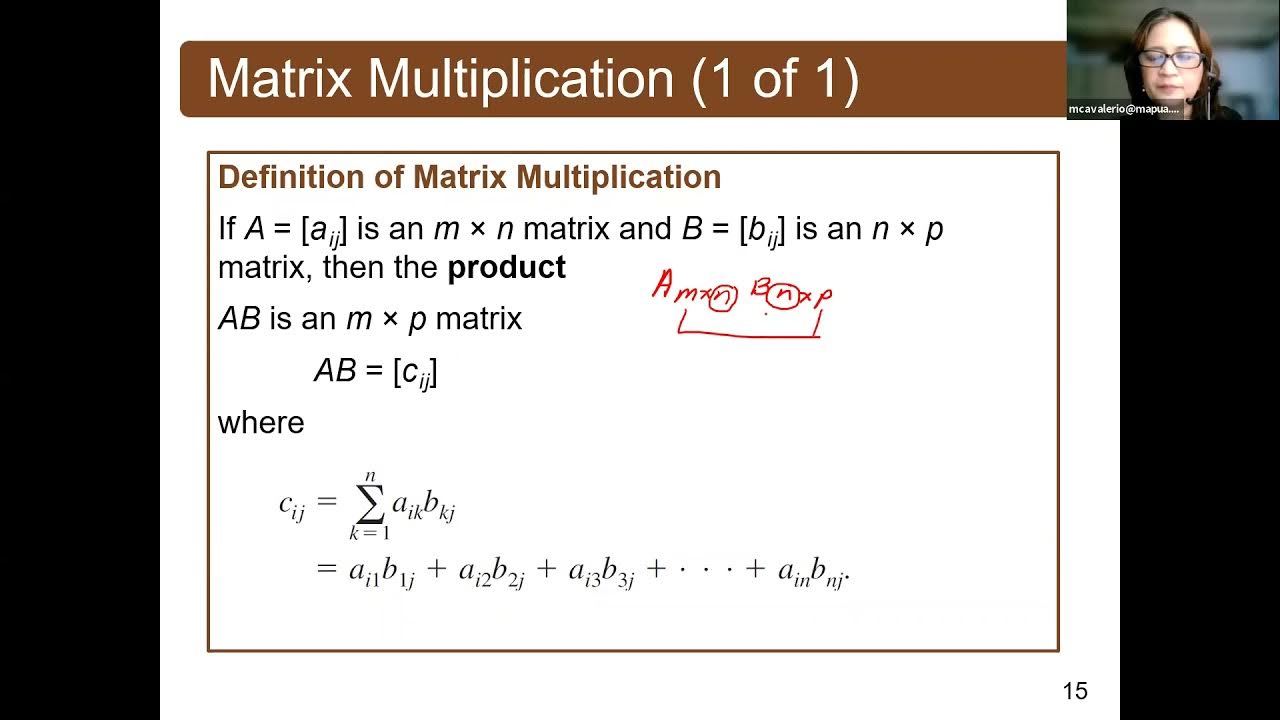
Matrices and Matrix Operations shorter

🔴 HABILIDADE 1. SOMA e SUBTRAÇÃO entre inteiros
5.0 / 5 (0 votes)