Kalkulus : Jumlah Riemann dan Limitnya
Summary
TLDRThis video introduces the concept of definite integrals, focusing on partitioning an interval and calculating Riemann sums. It explains how a partition divides an interval into smaller subintervals, with each subinterval having a corresponding rectangle whose area is part of the Riemann sum. As the partition becomes finer, the sum converges to the exact area under the curve, leading to the definition of the definite integral. The video also distinguishes between integrable and non-integrable functions, using examples to demonstrate the concept. The lecture sets the foundation for further exploration of integration in future lessons.
Takeaways
- 😀 The script introduces the topic of integral calculus, specifically focusing on Riemann sums and the concept of partitions on a closed interval [a, b].
- 😀 A partition of the interval [a, b] is a set of points that divides the interval into smaller subintervals.
- 😀 The points in the partition are denoted as x0, x1, ..., xn, where the number of points (n) depends on the desired level of precision.
- 😀 Each subinterval created by the partition has its own length, denoted as Δxi, which may vary in size.
- 😀 The script explains the concept of the longest subinterval (denoted as non-p) and gives an example where the longest subinterval is ΔX2.
- 😀 A Riemann sum is defined as the sum of areas of rectangles formed by taking sample points in each subinterval and multiplying them by the subinterval lengths.
- 😀 The choice of sample points within each subinterval can vary, and it’s possible to select points at the ends or somewhere within the subintervals.
- 😀 The Riemann sum can be calculated using the formula: Σ f(x_i) * Δx_i, where x_i is the sample point and Δx_i is the length of the corresponding subinterval.
- 😀 As the length of the subintervals becomes smaller (approaching zero), the Riemann sum approaches the exact area under the curve, represented as the integral.
- 😀 If the limit of the Riemann sum exists and is the same regardless of how the partition and sample points are chosen, the function is said to be integrable over the interval [a, b].
Q & A
What is a partition in the context of definite integrals?
-A partition of the interval [a, b] is a set of points that divides the interval into smaller subintervals. These points are denoted as x0, x1, x2, ..., xn, where each xi represents a division point in the interval.
How does a partition affect the subintervals in a definite integral?
-The partition divides the interval [a, b] into several smaller subintervals, each of which may have different lengths. These subintervals are crucial for defining the Riemann sum approximation of the integral.
What is the notation for the longest subinterval in a partition?
-The notation for the longest subinterval in a partition is typically represented as 'delta X_max', which signifies the largest length among the subintervals in the partition.
What is a Riemann sum?
-A Riemann sum is a method for approximating the integral of a function over a closed interval [a, b]. It is calculated by summing the areas of rectangles formed by the function values at certain points within the subintervals of a partition.
How do you calculate a Riemann sum for a given partition?
-To calculate a Riemann sum, for each subinterval, you select a sample point, compute the function value at that point, and multiply it by the width of the subinterval. The sum of these products across all subintervals gives the Riemann sum.
What happens to the Riemann sum as the partition becomes finer (with more subintervals)?
-As the partition becomes finer, meaning the lengths of the subintervals approach zero, the Riemann sum approximates the exact value of the integral more accurately. The limit of the Riemann sum as the partition gets infinitely small is the definite integral.
What is the significance of the limit of the Riemann sum in defining the integral?
-The limit of the Riemann sum as the partition becomes infinitely fine represents the definite integral of the function over the interval [a, b]. This limit exists if the function is integrable on that interval.
What does it mean for a function to be integrable on an interval?
-A function is integrable on an interval if the limit of the Riemann sums exists and does not depend on how the partition is chosen, as long as the partition gets arbitrarily fine.
Can every function be integrated using Riemann sums?
-No, not every function is integrable using Riemann sums. For instance, functions that have discontinuities or undefined behavior over the interval may not have a well-defined Riemann sum or definite integral.
What happens when a function is not integrable, as shown in the example with f(x) = 1/x^2 for x ≠ 0?
-When a function is not integrable, the limit of the Riemann sum does not exist, and the integral does not yield a finite value. For the example with f(x) = 1/x^2, the Riemann sum becomes unbounded as the partition is refined, leading to an infinite result, indicating that the function is not integrable over that interval.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
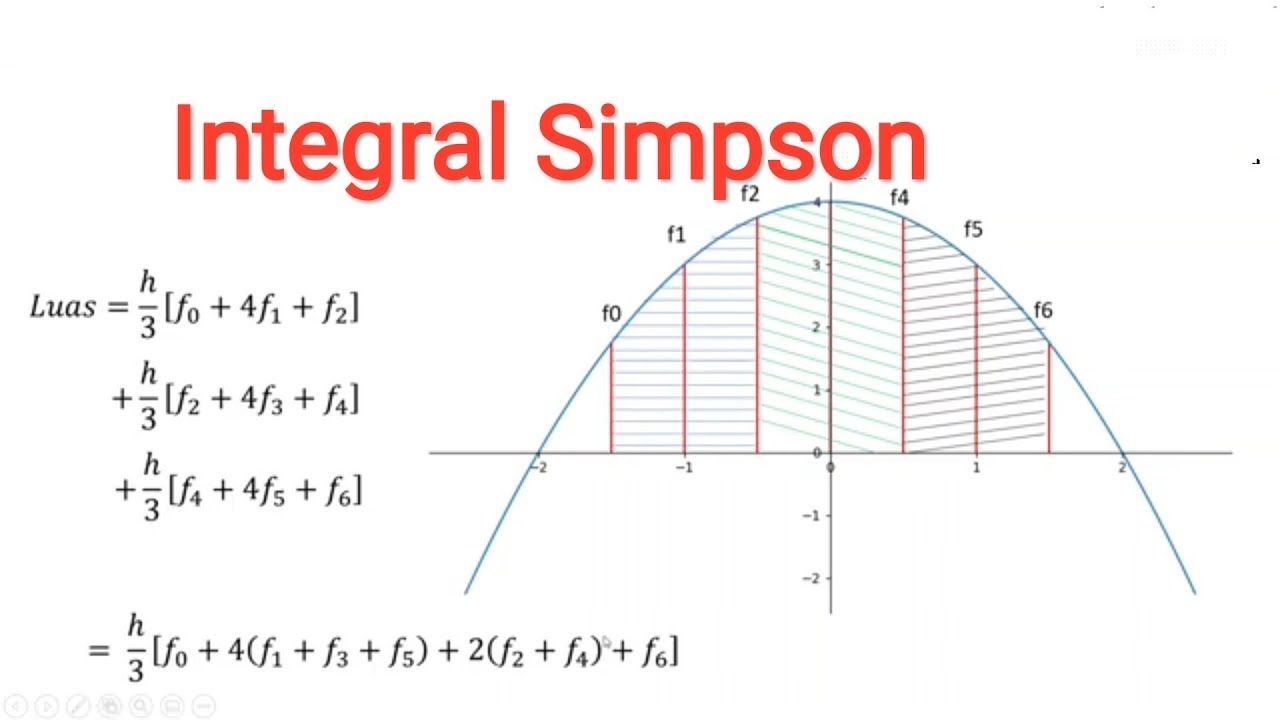
Integral Numerik dengan metode Simpson serta kode Pythonnya.
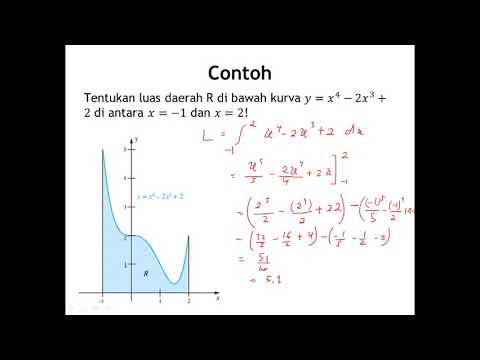
5 1 Luas Daerah Bidang Rata

Integral Tentu - Apa hubungannya dengan notasi sigma? | Integral Tentu (Part 1) | Kalkulus

#06 Konsep Dasar Integral dalam Matematika untuk Fisika Bagian #1

Matematika Dasar II | Integral Lipat Dua pada Persegi Panjang

Integral (Tak Tentu)
5.0 / 5 (0 votes)