Solving Fluid Flow with Bernoulli’s Equation

Summary

In this video, the hosts walk through a fluid mechanics problem involving ideal fluid flow through a pipe. The problem involves calculating the radius of the pipe at point 2 given several known variables, such as fluid density, speed, and pressure differences between two points. Using Bernoulli’s equation and the principle of constant volumetric flow rate, they demonstrate how to solve for the unknown radius. Along the way, the hosts emphasize the importance of careful calculations and correcting errors, resulting in a step-by-step guide to solving this type of physics problem. The explanation is both detailed and engaging, aimed at helping viewers grasp the concept.

Takeaways

• 😀 Bernoulli's equation can be used to solve fluid flow problems involving pressure, speed, and height differences.
• 😀 The problem involves fluid flowing through a pipe with a circular cross-section, and the goal is to find the radius at point 2.
• 😀 The known values in the problem include fluid density, radius at point 1, heights at points 1 and 2, speed at point 1, and pressure difference.
• 😀 The pressure difference between point 1 and point 2 is given as 10.5 kPa, which needs to be converted to pascals (10,500 Pa).
• 😀 Bernoulli's equation relates pressure, speed, and height at two points along the flow, and is key to solving for unknown values like speed and radius.
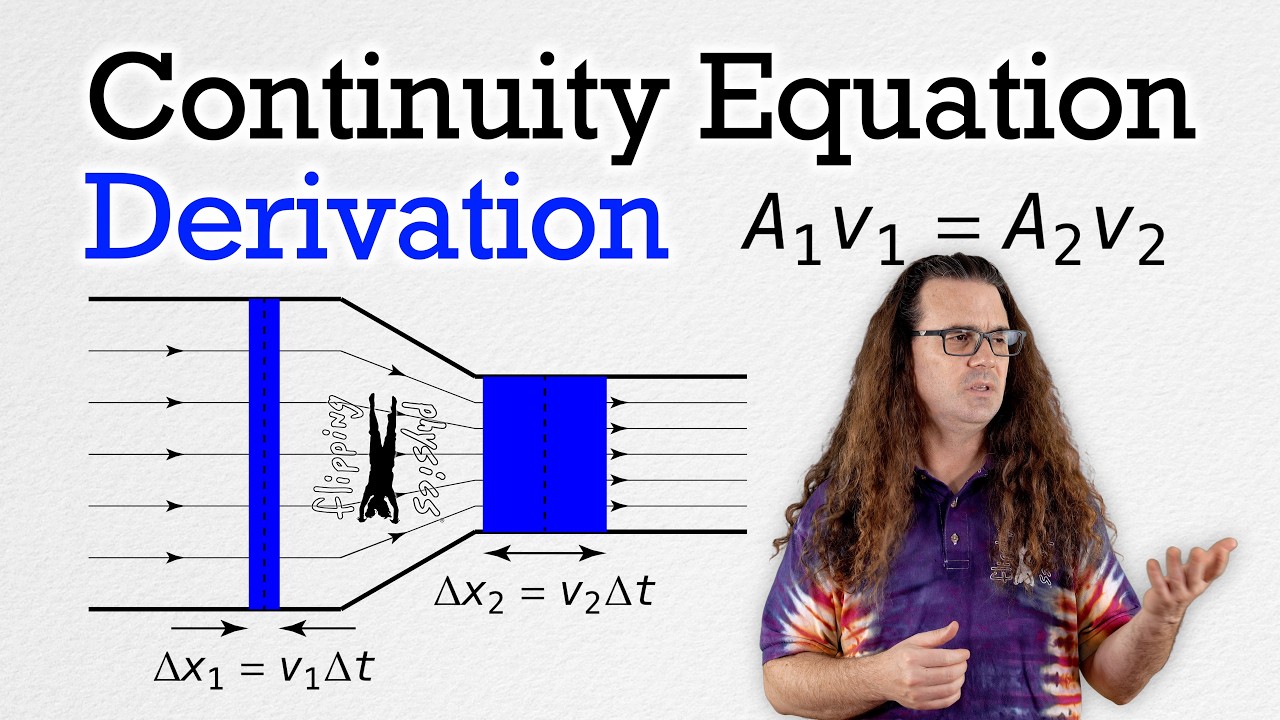
• 😀 The volumetric flow rate is constant in this scenario, meaning that the cross-sectional area times speed is constant between points 1 and 2.
• 😀 To solve for speed at point 2, the equation must be rearranged to account for the change in pressure and height differences.
• 😀 A sign error initially led to an incorrect calculation for speed at point 2, but correcting it gives a final speed of 1.55 m/s at point 2.
• 😀 After finding speed at point 2, the radius at point 2 can be calculated using the relationship between cross-sectional area and speed.
• 😀 The final radius at point 2 is determined to be 0.34 meters by solving the equation for volumetric flow rate and substituting known values.

Q & A

What is the basic principle used to solve this fluid flow problem?

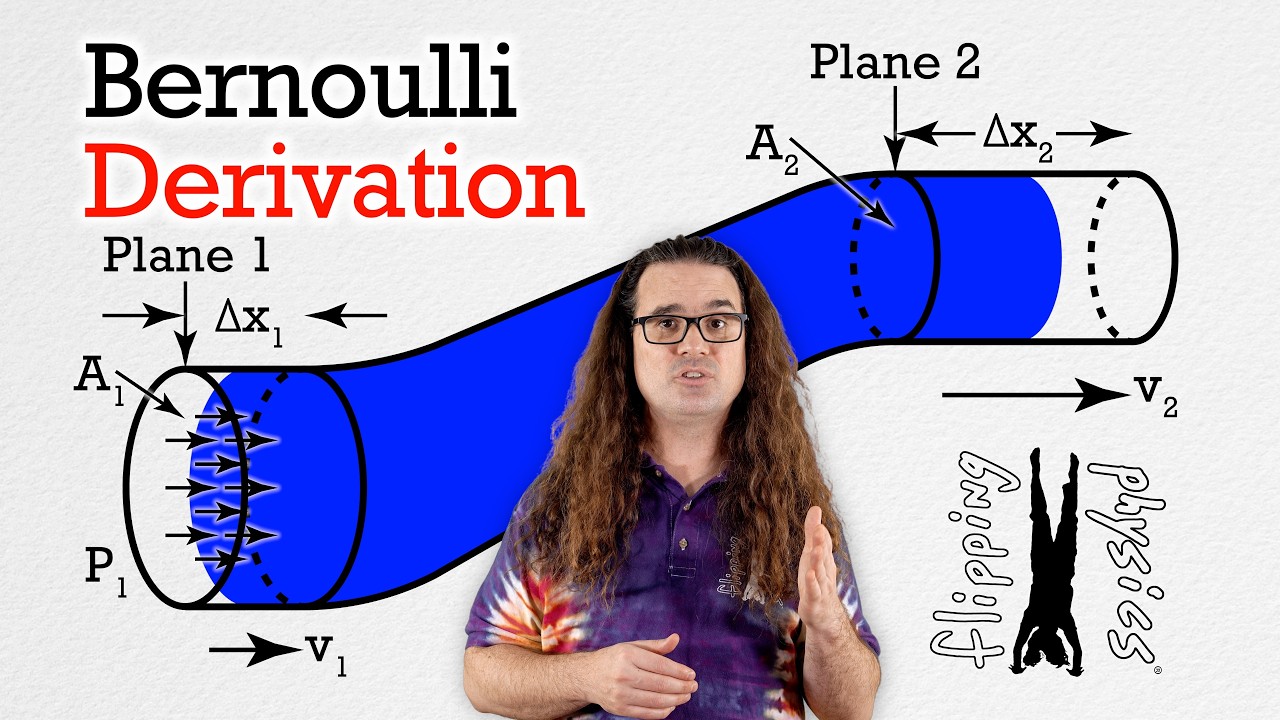
The problem is solved using Bernoulli's equation, which relates pressure, speed, and height in an ideal fluid flow. Additionally, the continuity equation is used, which states that the volumetric flow rate remains constant throughout the pipe.

What are the known values provided in the problem?

The known values include the fluid density (975 kg/m³), radius at point 1 (0.50 meters), height at point 1 (1.5 meters), height at point 2 (2.5 meters), speed at point 1 (0.70 m/s), and the pressure difference between points 1 and 2 (10.5 kilopascals).

How is the pressure difference between points 1 and 2 handled in the calculations?

The pressure difference is given as 10.5 kilopascals lower at point 2 than at point 1. To convert this into the correct units for calculations, it is multiplied by 1000 to convert to pascals (10,500 pascals).

What was the error in the initial steps of solving the problem?

The error occurred due to incorrectly treating the pressure difference as positive. The pressure at point 2 is lower than at point 1, so the pressure difference should be negative (-10.5 kilopascals), which was a mistake that needed to be corrected.

How does Bernoulli's equation apply to this problem?

Bernoulli’s equation is used to relate the pressures, speeds, and heights at both points 1 and 2 in the fluid flow. The equation helps determine the relationship between the pressure difference and the speeds at the two points, allowing for the calculation of the unknown variables.

What role does the continuity equation play in this problem?

The continuity equation is used to relate the speed and cross-sectional area of the pipe at points 1 and 2. Since the volumetric flow rate must remain constant, the equation allows for the calculation of the radius at point 2 after determining the speed at that point.

Why can't we take the square root of a negative number in this context?

Taking the square root of a negative number is not possible in real-number arithmetic, which led to the error in the calculation. This indicates a mistake in the input values, particularly in the sign of the pressure difference, which was later corrected.

What is the significance of the speed at point 2 in this problem?

The speed at point 2 is a key value that can be derived after applying Bernoulli's equation. Once this speed is found (1.5519 m/s), it is used in the continuity equation to determine the radius at point 2.

How is the radius at point 2 calculated?

The radius at point 2 is calculated by applying the continuity equation, which relates the cross-sectional area at point 1 and point 2, using the speeds at both points. The formula involves solving for the radius at point 2 using the speed values and the known radius at point 1.

What is the final answer for the radius at point 2?

After applying the continuity equation and solving for the radius, the final answer for the radius at point 2 is 0.34 meters.