PERNAH NAIK ROLLERCOASTER ? PASTI PERNAH RASAIN GAYA SENTRIPETAL
Summary
TLDRThe video explains the dynamics of centripetal force in circular motion, particularly focusing on objects moving vertically. It covers the relationship between mass, acceleration, and forces at different positions of an object tied to a string, moving in a circular path. The transcript discusses various positions, such as the bottom, top, and intermediate points, and their corresponding forces like tension and gravitational force. Key equations for centripetal force are explained, along with how tension varies at different positions, emphasizing that the greatest tension occurs at the lowest point of the motion.
Takeaways
- 😀 The force acting on a rotating object is centripetal force, which always points toward the center of the circle.
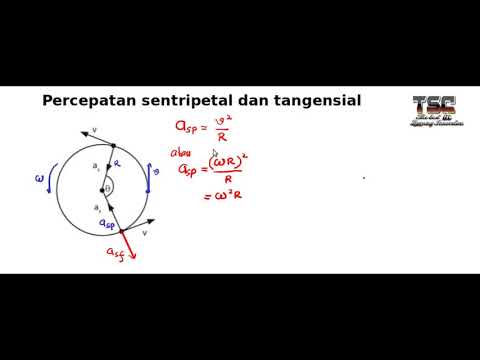
- 😀 The formula for centripetal force is F = m * v² / r, where 'm' is mass, 'v' is velocity, and 'r' is the radius of the circular path.
- 😀 Centripetal acceleration can also be expressed as a = v² / r or a = ω² * r, where 'ω' is angular velocity.
- 😀 Angular velocity (ω) is related to velocity (v) by the equation ω = 2π * f or ω = 2π / t, where 'f' is frequency and 't' is time.
- 😀 The script discusses a vertical ball on a string as a simple example to demonstrate varying forces at different positions during circular motion.
- 😀 At position 1 (the lowest point), the force is the sum of tension in the string and weight acting downward, with centripetal force pointing toward the center.
- 😀 At position 2, the tension force (T) is directed toward the center of the circle, while the weight (W) has a component acting downward and outward.
- 😀 At position 3, the weight is perpendicular to the direction of tension, so only the tension contributes to the centripetal force.
- 😀 Position 4 represents the highest point, where the tension force is counteracted by the object's weight in the downward direction, minimizing tension.
- 😀 Position 5 is similar to position 2, where tension and weight both contribute to the forces acting on the object, with the tension being slightly less than at the lowest point.
Q & A
What is centripetal force in circular motion?
-Centripetal force is the force that acts on an object moving in a circular path, always directed toward the center of the circle. It keeps the object in circular motion, preventing it from flying off tangentially.
How is centripetal force related to the velocity and radius of the circular motion?
-The centripetal force (F_s) is given by the formula F_s = mv²/r, where m is the mass of the object, v is the velocity, and r is the radius of the circle. It can also be expressed as F_s = m * ω² * r, where ω is the angular velocity.
What is the role of tension in circular motion?
-Tension in the string or rope acts to provide the centripetal force required for circular motion. The tension varies at different positions of the circular path, especially in vertical circular motion, where it also has to counteract the force of gravity.
How does the tension in the rope vary at different positions in vertical circular motion?
-At the lowest point (position 1), the tension is highest because it has to support both the gravitational force and provide the centripetal force. At the highest point (position 4), the tension is lowest as only the centripetal force needs to be provided.
Why is the tension in the rope highest at the lowest point of the circular motion?
-At the lowest point, the rope must counteract both the gravitational force pulling the object downward and supply the necessary centripetal force to keep the object in circular motion. Therefore, the total force (tension) is highest at this point.
What happens to the tension at the highest point of the circular motion?
-At the highest point, the tension in the rope is at its lowest because the gravitational force assists in providing the centripetal force. The rope only needs to supply enough force to maintain circular motion.
What is the significance of the angle (α or β) in determining the forces in circular motion?
-The angles α and β represent the positions of the object during its motion. These angles are used to resolve forces into components, especially in the case of forces like gravity that are acting at an angle. The components help calculate the net force acting toward the center of the circle.
What happens to the forces acting on the object at position 3 in vertical circular motion?
-At position 3, the gravitational force (W) and the tension in the rope (T) are both perpendicular to the direction of motion. The tension is the only force contributing to the centripetal force, while gravity does not directly affect the centripetal force at this position.
Why does the object experience the largest tension at the bottom of the circular path?
-At the bottom of the circular path, the object is subject to both gravitational force pulling it downward and the centripetal force pulling it toward the center of the circle. The combined effect results in the largest tension in the rope.
How does the direction of forces change as the object moves along the circular path?
-As the object moves along the circular path, the direction of the centripetal force remains toward the center of the circle. However, the direction of the gravitational force remains constant (downward), and the direction of the tension force varies based on the position in the circle.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade Now5.0 / 5 (0 votes)