OPERAÇÕES COM FRAÇÕES | - VOCÊ SABE? \Prof.Gis
Summary
TLDRThis video offers a comprehensive guide to mastering fraction operations, covering addition, subtraction, multiplication, division, exponentiation, and square roots. Using practical methods like the butterfly technique, ping-pong method, and clear explanations, the instructor helps students overcome common difficulties with fractions. The video emphasizes understanding and practicing core concepts, simplifying fractions, and using visual aids. It’s an engaging, step-by-step approach that helps learners gain confidence in working with fractions and prepares them for activities and tests.
Takeaways
- 😀 The butterfly technique is a helpful method for adding and subtracting fractions with different denominators.
- 😀 When adding or subtracting fractions, multiply the denominators, then add or subtract the results of the numerators accordingly.
- 😀 Simplifying fractions involves finding the greatest common divisor (GCD). If no GCD exists, the fraction is irreducible.
- 😀 Multiplying fractions is straightforward: multiply the numerators and the denominators together.
- 😀 After multiplying fractions, check if the result can be simplified by finding a common divisor for the numerator and denominator.
- 😀 Division of fractions can be simplified using the 'ping-pong' technique: multiply the numerator of the first fraction by the denominator of the second, and vice versa.
- 😀 Potentiation involves multiplying equal factors. In the case of fractions, this means multiplying the fraction by itself.
- 😀 When squaring a fraction, both the numerator and the denominator are squared separately.
- 😀 The square root of a fraction can be simplified by taking the square roots of the numerator and the denominator independently.
- 😀 Potentiation and rooting are inverse operations—squaring a fraction and then taking its square root will return the original fraction.
Q & A
What is the butterfly technique used for?
-The butterfly technique is used to add or subtract fractions with different denominators. It involves multiplying the numerator of one fraction by the denominator of the other (forming 'wings'), then adding or subtracting these results. The denominator is found by multiplying the two denominators.
Can you explain the steps for adding two fractions using the butterfly technique?
-To add two fractions using the butterfly technique, first multiply the numerator of the first fraction by the denominator of the second. Then do the same for the other pair. Add the results together for the numerator and multiply the denominators to find the new denominator.
How do you handle fractions that cannot be simplified?
-A fraction that cannot be simplified is called an 'irreducible fraction.' This occurs when the numerator and denominator do not share any common divisors. For example, 23/20 is irreducible because 23 is a prime number.
What is the ping-pong method for dividing fractions?
-The ping-pong method for dividing fractions involves multiplying the numerator of the first fraction by the denominator of the second, and vice versa. This method avoids the need for complex steps, such as inverting the second fraction.
When multiplying fractions, how do you simplify the result?
-To simplify the result after multiplying fractions, check if the numerator and denominator share any common factors. If they do, divide both by the greatest common divisor to simplify the fraction.
What does it mean to simplify a fraction?
-Simplifying a fraction means reducing it to its simplest form by dividing both the numerator and the denominator by their greatest common divisor (GCD). If no common divisor exists, the fraction is irreducible.
How is potentiation (exponentiation) with fractions performed?
-To raise a fraction to a power, you multiply the fraction by itself as many times as the exponent indicates. For example, to square 3/4, you multiply it by itself: (3/4) × (3/4) = 9/16.
What is the relationship between square roots and potentiation?
-Square roots and potentiation are inverse operations. If you square a fraction and then take the square root of the result, you should return to the original fraction. For example, the square root of 9/16 is 3/4.
Why is it unnecessary to convert fractions into decimals when performing operations?
-It is unnecessary to convert fractions into decimals when performing operations because fractions can be directly manipulated using arithmetic rules. Converting to decimals is often more complicated and less precise.
Can you explain how to calculate the square root of a fraction?
-To calculate the square root of a fraction, take the square root of the numerator and the denominator separately. For example, the square root of 9/16 is calculated as √9/√16 = 3/4.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
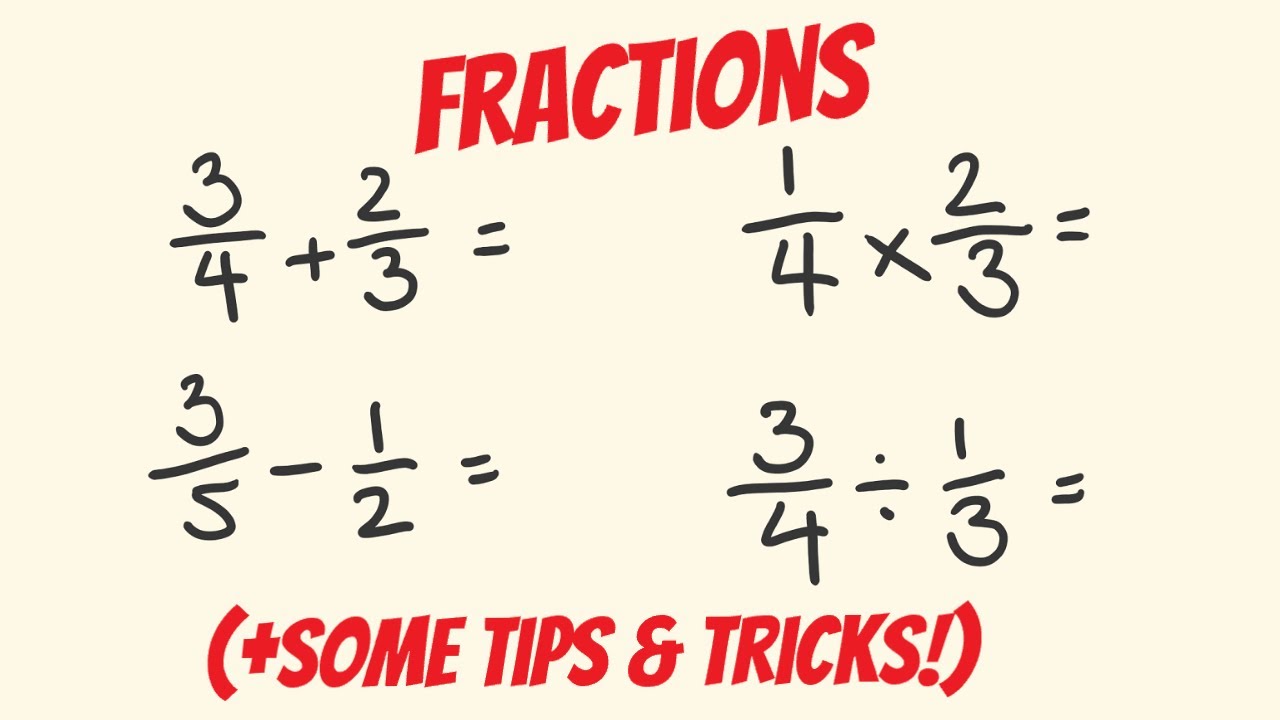
How to Calculate ANY Fraction Easily!

MATEMATIKA DASAR ke -3 untuk SD, SMP, SMA

Tutorial on Using Arithmetic Operators in Microsoft Excel

CARA MUDAH PENJUMLAHAN PENGURANGAN PERKALIAN DAN PEMBAGIAN BENTUK AKAR

Cara Mengenalkan Konsep Perkalian dan Pembagian kepada siswa MI/SD

Tips Mudah Menyederhanakan Bentuk Akar || Bab Bilangan Berpangkat (Part 2)
5.0 / 5 (0 votes)