PROGRAM LINEAR
Summary
TLDRIn this educational video, the concept of linear programming is applied to a culinary business. The story follows Remon, who bakes and sells donuts and cupcakes, and faces two key constraints: limited flour (240 grams) and a display rack that can hold only 10 items. The goal is to maximize revenue by determining how many donuts (Rp10,000 each) and cupcakes (Rp7,000 each) Remon should make. Using a system of linear inequalities, the optimal solution is found: by producing 4 donuts and 6 cupcakes, Remon can achieve the maximum revenue of Rp82,000. The video demonstrates how to approach such problems using linear programming techniques.
Takeaways
- 😀 Remon runs a bakery, making and selling donuts and cupcakes at a local store.
- 😀 Donuts are sold for Rp10,000 each, while cupcakes are sold for Rp7,000 each.
- 😀 There is a limited supply of flour at home, only 240 grams available.
- 😀 To make one donut, 30 grams of flour is required, and to make one cupcake, 20 grams of flour is needed.
- 😀 The bakery display can hold a maximum of 10 cakes at once.
- 😀 The goal is to maximize profit by determining how many donuts (X) and cupcakes (Y) to make, given constraints.
- 😀 The problem is formulated as a system of linear inequalities, forming a linear programming model.
- 😀 The inequalities based on flour availability and display capacity are X ≥ 0, Y ≥ 0, 3X + 2Y ≤ 24, and X + Y ≤ 10.
- 😀 The feasible solution region is determined by the intersection of these inequalities, where X and Y are non-negative.
- 😀 The four potential corner points of the feasible region are (0, 10), (8, 0), (4, 6), and (0, 0).
- 😀 By testing different combinations of donuts and cupcakes, the maximum profit of Rp82,000 is achieved by making 4 donuts and 6 cupcakes.
Q & A
What is the primary goal of Remon's culinary business?
-The primary goal is to maximize profits from selling donuts and cupcakes by optimizing the number of each item produced, considering constraints such as limited flour and display space.
How much does Remon sell each donut and cupcake for?
-Each donut is sold for Rp10,000, and each cupcake is sold for Rp7,000.
What is the flour constraint in the problem?
-Remon has only 240 grams of flour available, with each donut requiring 30 grams and each cupcake requiring 20 grams of flour.
What is the display capacity for the products?
-The display can hold a maximum of 10 items (either donuts or cupcakes or a combination of both).
How are the number of donuts and cupcakes represented in the model?
-The number of donuts is represented by 'X' and the number of cupcakes is represented by 'Y'. Both X and Y must be non-negative integers.
What are the constraints in the linear programming model?
-The constraints include: the flour constraint (3X + 2Y ≤ 240), the display capacity (X + Y ≤ 10), and the non-negativity conditions (X ≥ 0, Y ≥ 0).
What does the solution region represent in the context of this problem?
-The solution region, which is the area where all constraints are satisfied, represents the possible combinations of donuts and cupcakes that Remon can produce and sell.
How is the maximum profit determined in the problem?
-The maximum profit is determined by evaluating the profits at the corner points (vertices) of the feasible region. The combination that yields the highest profit is selected.
What are the key corner points in the feasible region for this problem?
-The key corner points are (0, 100), (8, 0), and (4, 6). These are the points where the profit is maximized.
What is the maximum profit Remon can earn from his bakery business?
-The maximum profit is Rp82,000, which can be achieved by producing 4 donuts and 6 cupcakes.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
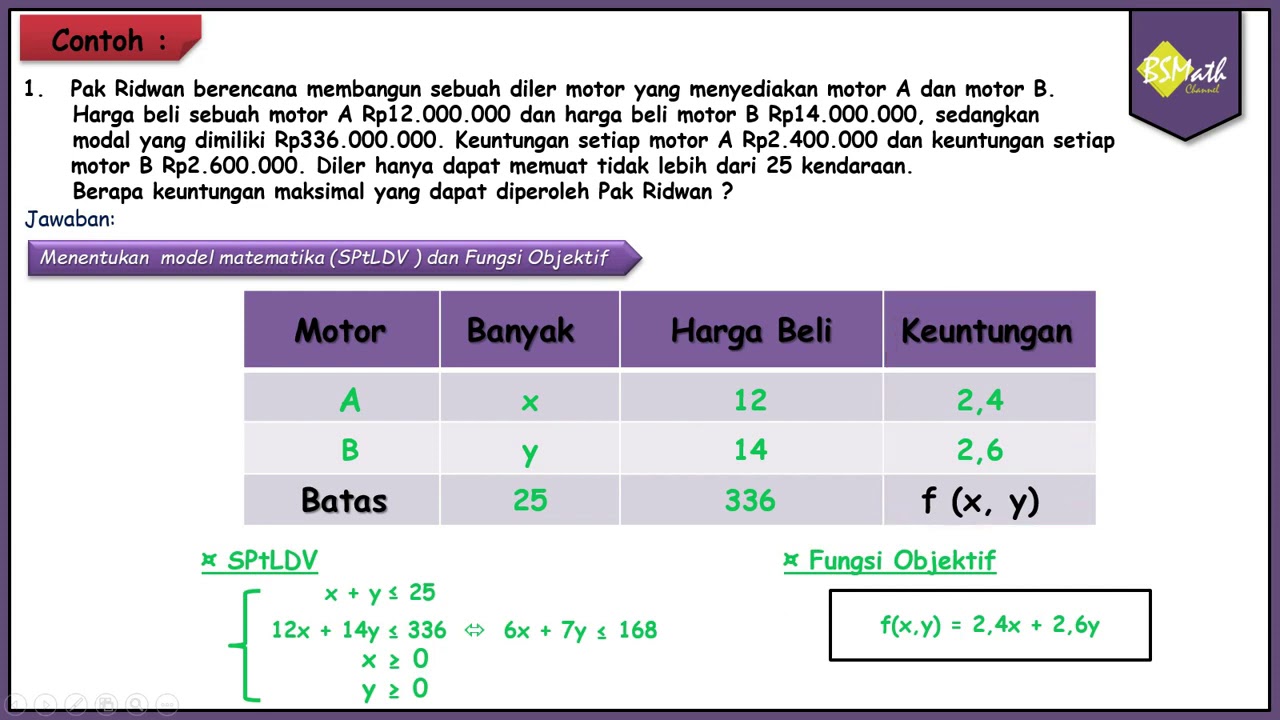
Menyelesaikan Permasalahan Program Linear Menentukan Nilai Optimum dengan Metode Uji Titik Pojok

Linear Programming, GAMPANG BANGET!

1 Linear Programming - Concept17072020
PROGRAM LINIER - METODE GRAFIK - RISET OPERASI

Riset Operasi #1 - Konsep Dasar Riset Operasi | Tutor Manajemen by Gusstiawan Raimanu

Materi Pemuaian Kelas 7 SMP
5.0 / 5 (0 votes)