Domain & Range dari Grafik Fungsi #fazanugas

Summary

This video tutorial provides a clear explanation of how to determine the domain and range of different mathematical functions using their graphical representations. It covers linear, quadratic, and rational functions, focusing on interpreting the graphs' boundaries. The domain is defined by the possible **x** values, and the range by the corresponding **y** values. Examples show how to handle filled and open dots, asymptotes, and the influence of the graph's limits. This tutorial is a helpful guide for understanding the relationship between the graph and its mathematical properties.

Takeaways

• 😀 The domain of a function refers to the set of all possible input values (x-values) that the function can accept based on its graph.
• 😀 The range of a function refers to the set of all possible output values (y-values) that the function can produce, determined by its graph.
• 😀 For a linear function, the domain and range are typically infinite, with limits only specified by any boundaries seen on the graph.
• 😀 The domain can be written using set notation, such as 'x ∈ ℝ' for real numbers, or interval notation, like '(-∞, 4]' for an x-value range up to 4.
• 😀 The range of a function is written similarly, for example, 'y ≥ -2' or 'y ∈ ℝ' for all real y-values, depending on the graph's limits.
• 😀 A filled dot on the graph indicates that the value at that point is included in the domain or range (e.g., x = 4). An open dot means the value is excluded (e.g., x = -3).
• 😀 For quadratic functions (parabolas), the domain is always all real numbers, but the range is limited by the minimum or maximum y-value of the vertex.
• 😀 Rational functions often have asymptotes where the graph approaches but never crosses, resulting in excluded values in the domain (e.g., x ≠ -1).
• 😀 Asymptotes on the graph, whether vertical or horizontal, indicate values the function cannot reach or touch, which affect the domain and range.
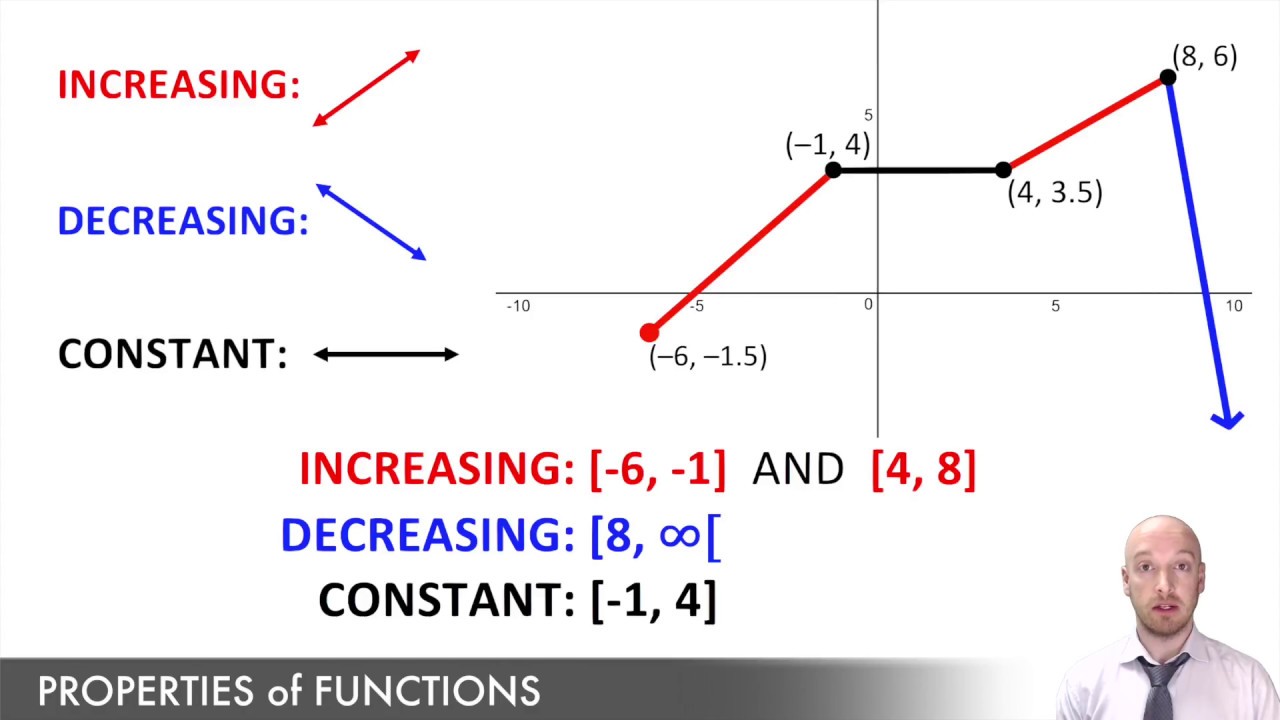
• 😀 The domain and range of piecewise functions are determined by analyzing each piece individually and noting any breaks or discontinuities between them.
• 😀 Understanding how to interpret the graph's points, including filled and open dots, as well as the behavior at the edges of the graph, is crucial for correctly determining the domain and range.

The video is abnormal, and we are working hard to fix it.
Please replace the link and try again.