Differentiability of functions of several variables/L18/existence partial derivatives multivariable
Summary
TLDRIn this tutorial, the instructor explains methods for checking the differentiability of functions with two variables, emphasizing the importance of partial differentiation. The video covers the steps to assess whether a function is differentiable using both standard methods and an alternate approach without calculating partial derivatives. The instructor demonstrates the application of these methods through a series of examples, stressing the need for understanding the continuity of derivatives and conditions under which a function can still be considered differentiable. Viewers are encouraged to review related topics and videos for a comprehensive understanding.
Takeaways
- 😀 The script focuses on understanding the concept of differentiability of functions with two variables, using different methods for checking it.
- 😀 The main topic discussed is partial differentiation, with emphasis on its application in determining the differentiability of functions.
- 😀 It's explained that a function's differentiability is determined by checking if partial derivatives exist and are continuous.
- 😀 There is a method to check differentiability without directly computing partial derivatives, using a specific approach to analyze the behavior of functions.
- 😀 A function is considered differentiable if, at a given point, its partial derivatives exist and satisfy certain continuity conditions.
- 😀 If partial derivatives exist and are continuous, the function is guaranteed to be differentiable at that point.
- 😀 However, even if partial derivatives exist and are not continuous, the function may still be differentiable in some cases.
- 😀 The script outlines that differentiability also depends on whether the function behaves well when tested with limit processes.
- 😀 The process of checking differentiability is illustrated with the example of a function given in the script, using specific steps and conditions to confirm its differentiability.
- 😀 The script stresses the importance of understanding the behavior of functions in limit conditions, where certain rules of continuity and partial differentiation apply to determine differentiability.
Q & A
What is the main topic of the script?
-The main topic of the script is differentiability and partial differentiation of functions with two variables, as well as methods to check for differentiability in multivariable functions.
What is the significance of continuous partial derivatives in the context of differentiability?
-The script emphasizes that for a function to be differentiable, both partial derivatives must either exist or at least one of them must be continuous. If either condition is met, it can indicate differentiability, but continuity of both partial derivatives is crucial for a confirmed differentiability.
Can a function be differentiable even if its partial derivatives are not continuous?
-Yes, a function can still be differentiable even if its partial derivatives are not continuous, as long as the partial derivatives exist at the point. The script mentions that it’s possible for the partial derivatives to exit but not be continuous and the function may still be differentiable.
What is the method discussed for checking differentiability without directly calculating partial derivatives?
-The script outlines a method where you check the differentiability of a function by considering if the function behaves like a sum of terms that satisfy certain conditions, including going to zero when certain limits are applied.
How does the script suggest testing a function for differentiability at a specific point?
-The script suggests testing differentiability at a point by checking if the function can be written in a form where both partial derivatives go to zero at the point of interest, while also confirming that the terms behave independently and approach zero under the right conditions.
What condition must be satisfied for a function to be differentiable at a point, according to the script?
-For a function to be differentiable at a point, the script indicates that both the partial derivatives must exist and either both must be continuous, or at least one of them should be continuous at that point.
What does the script explain about the continuity of partial derivatives?
-The script explains that while it is ideal for partial derivatives to be continuous for the function to be differentiable, even if they are not continuous, the function can still be differentiable, as long as the derivatives exist at the point being considered.
What is the role of the method involving limits in checking differentiability?
-The method involving limits plays a crucial role in checking the behavior of the function’s partial derivatives. By calculating limits, the script shows that if the limits approach zero under the right conditions, it confirms that the function is differentiable.
What does the script suggest when both partial derivatives are not continuous?
-The script suggests that even if both partial derivatives are not continuous, the function can still be differentiable, but the specific conditions of their behavior at the point must be carefully analyzed using the limit approach.
How does the script explain the difference between partial derivatives that exist but are not continuous?
-The script clarifies that when partial derivatives exist but are not continuous, the function may still be differentiable, but there is a possibility that the function does not exhibit full continuity in its derivatives, which requires further verification through limit calculations.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Partial Derivatives (Part 1)

Partial Differentiation Example And Solution | Multivariable Calculus

Partial derivatives, introduction
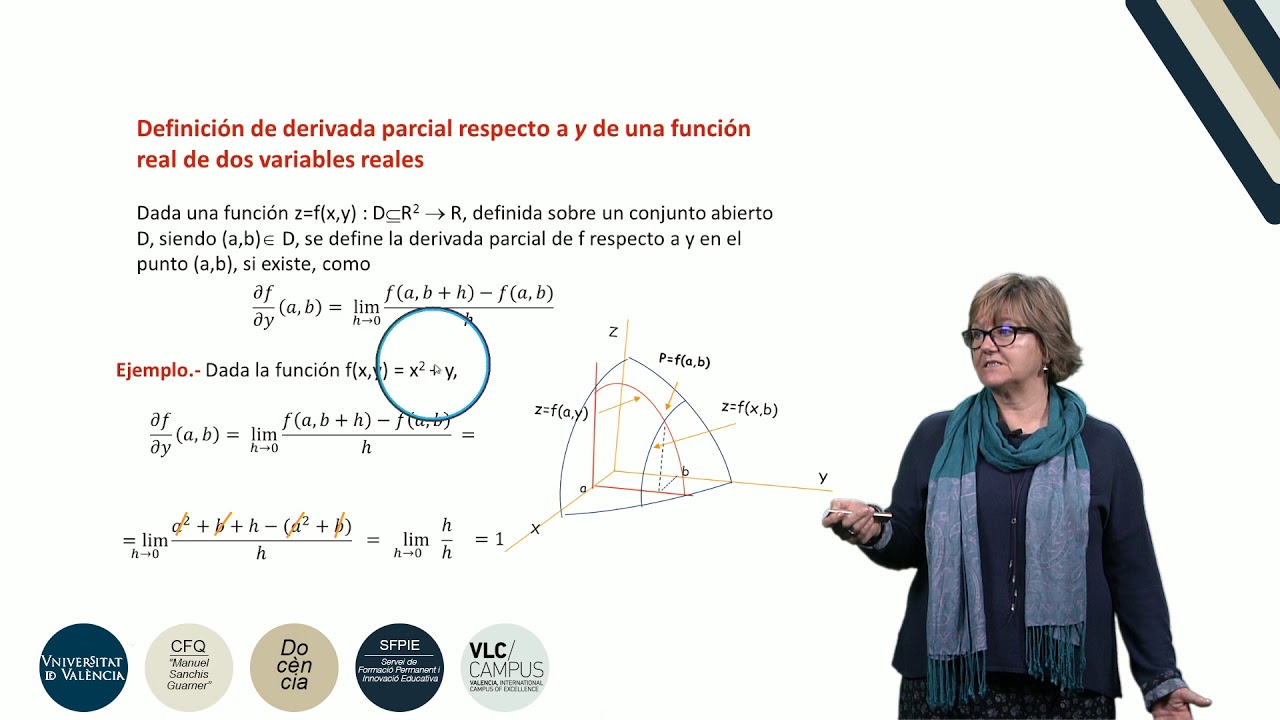
Derivadas parciales. Interpretación geométrica

KALKULUS | TEKNIK PENGINTEGRALAN | METODE INTEGRAL PARSIAL

رياضيات 3 - ثالث ثانوي - درس : تحليل التمثيلات البيانية للدوال والعلاقات
5.0 / 5 (0 votes)