Busur Lingkaran | Matematika SMA Kelas XI Kurikulum Merdeka
Summary
TLDRThis video tutorial explains the concept of an arc in a circle, focusing on its definition, types (minor and major arcs), and how to calculate its length. The presenter begins by introducing the definition of an arc as a curved set of points on a circle, before distinguishing between minor and major arcs based on their length relative to the circle. The tutorial then delves into the formula for calculating the length of an arc, involving the central angle and the radius of the circle. A practical example demonstrates the application of this formula, making the topic accessible and engaging for viewers.
Takeaways
- 😀 Busur (arc) in mathematics refers to a curved segment of a circle’s circumference.
- 😀 A busur can either be a minor arc (smaller part) or a major arc (larger part) of the circle.
- 😀 A minor arc has a length less than half of the circle’s circumference, while a major arc is more than half.
- 😀 The symbol for an arc is represented as a curve above the points, such as 'AB' for the arc between points A and B.
- 😀 When no specific mention of a minor or major arc is made, it is assumed to refer to the minor arc.
- 😀 To calculate the length of a busur, the formula used is: length = (α / 360°) * 2πr, where α is the central angle and r is the radius.
- 😀 The central angle α is the angle formed at the center of the circle by lines drawn from the center to the points on the circle.
- 😀 The total angle in a circle is 360°, and the central angle represents a portion of that full angle.
- 😀 The formula to calculate the arc length is derived from the relationship between the central angle, the circumference of the circle, and the radius.
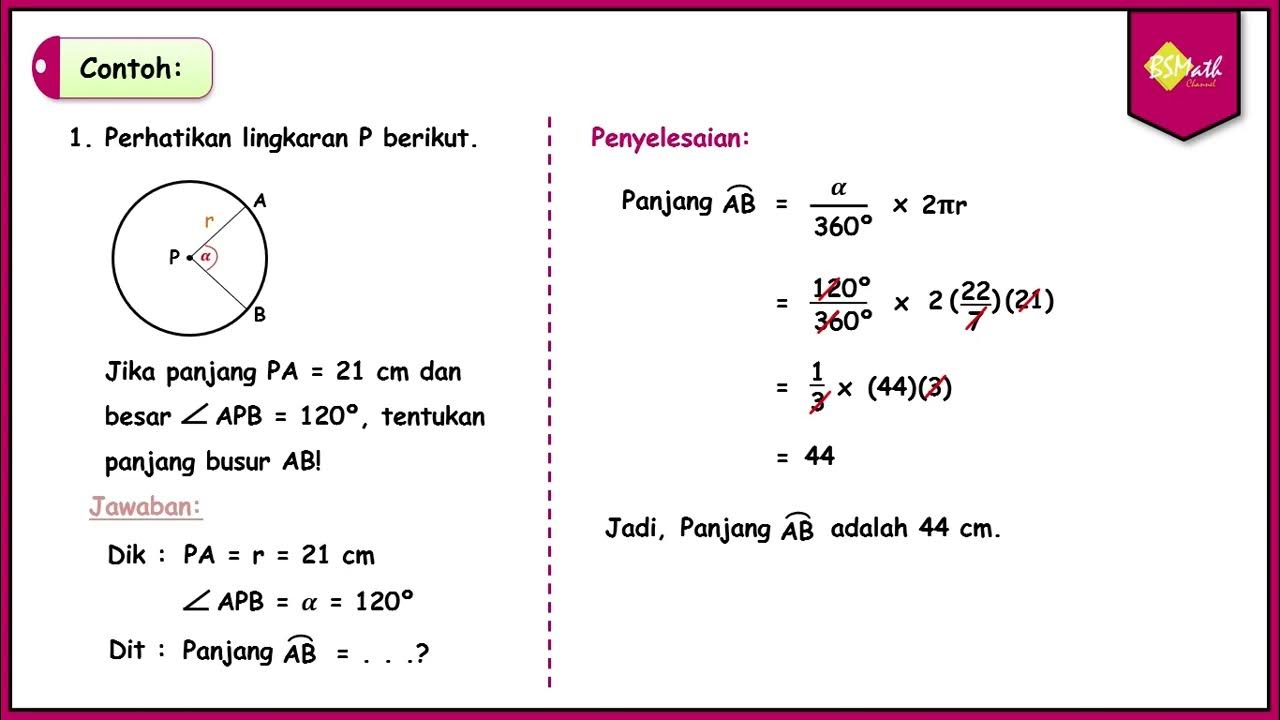
- 😀 In the example problem, the arc length is calculated using the given radius (21 cm) and central angle (120°), yielding a busur length of 44 cm.
Q & A
What is the definition of a busur (arc) in mathematics?
-In mathematics, a busur (arc) is defined as a curve formed by a set of points along the circumference of a circle. It can be either a minor arc, which is less than half the circumference, or a major arc, which is greater than half the circumference.
What is the difference between a minor arc and a major arc?
-A minor arc is the smaller arc between two points on the circumference of a circle, and its length is less than half the circumference. A major arc, on the other hand, is the larger arc between the same two points, and its length is greater than half the circumference.
How is an arc symbolized in mathematics?
-An arc is symbolized by the two endpoints of the arc with a curved line above them. For example, the arc between points A and B on a circle is represented as AB with a curved line above the letters.
What elements are needed to calculate the length of an arc?
-To calculate the length of an arc, you need to know the radius of the circle (r) and the central angle (alpha) formed by the two endpoints of the arc.
What is the formula for calculating the length of an arc?
-The formula for calculating the length of an arc is: Length of arc = (α / 360°) * 2πr, where α is the central angle in degrees, r is the radius of the circle, and π is approximately 3.14 or 22/7.
What is the central angle in a circle?
-The central angle in a circle is the angle formed at the center of the circle by two radii connecting the center to two points on the circumference. The central angle determines the size of the arc it subtends.
In the example problem, what are the known values used to calculate the length of the arc?
-In the example problem, the known values are: the radius of the circle (r = 21 cm) and the central angle (α = 120°). These values are used to calculate the length of the arc.
How can we simplify the fraction when calculating the length of an arc?
-In the example, the fraction representing the central angle (120°/360°) is simplified to 1/3, as 120 is a third of 360. This simplification helps make the calculation easier.
Why do we use 22/7 as an approximation for π in this calculation?
-In this calculation, 22/7 is used as an approximation for π because the radius (21 cm) is a multiple of 7, making the calculation easier and avoiding the need to use a decimal approximation for π.
What is the final result when calculating the length of the arc in the example?
-The final result for the length of the arc, after applying the formula and simplifying the calculation, is 44 cm.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
Lingkaran dan Busur Lingkaran - Matematika SMA Kelas XI Kurikulum Merdeka
THEOREMS INVOLVING CHORDS, ARCS, CENTRAL ANGLES || GRADE 10 MATHEMATICS Q2
Circles and Angles (Outside, Inside, On)

Comprimento de um Arco

Cara Menghitung Panjang Busur dan Luas Juring Lingkaran
Pre-Calculus 12 - Video #25: Trigonometric Basics
5.0 / 5 (0 votes)