Power Series

Summary

In this lecture, Professor Dave introduces the concept of power series, explaining their general form and how they represent infinite sums. He covers essential topics like convergence, divergence, and the use of the ratio test to assess whether a series converges. The lecture also delves into the concept of the radius and interval of convergence, offering a clear explanation with examples. Ultimately, the lesson provides a solid foundation for understanding the conditions under which a power series converges and how to calculate its interval of convergence, making it a crucial topic for calculus students.

Takeaways

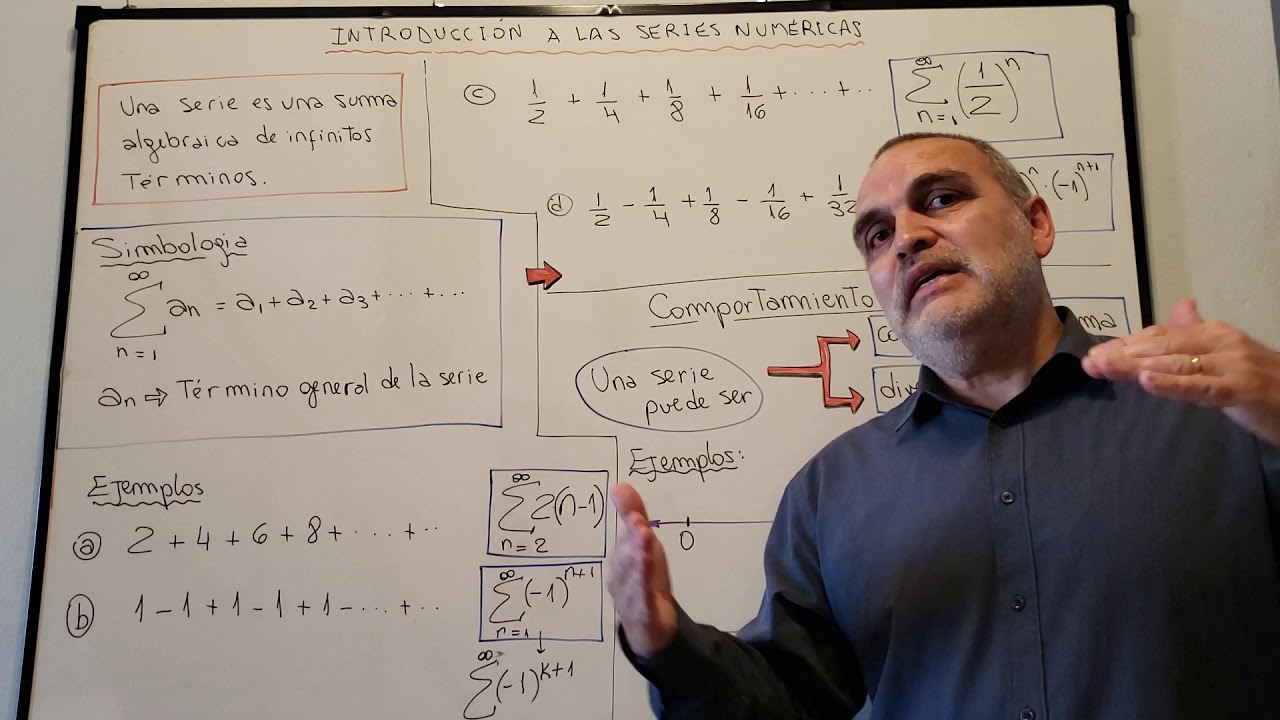
• 😀 A power series is a sum of terms in the form Cₙ * Xⁿ, where Cₙ are coefficients and X is raised to increasing powers of n.
• 😀 The sum of a power series can be represented by a function F(X), which often resembles a polynomial with infinitely many terms.
• 😀 The domain of a power series depends on the values of X for which the series converges.
• 😀 A power series can be convergent or divergent, depending on the X values within its domain.
• 😀 When all coefficients in a power series are equal to one, the series becomes a geometric series, which converges when |X| < 1.
• 😀 In some power series, terms involve (X - A) raised to the N-th power, and convergence can be assessed using the ratio test.
• 😀 The ratio test for convergence involves comparing the ratio of consecutive terms and determining whether the limit as N approaches infinity is less than 1.
• 😀 The convergence criteria from the ratio test for the series (X - 3)ⁿ/N shows that the series converges when 2 < X < 4, or |X - 3| < 1.
• 😀 A general theorem states that power series can have three convergence behaviors: only at X = A, for all X, or for values of X within a certain radius R.
• 😀 The radius of convergence (R) defines the interval where the series converges. If |X - A| < R, the series converges, and if it's greater than R, the series diverges.
• 😀 The radius of convergence can be 0 (for convergence only at a single point), infinite (for convergence for all X), or a specific value depending on the series, as demonstrated by examples like Xⁿ / N!.

Q & A

What is the general form of a power series?

A power series takes the form: ∑(C_n * X^n) from n=0 to infinity, where C_n are constants (coefficients), and X is a variable raised to increasing powers.

How is the domain of a power series determined?

The domain of a power series is the set of all X values for which the series converges.

What happens when all the coefficients in a power series are equal to one?

When all the coefficients are equal to one, the series becomes a geometric series, which converges for X values between -1 and 1.

How can we determine if a power series converges or diverges?

The convergence or divergence of a power series can often be determined using the ratio test, which compares the terms of the series and assesses their behavior as n approaches infinity.

What does the ratio test reveal about the convergence of a series?

The ratio test helps determine the values of X for which the series converges by examining the limit of the ratio of successive terms in the series.

What is the radius of convergence for a power series?

The radius of convergence, denoted by R, is the distance from the center (A) within which the power series converges. If |X - A| < R, the series converges, and if |X - A| > R, it diverges.

What does it mean if the radius of convergence is infinite?

If the radius of convergence is infinite, it means that the series converges for all values of X, as in the case of the series ∑(X^n / n!).

What is the interval of convergence of a power series?

The interval of convergence refers to the set of all X values for which the series converges. This interval can be finite or infinite, depending on the series.

Can a power series converge for only one specific value of X?

Yes, in some cases, a power series may only converge for a single value of X, in which case the radius of convergence is zero.

What is the significance of the ratio test when dealing with factorials in a power series?

When dealing with factorials in a power series, the ratio test is useful because it simplifies the expression and shows that the series converges for all X, as seen in the example of ∑(X^n / n!).