ADM1305 - Section 2.1 and Section 2.2
Summary
TLDRThis chapter on differentiation explores how to compute the derivative of a function, emphasizing its visual interpretation through graphs. The discussion covers key concepts such as increasing and decreasing functions, local extrema, and concavity, all linked to the slope of the tangent line. The video introduces the informal definition of the derivative and connects visual understanding with algebraic computation. It also defines critical points where the derivative is zero or undefined, providing visual cues for identifying these points on graphs. Ultimately, the chapter aims to equip viewers with the tools to analyze functions both visually and analytically.
Takeaways
- 📈 The derivative of a function provides the slope of the tangent line at a given point, indicating whether the function is increasing or decreasing.
- 🔍 Understanding derivatives visually is crucial for interpreting function behavior from graphs.
- ⚠️ A critical point occurs when the derivative is zero or undefined, indicating potential local maxima or minima.
- 🌄 Points where the slope of the tangent line is horizontal correspond to local maximum or minimum points on the graph.
- 🚧 Derivatives do not exist at corners, discontinuities, or vertical tangents, which are important in identifying critical points.
- 📊 A positive derivative means the function is increasing, while a negative derivative means it is decreasing.
- 🗺️ Visual analysis of graphs helps determine intervals where the function is increasing or decreasing.
- 🔄 The next step after visual understanding is to compute derivatives for functions expressed as formulas.
- 🌟 Recognizing the relationship between the slope of the tangent and the function's behavior is key to mastering calculus concepts.
- 📚 This chapter emphasizes the importance of transitioning from visual interpretations to algebraic computations in calculus.
Q & A
What is the primary goal of the chapter on differentiation?
-The primary goal is to compute the derivative of a function and understand its applications, particularly in graphing functions using the first and second derivatives.
How can one determine if a function is increasing or decreasing from its graph?
-By examining the slope of the tangent line at a point; if the slope is positive, the function is increasing, and if it is negative, the function is decreasing.
What does the notation f'(a) represent?
-The notation f'(a) represents the derivative of the function f at the point x = a, indicating the slope of the tangent line at that point.
What is the significance of critical points in a function?
-Critical points indicate where the derivative is zero or undefined, helping to identify local maxima, minima, and points of discontinuity.
What happens when the derivative f'(a) is zero?
-When f'(a) is zero, it indicates a horizontal tangent line at that point, which can suggest a local maximum or minimum.
How can you visually identify critical points on a graph?
-Critical points can be identified by looking for points where the slope of the tangent line is horizontal (f'(a) = 0), corners, or points of discontinuity.
What are the three cases where the derivative does not exist?
-The derivative does not exist at corners, discontinuities, or vertical tangents.
How does one determine if a function is concave up or concave down?
-Concavity can be determined by examining the second derivative; if the second derivative is positive, the function is concave up, and if it is negative, it is concave down.
What role do visual definitions play in understanding derivatives?
-Visual definitions help establish an intuitive grasp of concepts such as increasing/decreasing behavior and critical points before delving into algebraic computations.
What is the importance of the slope of the tangent line in relation to function behavior?
-The slope of the tangent line provides crucial information about the function's behavior at a point, indicating whether it is increasing, decreasing, or stationary.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

12.2 Finding the derivative (PURE 1- Chapter 12: Differentiation)
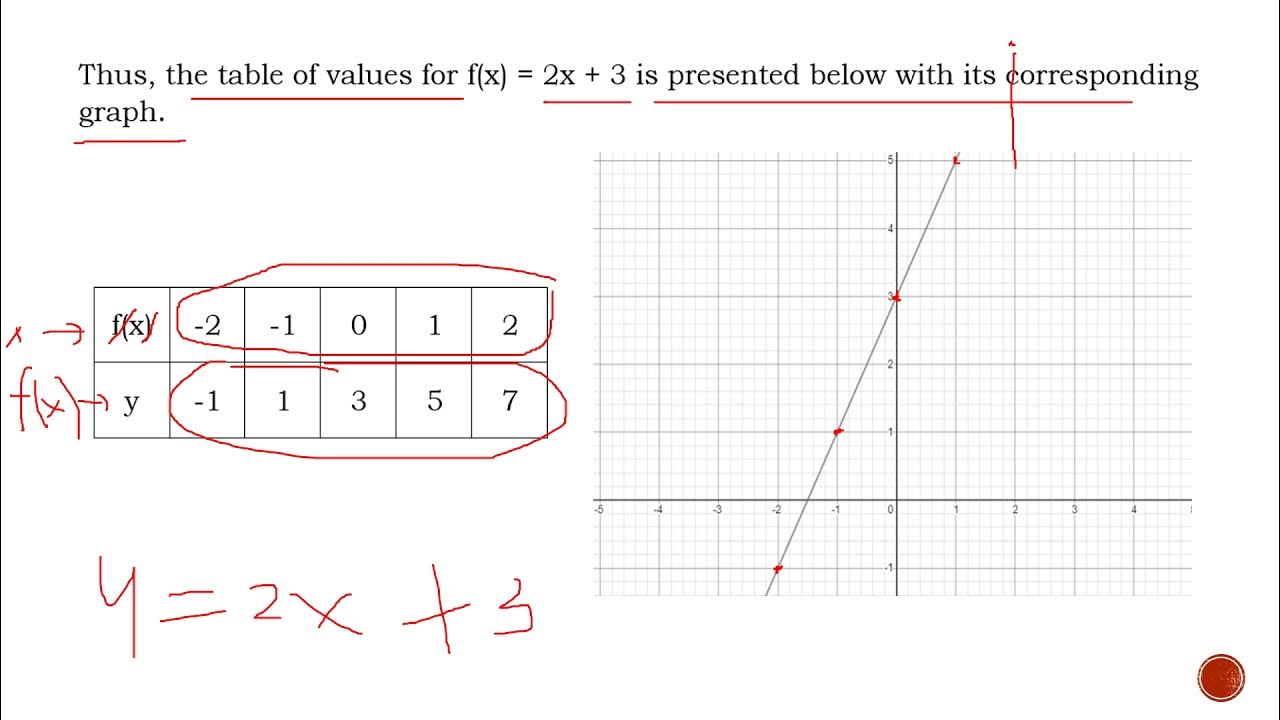
REPRESENTATIONS OF AN INVERSE FUNCTIONS | General Mathematics | Quarter 1 - Module 13

Übersicht f f´ f´´, Zusammenhänge der Funktionen/Graphen, Ableitungsgraphen | Mathe by Daniel Jung

Calculus- Lesson 8 | Derivative of a Function | Don't Memorise
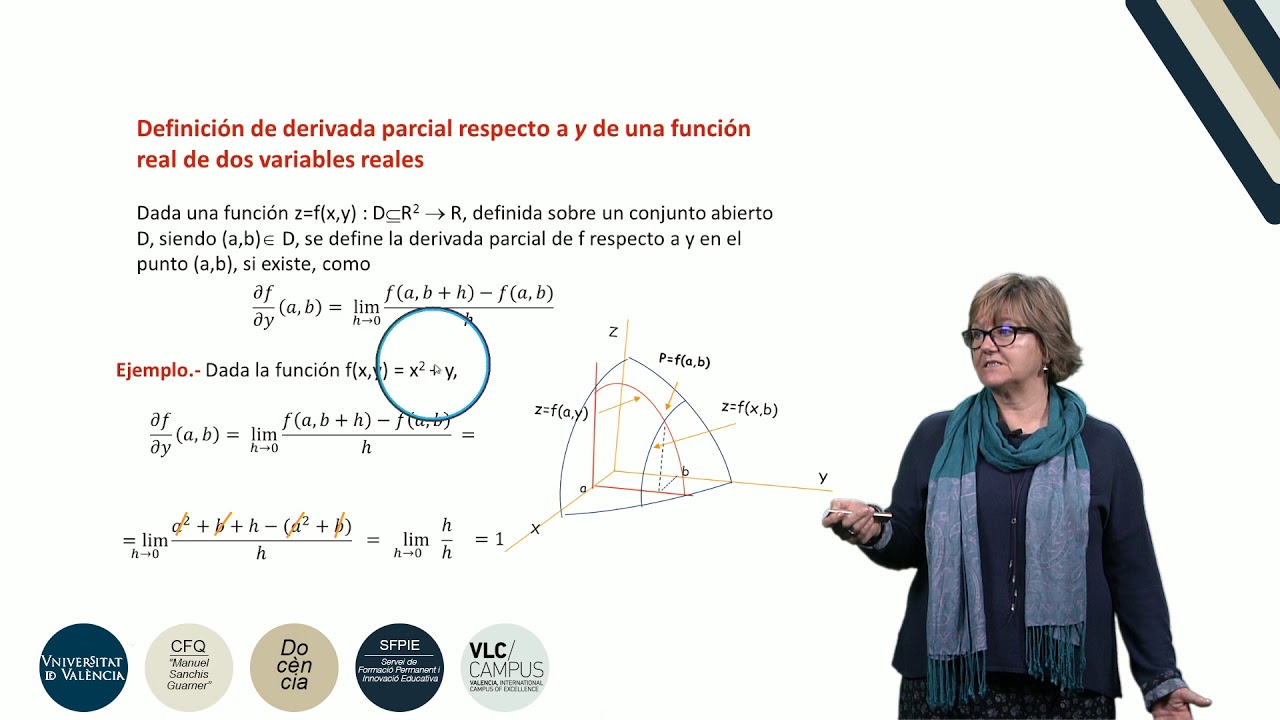
Derivadas parciales. Interpretación geométrica

Concavidad y puntos de inflexión para principiantes. Uso de la segunda derivada | Video 88
5.0 / 5 (0 votes)