79. Funciones linealmente independientes ¿qué son? CON EJEMPLOS
Summary
Please replace the link and try again.
Takeaways
- 😀 Linear independence of solutions in second-order linear differential equations is explained with clear examples.
- 😀 Two functions are linearly independent if one cannot be obtained by multiplying the other by a constant.
- 😀 A solution is linearly independent if no constant multiplication of one solution will result in another solution (e.g., y1 = e^(2x) and y2 = e^(3x)).
- 😀 If both constants (a and b) in a linear combination are zero, then the functions are linearly independent. Otherwise, they are dependent.
- 😀 An example is given using the functions y1 = x^2 and y2 = 5x^2 + 2x^2 to demonstrate linearly independent solutions.
- 😀 Functions that can be written as constant multiples of each other (e.g., y1 = e^(2x) and y2 = 4e^(2x)) are linearly dependent.
- 😀 For second-order differential equations, there are exactly two linearly independent solutions.
- 😀 A linear combination of two independent solutions (with arbitrary constants) forms the general solution to a second-order differential equation.
- 😀 Higher-order linear differential equations (third order, fourth order, etc.) will have as many linearly independent solutions as their order (e.g., third-order = three solutions).
- 😀 The video emphasizes the importance of finding linearly independent solutions when solving linear homogeneous differential equations, which can be done by combining solutions with arbitrary constants.
Q & A
What does it mean for solutions to be linearly independent in a second-order linear differential equation?
-Two solutions of a second-order linear differential equation are linearly independent if one cannot be obtained from the other by simply multiplying it by a constant. This means that neither solution is a multiple of the other.
What is the definition of linear independence in the context of differential equations?
-Two solutions y1 and y2 are linearly independent if there exist constants a and b such that the equation a * y1 + b * y2 = 0 only holds when a = 0 and b = 0. In simpler terms, if one solution cannot be written as a constant multiple of the other, they are linearly independent.
How can we determine if two functions are linearly independent or dependent?
-To determine if two functions are linearly independent, multiply each solution by a constant (a and b), then compare the results. If the only solution to the equation a * y1 + b * y2 = 0 is when both constants a and b are zero, the functions are linearly independent. If a non-zero value of a or b exists, they are dependent.
Can two solutions be linearly dependent even if the functions are different?
-Yes, two solutions can be linearly dependent if one can be expressed as a constant multiple of the other, regardless of whether the functions themselves look different. For example, e^(2x) and 4e^(2x) are linearly dependent because one is just a multiple of the other.
What is the significance of linearly independent solutions in solving differential equations?
-Linearly independent solutions form the basis of the general solution to a differential equation. In the case of second-order differential equations, two linearly independent solutions are enough to express the general solution, which is a linear combination of these two solutions.
What does the term 'general solution' refer to in the context of a linear differential equation?
-The general solution of a linear differential equation is the combination of all possible solutions that can be formed using two linearly independent solutions. This is expressed as a linear combination of the solutions with arbitrary constants.
Why is it important to understand the concept of linearly independent solutions in solving differential equations?
-Understanding linear independence helps identify the number of independent solutions to a differential equation. This is crucial for solving the equation and finding the general solution, which can represent a family of solutions based on different initial conditions.
How do we recognize if two functions are linearly dependent, with an example?
-Two functions are linearly dependent if one can be expressed as a constant multiple of the other. For example, if y1 = e^(2x) and y2 = 4e^(2x), these functions are dependent because y2 is simply 4 times y1.
Can a second-order linear differential equation have more than two linearly independent solutions?
-No, a second-order linear differential equation will always have exactly two linearly independent solutions. For equations of higher orders, like third-order, there will be three linearly independent solutions, and so on.
What role do constants play in forming solutions to a second-order linear differential equation?
-Constants, such as C1 and C2, are used to form the general solution of a second-order linear differential equation. These constants are arbitrary real numbers, and their values depend on the initial conditions of the problem being solved.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
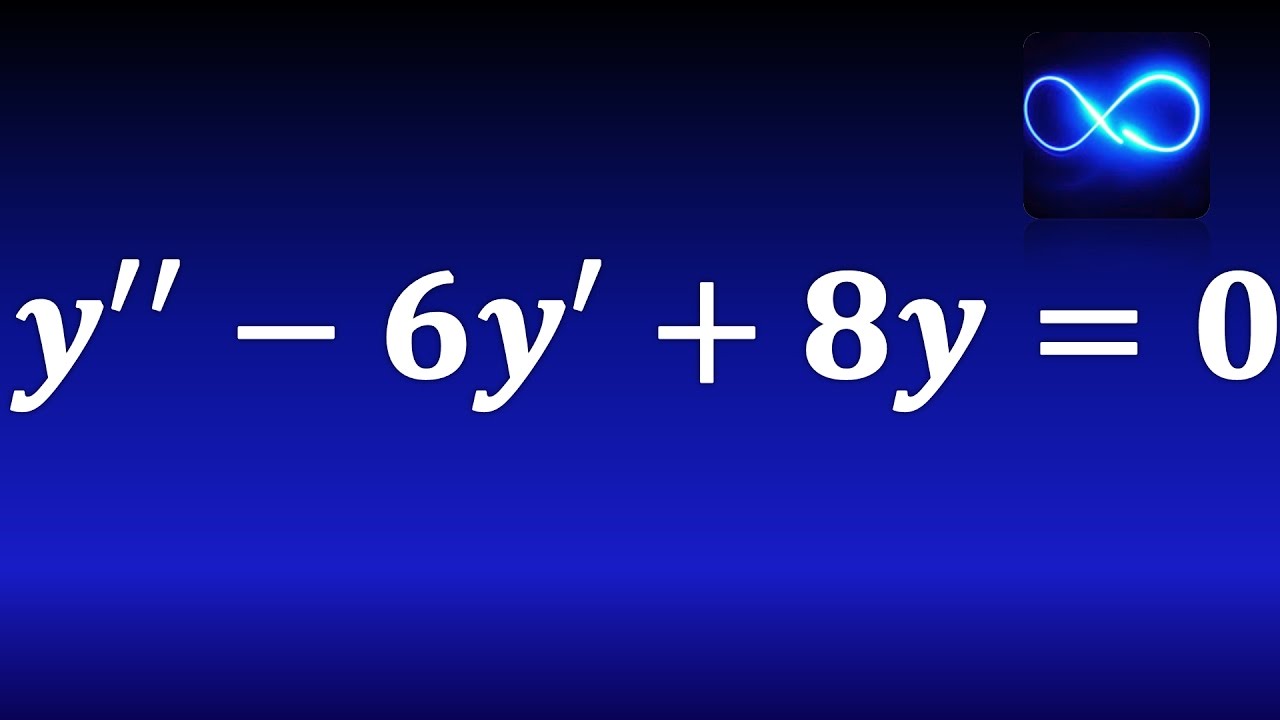
86. Ecuación diferencial de coeficientes constantes (segundo orden, homogénea) EJERCICIO RESUELTO

AMD T13 - Conjuntos linealmente independientes y generadores
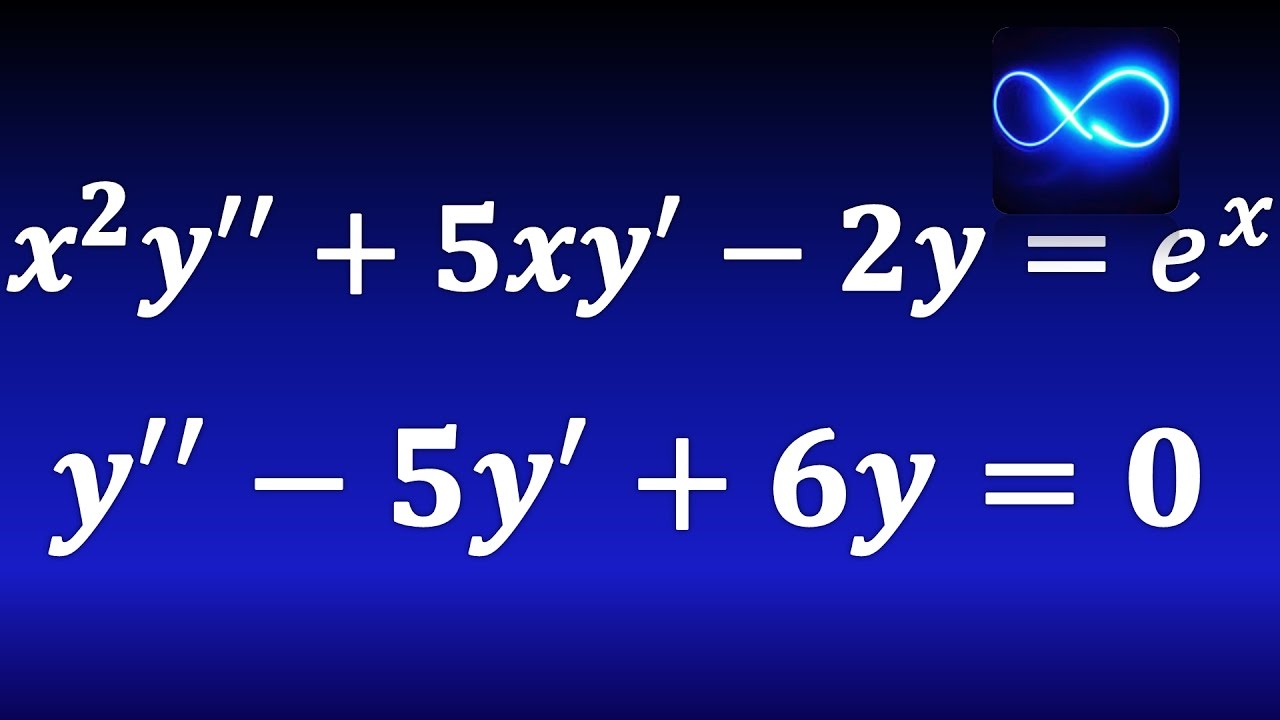
78. Qué son las ecuaciones de segundo orden, ecuaciones homogéneas y de coeficientes constantes
Práctica04 Ejercicio1

Pensamiento Matemático I – Progresión 5

DIFERENCIA ENTRE VARIABLE INDEPENDIENTE Y DEPENDIENTE | Metodología Básica (y no tan básica)
5.0 / 5 (0 votes)