PEMBUKTIAN RUMUS LOGIKA MATEMATIKA PADA PENALARAN UMUM UTBK 2020

Summary

The video covers mathematical logic, focusing on statements, truth values, and how they apply to solving problems. It explains different logical operators like conjunction ('and'), disjunction ('or'), and implication ('if p, then q'). The speaker demonstrates how to determine the truth value of statements using truth tables and discusses various logical equivalences. The video also includes examples and practice questions to help viewers understand how to apply these concepts in exams, particularly in general reasoning tests. The goal is to prepare students for their upcoming entrance exams in a clear and practical manner.

Takeaways

• 📚 The video focuses on mathematical logic, particularly on conditional statements and logical operations.
• 📝 The key concept discussed is the logical implication: 'If P, then Q' and its equivalence to other logical forms.
• 🧠 The presenter introduces logical operators such as conjunction (AND), disjunction (OR), implication (IF...THEN), and bi-implication (IF AND ONLY IF).
• 🔄 Negation (NOT P) and how it reverses the truth value of a statement is explained, highlighting its significance in logical reasoning.
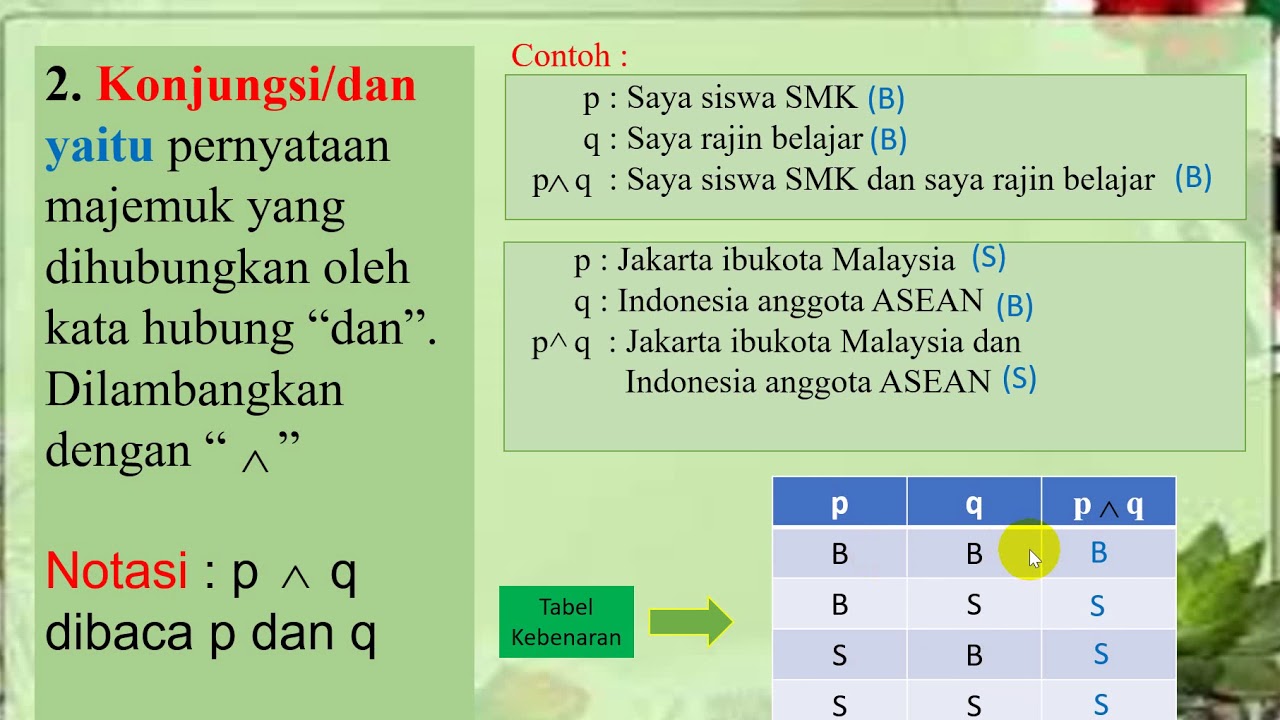
• ✅ The truth table for conjunction is explained: a conjunction of two true statements results in true, otherwise false.
• ☑️ The truth table for disjunction is also covered: a disjunction is true if at least one of the statements is true, and false only when both are false.
• 🔀 Logical equivalence of statements like 'P or Q' is explored, showing that it is equivalent to 'Q or P'. Similarly, 'P and Q' is equivalent to 'Q and P'.
• 🚫 The video addresses the common student question of whether 'If P, then Q' is equivalent to 'If not Q, then not P' (and proves that it is).
• 🧪 Examples of applying logical rules are provided, like solving logic problems involving conditions for doing an action (e.g., using soap or shampoo when washing a car).
• 💡 The video emphasizes understanding and using truth tables, logical negations, and implications to solve problems in quantitative reasoning and general logic exams.

Q & A

What is a statement in mathematical logic?

A statement in mathematical logic is a sentence that has a truth value, meaning it can be either true or false, but not both. For example, 'Jakarta is the capital of Indonesia' is a true statement.

What is the difference between a statement and a non-statement in logic?

A statement has a definite truth value (true or false), while a non-statement, such as 'Febri is handsome,' does not have a definite truth value because it is subjective and depends on personal opinion.

What is the 'negation of P' in mathematical logic?

The negation of P, symbolized as ¬P, means 'not P.' If P is true, ¬P is false, and vice versa. It is the logical opposite of the original statement.

What are the four types of compound statements in logic?

The four types of compound statements are conjunction (P and Q), disjunction (P or Q), implication (If P then Q), and biconditional (P if and only if Q).

When is a conjunction (P and Q) true?

A conjunction (P and Q) is true only when both P and Q are true. If either P or Q is false, the entire conjunction is false.

What is the truth table rule for disjunction (P or Q)?

A disjunction (P or Q) is true if at least one of P or Q is true. It is only false when both P and Q are false.

When is an implication (If P then Q) false?

An implication (If P then Q) is false only when P is true and Q is false. In all other cases, the implication is considered true.

What is the difference between implication and biconditional?

Implication (If P then Q) means that if P is true, Q must also be true. Biconditional (P if and only if Q) means P is true if and only if Q is true, meaning both P and Q must have the same truth value.

What is De Morgan's law in logic?

De Morgan's law states that the negation of a disjunction (P or Q) is equivalent to the conjunction of the negations (¬P and ¬Q), and the negation of a conjunction (P and Q) is equivalent to the disjunction of the negations (¬P or ¬Q).

Is 'If P then Q' equivalent to 'If not Q then not P'?

Yes, 'If P then Q' is logically equivalent to 'If not Q then not P.' This is known as contrapositive, and both forms have the same truth value.