Complex Power || Apparent Power || Real || Example 11.11 || Practice Problem 11.11 || ENA 11.6(E)
Summary
TLDRThis video explains the concept of complex power in electrical systems, using a power triangle to simplify the calculations of reactive and real powers. It explores how real power (P) and reactive power (Q) combine to form complex power (S), with the magnitude of S representing apparent power. Key formulas are discussed for calculating complex power using RMS values, voltage, current, and impedance. The video also covers how to find load impedance, power factor, and the types of circuits (capacitive or inductive) based on phase angles, with practical examples demonstrating these calculations.
Takeaways
- 📐 Complex power is the sum of real power and reactive power, visualized using a power triangle.
- ⚡ Real power is on the horizontal axis, reactive power is on the vertical axis, and their vector sum gives complex power.
- 💡 The magnitude of complex power is the apparent power, previously discussed in earlier sections.
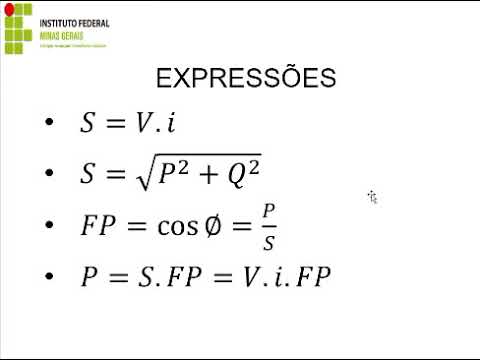
- 🔢 Complex power (S) is calculated as S = 1/2 V * I conjugate, and it can be expressed in terms of RMS values.
- 📏 Complex power consists of real power (P) and reactive power (Q), where the real part represents real power, and the imaginary part represents reactive power.
- 🧮 Real power (P) is given by V_RMS * I_RMS * cos(theta), with the unit in watts, while reactive power (Q) is V_RMS * I_RMS * sin(theta), measured in VAR.
- 🔄 Apparent power is the magnitude of complex power, which can be calculated using the Pythagorean theorem: S = sqrt(P^2 + Q^2).
- 🧲 The load impedance (Z) is V_phasor / I_phasor and can be calculated in terms of RMS values and phase angles.
- 🧑🏫 Power factor is cos(theta) and helps determine if the power is leading or lagging. A negative angle indicates a capacitive circuit, while a positive angle indicates an inductive circuit.
- 🔍 Through examples, the script explains how to compute complex power, apparent power, real power, reactive power, power factor, and load impedance based on voltage and current.
Q & A
What is complex power?
-Complex power is a way to represent the real and reactive power in a single complex number. It is used to simplify the calculation of these powers. It is represented as the sum of real power (P) and reactive power (Q), where the real part of the complex number is the real power and the imaginary part is the reactive power.
How is complex power calculated?
-Complex power (S) is calculated using the formula S = V*I*conjugate(I), where V is the applied voltage phasor, and I is the current phasor. This can also be written in terms of RMS values as S = V_RMS * I_RMS * conjugate(I_RMS).
What is the difference between real power and reactive power?
-Real power (P) represents the average power consumed by the load and is measured in watts (W). Reactive power (Q) represents the power that oscillates between the load and the source without conversion to heat and is measured in volt-amperes reactive (VAR).
What is the unit of complex power?
-The unit of complex power is volt-amperes (VA), which is the product of RMS voltage and RMS current.
How is apparent power related to complex power?
-Apparent power is the magnitude of the complex power. It is calculated as the square root of the sum of the squares of the real power and reactive power.
What is the significance of the power triangle?
-The power triangle is a graphical representation of real power, reactive power, and apparent power. It helps in understanding the relationship between these powers and the power factor.
How can you determine if a load is capacitive or inductive from the complex power?
-A load is capacitive if the reactive power is negative (Q < 0), meaning the current leads the voltage. A load is inductive if the reactive power is positive (Q > 0), meaning the current lags the voltage.
What is the power factor and how is it calculated?
-The power factor is the cosine of the angle between the voltage and current phasors. It is calculated as the ratio of real power to apparent power (P/S) or as cos(theta_V - theta_I), where theta_V and theta_I are the angles of the voltage and current phasors, respectively.
How can you find the load impedance from complex power?
-Load impedance (Z) can be found from complex power using the formula Z = V_RMS / I_RMS, where V_RMS is the RMS voltage and I_RMS is the RMS current. The angle of the impedance is theta_V - theta_I.
What is the significance of the angle between voltage and current?
-The angle between voltage and current (theta_V - theta_I) determines the power factor and indicates whether the load is capacitive (leading) or inductive (lagging). A positive angle indicates lagging, and a negative angle indicates leading.
How do you calculate the maximum current from apparent power?
-The maximum current (I_max) can be calculated from apparent power using the formula I_max = sqrt(2) * I_RMS, where I_RMS is the RMS current. This is derived from the relationship between apparent power (S) and RMS values of voltage and current.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video
Fator de Potência / Correção do FP

What is Apparent Power ? || Example & Practice11.9 || Example & Practice 11.10 || ENA 11.5(English)

Power Triangle and Impedance Triangle

¿Qué son las potencias Activa, Reactiva y Aparente? ⚡ Triángulo de Potencias

Power factor explained | Active Reactive Apparent Power correction

Circuitos Trifásicos Equilibrados e Ligação Estrela e Triângulo (#65)
5.0 / 5 (0 votes)