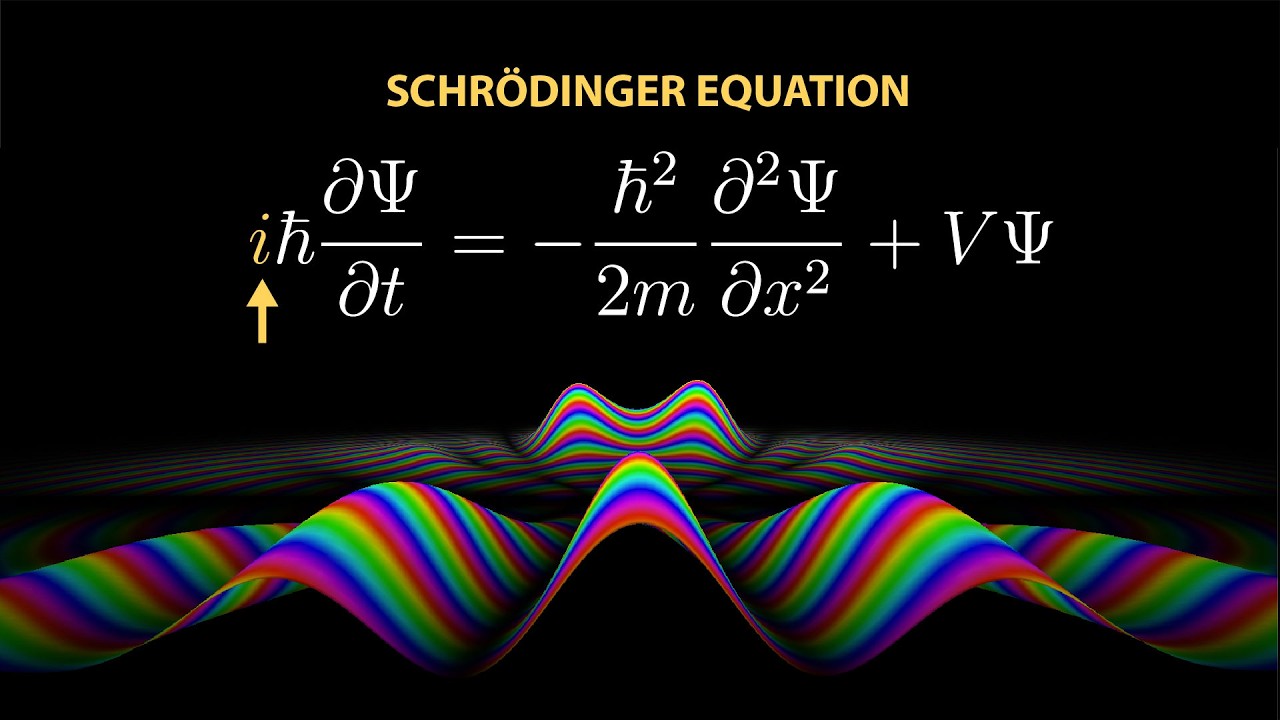
Complex Numbers in Quantum Mechanics

Summary

This video script delves into the intricacies of quantum mechanics, particularly the use of complex numbers to represent wave phenomena. It explains the two-dimensional nature of complex numbers as a generalization of positivity and negativity, illustrating their relevance through the analogy of waves. The script further explores how complex numbers facilitate the understanding of wave interference, Fourier analysis, and quantum systems like the quantum harmonic oscillator. It also touches on the profound implications of complex numbers in quantum electrodynamics, leaving viewers intrigued by the deep connections between mathematics and the physical world.

Takeaways

• 🧠 Quantum mechanics is complex due to the counterintuitive phenomena it describes and the use of complex numbers.
• 🔢 Complex numbers are two-dimensional, allowing for the representation of magnitude and direction, which is essential for wave functions in quantum mechanics.
• 🌀 Complex numbers generalize the concept of positivity and negativity, enabling the representation of numbers that are neither purely positive nor negative, akin to points on the unit circle.
• 🌊 Complex numbers are particularly useful for describing waves, as they can capture both the amplitude and phase of oscillations.
• 📉 The real and imaginary parts of a complex number can represent different aspects of a wave, such as the up and down or left and right movements.
• 📚 Euler's formula, \( e^{i\theta} = \cos(\theta) + i\sin(\theta) \), connects complex exponentials with trigonometric functions and is fundamental in understanding wave behavior.
• 🔍 Complex addition and multiplication are analogous to vector addition and the combination of amplitudes and phase shifts, respectively.
• 🌐 The multiplication of complex numbers by a unit length complex number with a varying phase angle can result in phase shifts of the original wave function.
• 📐 Fourier analysis uses complex numbers to construct arbitrary waveforms by adding sine and cosine waves, which is also applicable in quantum mechanics for superpositions.
• 🌌 In quantum mechanics, complex numbers are used to describe quantum states through superpositions of energy eigenfunctions, which can result in time-varying probability densities.
• 🔬 Quantum field theory utilizes plane waves as a basis to construct more complex quantum systems and is integral to the formulation of quantum electrodynamics.

Q & A

Why are complex numbers used in quantum mechanics instead of just real numbers?

Complex numbers are used in quantum mechanics because they allow for the representation of two-dimensional phenomena, such as the amplitude and phase of waves, which are fundamental in describing quantum states and wave functions.

What is the defining feature of complex numbers according to the script?

The defining feature of complex numbers is that they are two-dimensional, allowing for the representation of both magnitude and direction, which is essential for capturing the behavior of waves in quantum mechanics.

How does the script explain the concept of complex numbers in relation to real numbers?

The script explains that complex numbers can be seen as a generalization of the positive and negative binary system of real numbers, where a complex number can exist on any number ray between positive and negative, not just on the two discrete options of the real number line.

Outlines

00:00

😲 The Complexity of Quantum Mechanics and Complex Numbers

The paragraph delves into the intricacies of quantum mechanics, highlighting the perplexing phenomena that challenge conventional understanding. It emphasizes the initial confusion surrounding the use of complex numbers in physics, questioning their necessity over real numbers. The explanation unfolds to reveal the two-dimensional nature of complex numbers, contrasting them with the one-dimensional real numbers. The author illustrates how complex numbers generalize the concept of positivity and negativity, allowing for a continuous range of directions in the complex plane. The analogy of waves is introduced to demonstrate how complex numbers can capture the essence of oscillating phenomena, such as sound or light waves, more effectively than real numbers.

05:00

📊 Euler's Formula and the Wave Representation of Complex Numbers

This section explores the representation of waves using complex numbers, focusing on Euler's formula as a pivotal tool in understanding wave dynamics. The paragraph explains how the real and imaginary parts of a complex number can be visualized as waves, with the real part representing the wave's position in the complex plane and the imaginary part indicating its phase shift. The importance of complex addition and multiplication in wave interference is introduced, setting the stage for a deeper exploration of how these mathematical operations relate to physical phenomena such as constructive and destructive interference.

10:01

🌀 The Role of Complex Numbers in Wave Interference and Fourier Analysis

The paragraph examines the application of complex numbers in wave interference and Fourier analysis. It discusses the process of adding complex numbers as akin to vector addition, leading to constructive or destructive interference patterns. The concept of multiplying complex numbers is also explored, showing how it affects the amplitude and phase of waves. The paragraph further illustrates how complex numbers can be used to generate arbitrary waveforms through the superposition of sine and cosine waves, drawing parallels with quantum mechanics and the use of basis functions in quantum systems.

15:02

🌌 Higher Dimensional Wave Functions and Complex Numbers in Quantum Mechanics

This section expands on the concept of complex numbers in higher-dimensional wave functions, using the example of a two-dimensional plane wave. It clarifies misconceptions about the physical representation of complex numbers and emphasizes their role in capturing the dynamics of waves in quantum mechanics. The paragraph also touches on the use of plane waves as a basis for constructing more complex waveforms in quantum systems.

Keywords

💡Quantum Mechanics

Quantum Mechanics is a fundamental theory in physics that describes the physical properties of nature at the scale of atoms and subatomic particles. In the video, it's the central theme, as the script discusses the complexities and phenomena that occur at the quantum level, challenging traditional notions of reality. The script mentions 'crazy phenomena' in the quantum world, indicating the non-intuitive nature of quantum mechanics.

💡Complex Numbers

Complex numbers are numbers that consist of a real part and an imaginary part, usually written as a + bi, where 'a' and 'b' are real numbers and 'i' is the imaginary unit with the property that i^2 = -1. The script explains the necessity of using complex numbers in quantum mechanics to represent wave functions and their properties, such as amplitude and phase.

💡Wave Function

A wave function in quantum mechanics is a mathematical description of the quantum state of a system. The script uses the term to illustrate how particles in quantum mechanics are described by waves, and it discusses the use of complex numbers to represent these wave functions, which can exhibit interference patterns.

💡Amplitude

Amplitude refers to the magnitude or height of a wave, which in the context of the script, is used to describe the strength of a wave function. It's mentioned in the explanation of complex numbers representing waves, where the amplitude is constant, and only the phase changes.

💡Phase

Phase is a term used to describe the position of a point in time on a waveform, which can be thought of as the 'angle' in the complex plane. The script explains how phase is crucial in representing wave functions and how changes in phase can lead to constructive or destructive interference.

💡Euler's Formula

Euler's Formula is a mathematical formula that relates the exponential function with trigonometric functions and is written as e^(iθ) = cos(θ) + i*sin(θ). The script introduces Euler's Formula as a way to understand the relationship between complex numbers and waves, particularly in the context of quantum mechanics.

💡Fourier Analysis

Fourier Analysis is a method used to decompose a signal or function into its constituent frequencies. In the script, it's mentioned as a way to generate arbitrary waveforms by adding sine and cosine waves, which is analogous to how complex numbers can be used to create more complex wave functions in quantum mechanics.

💡Quantum Harmonic Oscillator

The Quantum Harmonic Oscillator is a model system in quantum mechanics that describes the behavior of a particle in a potential well that increases quadratically with distance. The script briefly mentions it as an example of using basis functions and superpositions in quantum mechanics.

💡Probability Density

In quantum mechanics, probability density is the amount of probability per unit volume. The script explains that the probability density of a particle can be found by integrating the square of the wave function (|ψ|^2) over a given volume, which is related to the concept of 'ψ*ψ' in the context of quantum mechanics.

💡Superposition

Superposition is a fundamental principle in quantum mechanics stating that any two (or more) quantum states can be added together, or 'superposed', and the result will be another valid quantum state. The script discusses how superposition leads to the time-varying probability densities when different energy eigenstates are added.

💡Complex Conjugate

A complex conjugate of a complex number is obtained by changing the sign of the imaginary part while keeping the real part the same. In the script, it's mentioned in the context of multiplying a complex number by its complex conjugate, which results in a real number, and is related to the probability density in quantum mechanics.

Highlights

Quantum mechanics involves complex numbers due to the two-dimensional nature of waves, challenging traditional real number systems.

Complex numbers are essential for capturing wave phenomena, such as the phase and amplitude changes in quantum systems.

The complex plane allows for a more nuanced representation of waves, beyond the simple positive and negative of real numbers.

Euler's formula, e^(iΘ) = cos(Θ) + i*sin(Θ), provides a bridge between complex numbers and wave functions in quantum mechanics.

Complex numbers are not just mathematical tools but are intrinsic to the physical description of quantum phenomena.

The concept of complex addition and multiplication is analogous to wave interference, with direct implications for quantum systems.

Complex numbers enable a unified approach to Fourier analysis, simplifying the representation of waveforms in quantum mechanics.

The quantum harmonic oscillator can be described using superpositions of energy eigenstates, demonstrating the utility of complex numbers.

Complex conjugates play a crucial role in quantum mechanics, particularly in calculating probability densities from wave functions.

The two-dimensional aspect of complex numbers does not correspond to physical space but to the directional properties of waves.

Plane waves are fundamental in quantum mechanics, serving as a basis for constructing more complex wave functions.

Complex numbers are not just an abstraction but have a tangible interpretation in terms of wave dynamics in quantum systems.

The video hints at a profound connection between local U(1) symmetry and electromagnetism in quantum electrodynamics, to be explored in future content.

Complex numbers are integral to understanding the quantum mechanical description of particles and their interactions.

The video emphasizes the importance of gaining intuition for complex numbers through practice and problem-solving in quantum mechanics.

The profound implications of complex numbers in quantum mechanics are only briefly touched upon, with deeper exploration promised for future videos.

Transcripts

quantum mechanics is a notoriously

complicated and confusing subject and

part of that is for good reason I mean

there really are these crazy phenomena

happening in the quantum worlds that are

challenging to imagine but uh one of the

reasons quantum mechanics is complicated

is that there are all these complex

numbers all over the place and at first

when you're getting into the subject

it's very confusing why are we using

complex numbers what do they mean why

can't we use real numbers isn't this

physics not math what why do we use

these crazy numbers right so that was

the source of confusion for me at least

for quite a long period of time and then

one day it clicked and I finally

understood and I was like oh okay yeah

actually that complex numbers are what

we want to use in quantum mechanics so

what are the complex numbers anyway

I think the most defining feature of the

complex numbers is that they're a

two-dimensional number and that seems

scandalous but if you've been using the

real number line then you're already

kind of complicit in using a

two-dimensional number system sort of

because when you write a real number you

write its magnitude but you also assign

it to one of the two number Rays either

positive or negative

so you already have this sense of

magnitude and direction in your number

system it's just that you only have two

options for the direction positive or

negative so the direction dimension of

the real numbers is just a discrete

binary thing rather than a continuous

thing

and all the complex numbers really are

is a generalization of that positive

negative binary that is the complex

numbers can be regarded as a

generalization of positivity and

negativity so that a number can be not

only on either the positive or negative

number Ray but also all of the number

rays in between

this is a very strange concept the first

time you see it

now if you're mostly used to using

numbers to count things this seems like

an affront to reason because after all

if you consider the number two for

example so you could have positive two

you could have negative two

but with the complex numbers you could

also have this two that's just somewhere

in this space of this somewhere in this

circle that has radius two the number

with magnitude 2 in the complex plane

can be at any one of these points

and that doesn't seem right because

you'll notice when two is up just purely

pointing straight up then it's neither

positive nor negative and yet it's still

two it still has the amplitude of two

uh that doesn't really fit into our

normal intuitions about counting right

it doesn't feel like it makes sense okay

but complex numbers are not about

counting in this kind of way

let's look at this

this is a wave what kind of wave I don't

know could be the surface of the ocean

it could be a sound wave where the

height represents the air pressure it

could be a wave of light just flopping

around in the electromagnetic field

whatever it is it's just a wave now this

is a very clean and pure wave that I'm

using to illustrate the point but it's a

wave nonetheless

so how can we use numbers to capture

what this wave is

well the first and most obvious thing is

if the wave is above the average level

if the wave is up we'll say it's a

positive number and if the wave is down

we'll say it's a negative number so

let's go ahead and color it in positive

and negative all right fair enough

that's not wrong

but let's pause time for a second

now look here

right at the point where the wave is

zero and going down

is that point really zero or does that

point exist in a harmonious Continuum

with the rest of the wave yeah it's zero

now but it's part of a bigger picture

and you know it's just gonna be changing

soon you know it'll soon be non-zero so

is that really zero in the same way that

a flat line is zero or does it somehow

have an amplitude even though it's also

kind of zero at the same time so do you

see an analogy between this thing that's

kind of zero and kind of not zero and

the example we were looking at earlier

when the two was pointing straight up

and it was also kind of zero and kind of

not zero now what's more look at this

point over here where it's also zero but

now it's going up all of the same

observations apply it's kind of zero but

it's not really it has some energy to it

even though it's zero it kind of is kind

of isn't so now we can see that this

number here is the opposite of the

previous number that we were looking at

because the previous one is on its way

down and this one is on its way up

let's transform our perspective and use

complex numbers to represent this wave

[Music]

this is what a complex wave looks like

notice that the amplitude is constant

and it's just the phase that's changing

so it's not moving up and down like the

other wave now this is a very pure wave

this is a wave of the form e to the I

Theta where in this case Theta is some

function of X and some function of time

now let's plot the real part of this

complex number that is how far left or

right the number is in the complex plane

and you'll see that we can recover that

wave we were looking at earlier

let's show the imaginary component of

this complex wave that is how far up and

down the wave is in the complex plane

and you'll notice here we get a wave

that looks very similar to the real part

but it's out of phase such that it takes

on maximum and minimum values when the

real part is at zero and vice versa by

the way the function e to the I Theta is

equal to cosine of theta plus I times

the sine of theta where I is the

imaginary unit this equation is known as

Euler's formula well one of his many

formulas and it gives us another way of

thinking about what this complex wave is

when you're first getting into complex

numbers you'll probably think about

Euler's formula as the definition of

what e to the I Theta is but as you

become more comfortable with e to the I

Theta you'll eventually just see that as

the wave and then the cosine and sine is

a way of splitting it up into the real

Parts in the imaginary part by the way

let me just quickly say on the topic of

the imaginary part imaginary numbers are

a misnomer okay they're just as real or

just as imaginary as the real number

members the complex numbers are

numerical structure they're a holistic

thing you know it doesn't make any sense

to say imaginary and real but whatever

this is the terminology you're stuck

with so it is what it is ultimately it's

a consequence of the fact that the

imaginary numbers were named before they

were understood and that's I think one

of Descartes greatest mistakes well that

in dualism but um anyway where were we

what are we talking about here to

understand why it's useful to be able to

represent a wave as a constant amplitude

complex number whose phase is changing

we'll have to take a look at the nature

of complex addition multiplication and

how this relates to wave interference

we'll do that in a moment but first I

want to make a quick comment about why

is it e to the I Theta gives us this

wave I don't have time in this video to

give a really satisfactory answer but I

can lead you in the right direction so

if you take derivatives of e to the I

Theta and and sine of theta and cosine

of theta you can write these functions

in terms of a Taylor series when you do

that you'll find that e to the I Theta

has a term of theta at every degree

whereas cosine has even terms and sine

has odd terms and if you look closely at

these series you can see that the terms

on the right hand side of the equation

zip together into the terms on the left

hand side of the equation so by taking

Taylor series you can prove to yourself

that in fact e to the I Theta is cosine

of theta plus I sine of theta

okay so that was a bit of a tangent but

I think it's important for you to know

now let's take a look at complex Edition

if we have any two complex numbers we

can add them just like their vectors so

we put them tail to tip or another way

of looking at it is the sum is the

diagonal of the parallelogram so here

I'm showing two complex numbers both of

which have magnitude 2 swinging around

in the complex plane

the complex number between them is their

sum and you'll see that since the

numbers both have magnitude 2 their sum

has a magnitude of anywhere from zero to

four zero when the two numbers are

perfectly out of phase four when the

numbers are perfectly in phase and some

intermediary value when the angles are

kind of in phase and kind of not in

phase and we'll see later how that has a

very close relationship to the idea of

constructive and destructive

interference in Waves by the way here's

the algebraic formula for complex

Edition and that's the same as adding

vectors like the animation shows

now let's let one of those twos become a

little bit longer and you can see a more

General representation of complex

addition and you still see this effect

where sometimes the numbers will align

with each other and will add it's the

magnitude sometimes they'll be

oppositely aligned and they'll sort of

destructively interfere so that's a

general phenomenon whenever you're

adding complex numbers

and we can also multiply any two complex

numbers so to multiply complex numbers

you multiply their amplitudes and you

add their phase angles relative to the

positive real number line so here I'm

showing a couple numbers both with

magnitude two they're swinging around in

the complex plane and I'm also showing

their product you'll notice that since

the two numbers both have magnitude 2

their product will always have magnitude

4 but the phase angle of their product

depends on the sum of the phase angles

of the individual twos and of course

that rule generalizes so any two complex

numbers to multiply them you multiply

their magnitudes and add to their phase

angles that's really useful because what

it means is that if we have a complex

number of unit length but some phase

angle in the plane we can multiply that

by some other complex number to shift

its phase by the unit 1 numbers phase

angle to demonstrate this idea of

rotating the phase of a complex number

by multiplying by a unit length complex

number consider the illustration that's

on your screen now here I have a blue

wave and that's the real part of the

function e to the i x so the classic

complex wave amplitude 1 function you

take the real part and it's basically

it's just cosine of x right now the wave

that's changing colors that's the same

function that's the real part of the

same function except now the function is

multiplied by some complex constant

let's call it a a has magnitude one but

its phase angle is changing so I'm

showing you here the blue line with the

dot at the end that's the number one in

the complex plane right the colorful

line with the dot at the end that's a

that's this unit length complex number

whose phase is swinging around and the

colorful wave that's changing color

that's the real part of the wave that

you get when you multiply by the complex

number a

now let's notice something when a is one

the two numbers overlap and the two

waves are the same

when a is negative one the two waves are

completely opposite so the sign of the

wave is switching at every moment what

was formerly up is now down and so on

but in all those angles in between the

waves are not just the same or not just

totally opposite but they're similar

their phase shifted by some amount

that's not a complete half wavelength

and so in this illustration you can see

how this notion of generalizing

positivity and negativity that we see in

the complex numbers actually has a

genuine natural a very real

interpretation we can see this even more

clearly if we put the sum of the two

waves into this illustration as well

now we can think about addition of waves

in two ways first you can sweep along

the x-axis and just add the value of the

two waves at any point and that gives

you the value of the third wave or you

can add the complex amplitudes of the

Waves you get a resulting complex number

that's the sum of those complex numbers

and then you multiply that by the way of

e to the IX and that gives you the sum

of the two waves so you see there's this

direct one-to-one relationship between

complex addition and the interference of

these waves

if you've studied signal processing then

you know that you can generate an

arbitrary waveform by adding sine waves

and cosine waves in the right amounts

and frequencies while complex numbers

let us create a more unified and

holistic way of doing Fourier analysis

by adding waveforms that are these e to

the i x kind of waves multiplied by a

complex coefficient and then summing

over frequencies and amplitudes in that

basis so the example I'm showing here of

generating a square wave you might

associate that more with like signal

processing or something in quantum

mechanics however we use the idea of

basis functions and superpositions all

the time consider for example the

quantum harmonic oscillator I already

made a video on the quantum harmonic

oscillator so I'm not going to rehash

all of the details here if you want to

see like the hamiltonian and Trojan's

equation and all that good stuff you can

check out that video but what I just

want to point out here is that we can

take this sum of energy eigenfunctions

the ground state and these few excited

States and if we add them all up we can

get the wave function of a particle

that's oscillating in the quantum

harmonic oscillator

and this is just one of the many ways

that these basis functions can be added

but when you look at it one thing to

notice is that if you just look at the

probability densities of each of the

energy eigenstates they're actually

stationary but when you add the

eigenstates because you're adding the

complex numbers and there's that complex

interference going on the subsequent

probability density of the sum of those

States the superposition of those States

is actually this thing that varies in

time

and so here we can see this cool kind of

Dynamics coming out of the Machinery of

complex numbers

my main point in this video is just to

get you familiar with the complex

numbers to show you that ultimately they

come from this notion of waves and we'll

see many examples of complex wave

functions going forward for example I'm

currently working on a video on the

hydrogen atom and so here I'll show you

just a little preview of that we have

the longitudinal component of the

hydrogen energy eigenstates so this is

what you get when you solve the

azimuthal equation you end up with the

hemholz differential equation and you

can derive the fact that hydrogen has

this quantized magnetic number M which

we learn about in chemistry from the

fact that the wave function has to loop

back in on itself as you go around

anyway we'll come back to this later in

the hydrogen video but for now I'd like

to look at a higher dimensional example

of a complex wave function

so for example here's a two-dimensional

plane Wave It's defined in the plane of

your screen it's constant amplitude and

the color represents the phase of the

wave function at every point

I've also superimposed these little

arrows and what the arrows represent is

numbers in the complex plane

now I want to use this to illustrate a

couple of points first when you look at

a picture like this it almost looks like

a vector field and there's a temptation

to think that the complex numbers are

embedded in this two-dimensional space

and that their direction is sort of

pointing in a direction in that space

this is a common misconception and I had

this misconception for a while when I

was learning quantum mechanics because

one of the things that confused me about

complex numbers was they're

two-dimensional right so why

I mean if you have a three-dimensional

wave function for example shouldn't you

have like some kind of three-dimensional

thing like how do you stick a

two-dimensional Arrow at a point in

space how does that even make sense but

I hope that based on everything you've

seen so far you realize that the

two-dimensionality of the complex

numbers actually is not about any

direction in physical space

the fact that the complex numbers are

two-dimensional is the fact that a wave

is up and down or left and right or back

and forth or high pressure low pressure

it's yin and yang when you see that then

the confusion goes away okay so that's

the first point the second point I want

to bring up when it comes to plane waves

is that these things you will see these

all over the place why a couple of

reasons one honestly it's kind of one of

the easiest solutions to all these wave

equations that you'll encounter in

quantum mechanics but also it can be

used as a Fourier basis to construct

these more complicated wave functions

like earlier when we looked at the

square wave and you could see how you

can make an arbitrary waveform by adding

a bunch of waves well if you take a

bunch of plane waves that satisfy for

example the Schrodinger equation or the

Klein Gordon equation or the Drac

equation although in that case you have

byspinner field it's more complicated

but whatever if you have plane waves

that satisfy some differential equation

and you add them you take their

superposition you can create these more

complicated systems that also satisfy

those equations and in fact in Quantum

field Theory the plane waves play a

essential constitutive role in the

second quantization that allows you to

actually make a Quantum field Theory

oh I almost forgot but earlier when we

were looking at the complex

multiplication let's go back to that

picture except now I'm showing you

something special so these are two

complex numbers that have amplitude 2

and their product has amplitude four but

notice this time the two numbers are

complex conjugates of one another that

means that the imaginary component has

flipped sine in other words it's been

mirrored about the real axis and so what

we're seeing here is a number of times

its complex conjugate and the result is

always stuck on the real number line why

is that well add the angles a complex

number and its complex conjugate always

have angles that add up such that you

get back on the real axis and for that

reason you'll often see the expression

PSI star PSI as a way of expressing the

amplitude squared of a complex number in

quantum mechanics PSI star and PSI are

very often the two slices of bread in an

operator sandwich but when you see PSI

star PSI without any operator in between

just think of that as amplitude squared

and by by the way if PSI is a wave

function then PSI star PSI is the

probability density relating to that

wave function so if you want to find

what's the probability of finding a

particle in some volume of space you

just integrate PSI star PSI over that

volume of space that gives you the

probability of finding the particle

there

okay I'm gonna end this video on a

cliffhanger by alluding to one of my

favorite ideas of all time which is

beautiful and profound and it relates to

the complex numbers and that is the idea

that in Quantum electrodynamics a local

U1 symmetry of the wave function implies

electromagnetism this is going to take a

while to unpack and I am going to come

back to this in a future video it's

probably going to take like an hour but

we're really going to get into it and

it's going to be awesome for now I'll

just show you these equations if you

know you know if you don't stay tuned

but it's a pretty profound concept

basically what it comes down to is that

when you're doing Quantum

electrodynamics you have a wave function

it's actually kind of four-way functions

in one but if you impose the condition

that you can swing your wave function

around in Phase space arbitrarily then

what you'll find is that in order to

keep the lagrangian density the same

that is in order to not affect the laws

of physics and Quantum electrodynamics

you need the gauge symmetry of the

electromagnetic 4 potential which is

what we actually see right so the four

potential is kind of like relativistic

voltage and there's this inherent tree

as a result of that which has to do with

the way that the derivatives not Bonds

the electrical Community Fields anyway

if there's a lot there I'm not going to

go into it now but just know that there

are really deep and profound ideas

relating to the complex numbers in

quantum mechanics this video today

really has only scratched the surface

but I hope it's given you at least some

intuition for the complex numbers

one final thing that I want to say

becoming familiar with the complex

numbers takes time it takes hours and

hours of like plotting equations and

solving problems and doing things so

you're not supposed to get the ideas

right away no one ever does but uh you

know hey a journey of a thousand miles

is you know one footstep at a time or

however that saying goes and I hope you

keep on going on that path because

physics is really one of the most

wholesome things a person can do I think

but maybe I'm biased all right well

that's the video hope you enjoyed it and

have a great day