Fractions Basic Introduction - Adding, Subtracting, Multiplying & Dividing Fractions

Summary

This lesson focuses on adding, subtracting, multiplying, and dividing fractions. It explains step-by-step methods to find common denominators, add or subtract fractions, and simplify the results. The lesson also covers how to handle multiple fractions, find the least common denominator, and provides useful tricks to break down large numbers for easier calculations. Additionally, the 'keep-change-flip' technique is introduced for dividing fractions. Through various examples, the lesson demonstrates efficient methods to solve fraction problems, both with simple and complex numbers, without needing a calculator.

Takeaways

• 🔢 The process of adding fractions involves finding a common denominator by multiplying the denominators.
• ✖️ To add fractions like 3/5 and 4/7, multiply the denominators (5 and 7) to get a common denominator of 35, then adjust the numerators accordingly.
• ➖ When subtracting fractions like 7/8 - 2/9, the same principle applies: find a common denominator, subtract the adjusted numerators, and simplify if possible.
• 🔄 The least common denominator (LCD) is essential when adding or subtracting multiple fractions, and it can be found by listing multiples or multiplying denominators together.
• 📉 When combining three fractions, first find the least common denominator by listing multiples or multiplying the denominators (e.g., for 3/4 + 5/3 - 7/2, the LCD is 12).
• ➕ Once a common denominator is found, combine the numerators and adjust for any negative signs if subtracting fractions.
• 📝 In more complex fraction operations, like adding three fractions, you can use a larger common denominator if needed and simplify at the end.
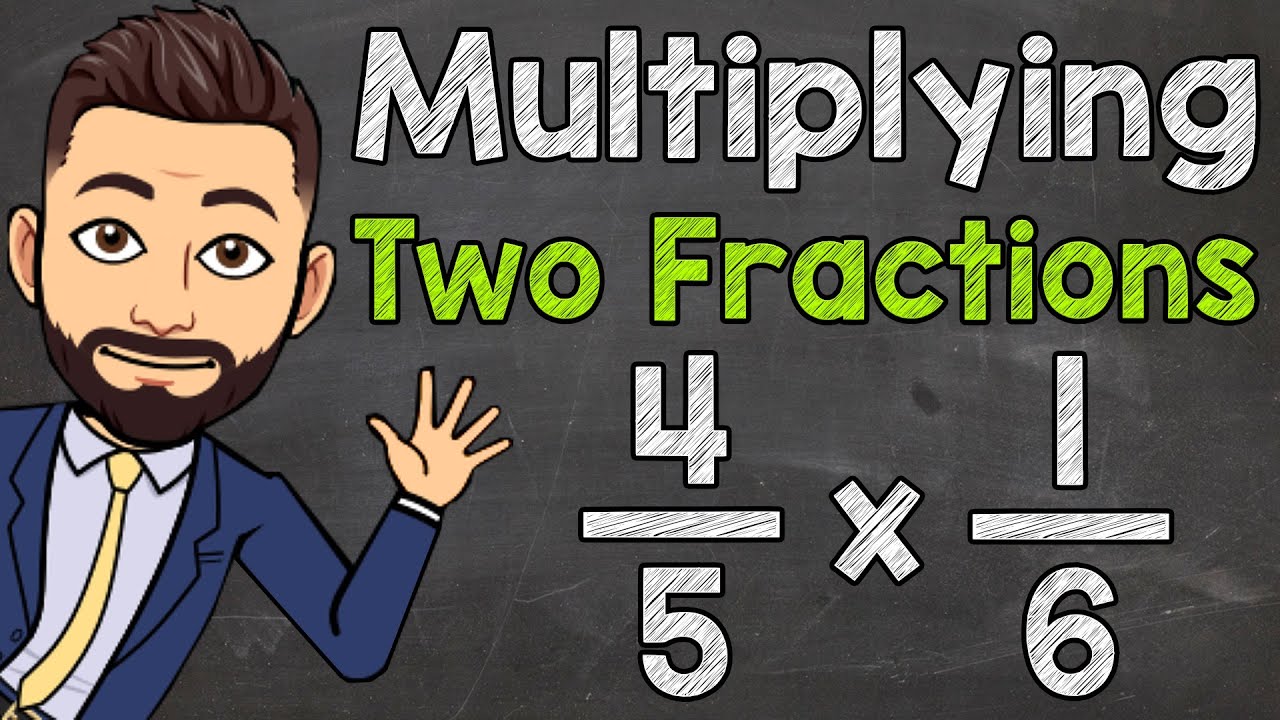
• 💡 Multiplying fractions involves multiplying the numerators and denominators directly, but you can simplify by breaking down larger numbers to avoid large products.
• 🧮 Simplifying before multiplying helps reduce the numbers, as shown when breaking 24/45 into smaller factors and canceling common terms.
• 🔄 Dividing fractions involves using the 'keep, change, flip' method: keep the first fraction, change the division to multiplication, and flip the second fraction before multiplying.

Q & A

What is the first step in adding or subtracting fractions?

The first step is to find a common denominator by multiplying the denominators of the fractions.

How do you add fractions like 3/5 + 4/7?

First, multiply the denominators (5 and 7) to get 35. Then, multiply 3 by 7 (21) and 5 by 4 (20). Finally, add the numerators to get 41, resulting in the fraction 41/35.

What should you do when subtracting fractions like 7/8 - 2/9?

Multiply the denominators (8 and 9) to get 72. Multiply 7 by 9 (63) and 8 by 2 (16), then subtract the numerators to get 47/72.

How can you find the least common denominator (LCD) when adding or subtracting fractions?

List the multiples of the denominators and find the smallest multiple they have in common. For example, the LCD of 2, 3, and 4 is 12.

What happens if you use a common denominator that is not the least common denominator?

You can still get the correct answer, but you will need to simplify the final result at the end.

In the example 3/4 + 5/3 - 7/2, why is 12 chosen as the common denominator?

12 is the least common denominator (LCD) because it is the smallest multiple common to 2, 3, and 4.

How do you add or subtract fractions with the same denominator?

Once the denominators are the same, combine the numerators. For example, in 9/12 + 20/12 - 42/12, add 9 and 20, then subtract 42 to get -13/12.

What is the process for multiplying two fractions?

Multiply the numerators and the denominators across the fractions. For example, 3/5 * 7/2 = 21/10.

Why is it helpful to break down large numbers when multiplying fractions?

Breaking down large numbers allows you to simplify the multiplication by canceling common factors, making the process easier and reducing the need for a calculator.

How do you divide fractions using the 'keep, change, flip' method?

Keep the first fraction, change the division to multiplication, and flip the second fraction. Then, multiply the fractions across.

Outlines

00:00

➕ Adding and Subtracting Fractions

This section introduces the concept of adding and subtracting fractions. It explains a method where you first multiply the denominators of the two fractions to get a common denominator. For example, in adding 3/5 and 4/7, you multiply the denominators 5 and 7 to get 35. Then, you multiply the numerators accordingly (3 x 7 = 21, 5 x 4 = 20), add the results (21 + 20), and place it over the common denominator to get 41/35. The same technique is applied to subtract fractions, such as 7/8 - 2/9. After getting a common denominator of 72, you subtract the numerators and arrive at the final simplified result.

05:01

➗ Adding or Subtracting Three Fractions

The video covers how to handle the addition and subtraction of three fractions. It introduces finding the least common denominator (LCD), and demonstrates how to do this by listing multiples of the denominators. For the example 3/4 + 5/3 - 7/2, the least common multiple of 4, 3, and 2 is 12. After converting all fractions to have this denominator, the numerators are manipulated (3/4 becomes 9/12, 5/3 becomes 20/12, 7/2 becomes 42/12). By adding and subtracting the numerators (9 + 20 - 42), you get the final result of -13/12.

10:04

💯 Adding Three Fractions with a Common Denominator

This section focuses on a more complex example of adding three fractions: 8/5 - 2/3 + 9/4. The common denominator is found by multiplying the denominators (5, 3, and 4), resulting in 60. Each fraction is then adjusted accordingly (8/5 becomes 96/60, 2/3 becomes 40/60, 9/4 becomes 135/60). The video explains how to subtract and add the numerators (96 - 40 + 135), arriving at a final answer of 191/60.

✖️ Multiplying Fractions

In this part, the process of multiplying fractions is demonstrated. The method is to simply multiply the numerators and the denominators across. For instance, multiplying 3/5 by 7/2 gives 21/10. The section also covers larger fractions like 24/45, and encourages breaking down larger numbers into smaller factors to simplify the multiplication, showing how factors can be canceled out before multiplying. For example, breaking down 24 as 6x4 and 45 as 9x5 allows for easier cancellations, leading to a simplified result of 4/3.

🧮 Multiplying Large Fractions by Simplification

This section explains how to multiply larger fractions using simplification techniques. The video gives an example of multiplying 56/77 by 35/40. By breaking down these numbers into their prime factors (e.g., 56 as 8x7, 77 as 11x7), the video shows how to cancel out common factors before multiplying the remaining numbers. This results in a simplified answer of 7/11.

➗ Dividing Fractions Using Keep-Change-Flip

This section introduces the 'Keep-Change-Flip' method for dividing fractions. It begins with an example of dividing 8/5 by 12/7. The first step is to keep the first fraction as it is, change the division sign to multiplication, and flip the second fraction (12/7 becomes 7/12). After simplifying common factors (such as canceling out a factor of 4), the final answer is 14/15. Another example is provided, 4/3 ÷ 9/5, demonstrating the same method, leading to a result of 20/27.

➕ Simplifying Complex Fraction Divisions

In this final section, a more complex fraction division is tackled: 36/54 ÷ 64/48. The video breaks down each number into its prime factors (e.g., 36 as 9x4, 54 as 9x6), then applies the keep-change-flip method. After simplifying by canceling common factors, the result is reduced to 1/2, demonstrating how larger fractions can be simplified step-by-step for an easier calculation.

Keywords

💡Fractions

A fraction represents a part of a whole and consists of a numerator (the top number) and a denominator (the bottom number). In the video, fractions are central to the lesson, as it focuses on adding, subtracting, multiplying, and dividing them. Examples include 3/5, 7/8, and more.

💡Common Denominator

The common denominator is a shared multiple of the denominators in different fractions, allowing them to be added or subtracted. The video explains how to find a common denominator, such as using 35 for 3/5 and 4/7, or 12 for 3/4, 5/3, and 7/2. This is essential for performing arithmetic operations on fractions.

💡Least Common Multiple (LCM)

The least common multiple (LCM) is the smallest number that is a multiple of two or more numbers. In the video, the LCM is used to find the least common denominator when adding or subtracting fractions. For example, the LCM of 2, 3, and 4 is found to be 12, allowing the fractions to be added.

💡Numerator

The numerator is the top number in a fraction, representing how many parts of the whole are being considered. The video explains how to multiply the numerators of fractions when adding or subtracting them, such as 3 and 7 in the example 3/5 + 4/7.

💡Denominator

The denominator is the bottom number of a fraction, showing how many equal parts the whole is divided into. The video frequently addresses how to find a common denominator to perform operations with fractions, such as multiplying 5 and 7 to get 35 for 3/5 and 4/7.

💡Multiplying Fractions

Multiplying fractions involves multiplying the numerators and denominators across the fractions. The video demonstrates this with the example of multiplying 3/5 by 7/2, where the numerators and denominators are multiplied to get 21/10.

💡Simplifying Fractions

Simplifying fractions involves reducing them to their simplest form by dividing both the numerator and denominator by their greatest common divisor. In the video, simplifying occurs after operations such as adding, subtracting, or multiplying, like simplifying 3/6 to 1/2.

💡Keep Change Flip

Keep Change Flip is a method used for dividing fractions. It involves keeping the first fraction, changing the division sign to multiplication, and flipping the second fraction (taking its reciprocal). The video uses this method when dividing 8/5 by 12/7, transforming it into a multiplication problem.

💡Reciprocal

A reciprocal of a fraction is obtained by swapping its numerator and denominator. The video explains that in division problems, such as dividing 8/5 by 12/7, you take the reciprocal of the second fraction (7/12) to convert the problem into a multiplication task.

💡Canceling Terms

Canceling terms refers to simplifying a multiplication problem by removing common factors in the numerator and denominator across the fractions. The video illustrates this with examples where numbers like 8 and 5 are canceled to simplify multiplication, such as in 56/77 multiplied by 35/40.

Highlights

Introduction to adding and subtracting fractions using common denominators.

Technique to find a common denominator by multiplying the denominators of two fractions.

Multiplying both numerators and denominators to add fractions with different denominators.

Example of subtracting two fractions by first finding the common denominator.

Steps to add or subtract three fractions by finding the least common denominator.

Using multiples of numbers to find the least common denominator for three fractions.

Simplification process: multiply fractions by necessary values to make denominators the same.

Adding numerators after converting fractions to the same denominator.

Solving an example with three fractions, finding a common denominator, and simplifying the result.

Introduction to multiplying fractions by multiplying across numerators and denominators.

Simplifying complex fractions by breaking larger numbers into factors before multiplying.

Cancellation of common factors before multiplying for easier fraction multiplication.

Using the 'keep, change, flip' method when dividing fractions.

Simplifying division problems by flipping the second fraction and converting the operation to multiplication.

Simplifying complex fraction division using factor cancellation and reduction to the simplest form.

Transcripts

in this lesson we're going to focus on

adding and subtracting fractions

let's say if we have 3 divided by 5

plus

4 divided by 7. how can we add these two

fractions

well here's a simple technique

first multiply five

and seven this will give you a common

denominator of thirty five

next

multiply

three and seven three times seven is

twenty-one

and also there's a plus in between

multiply

five times four which is twenty

twenty-one plus twenty

is forty-one so the answer is 41 divided

by 35.

let's try another example

let's say if we want to subtract 7 over

8

minus

2 over 9.

let's use the same technique

let's multiply the two denominators

eight and nine

which is uh 72

and then the next one

is going to be uh 7 times nine which is

sixty-three

minus

eight times two which is sixteen

now let's subtract what is sixty-three

minus sixteen

this is going to be 47.

now 47 is not divisible by 2

nor is it divisible by 3.

so this is it that's the final answer so

now you know how to add or subtract two

fractions

now what if we wanted to add or subtract

let's say three fractions instead of two

what should we do in this case

so let's say we wish to combine three

over four

plus

five over three

minus seven over two

whenever you wish to add or subtract

fractions

the denominator has to be the same the

denominator is the bottom part of the

fraction

and right now they're all different

so how can we make them the same how can

we get the common denominator

if you want to find the least common

denominator

make a list

all of the multiples of 2 are 2

4

6 8 10

12 14 and so forth

multiples of 3 are 3

6

9

12 15 18

and so forth and multiples of 4 are 4

8 12 16 20.

what is the least common multiple

we're looking for a multiple that is

common to all three numbers but is the

lowest

the least common multiple is 12.

12 is common to 2 3 and 4

and it's the lowest of such numbers

now granted

24 is also a common multiple

and if you use 24 you can get the right

answer you just got to simplify

at the end

so if you're ever like unsure about how

to find the least common denominator you

can find any common denominator one

simple technique is simply to multiply

these three

four times three times two is 24

and you could use 24 and still get the

right answer

so now that we know the least common

denominator is twelve

let's multiply each fraction in such a

way to get twelve

the first fraction

let's multiply the top and the bottom by

three because three times four is twelve

the second one let's use four

and for the last one let's use six

so looking at the first one three times

three is nine three times four is twelve

four times five is twenty four times

three is twelve

seven times six is forty two

two times six is twelve

now that we have the same denominator we

can combine the numerators

9 plus 20

is 29

and 29 minus 42

is negative 13. so this is the final

answer it's negative 13 divided by 12.

so now it's your turn go ahead and try

this example

8 over 5 minus 2 over 3

plus

9 divided by four

so go ahead and add these three

fractions

so this time

to find the least common multiple

we're just going to multiply five three

and four

it may not be the least common multiple

but it is a common denominator

just so you know

if we multiply 5 times 4 times 3

this will give us 5 times 4 is 20 20

times 3 is 16.

so 60 is going to be the common

denominator that we're going to try to

get

so we're going to multiply the first

fraction by 12

because 12 times 5 is 60

and the second one by 20

because 20 times 3 is 60. by the way if

you want to find out the number

divide it

60 divided by 5 will give you the 12.

60 divided by 3 will give you the 20

and 60 divided by 4

will give us the number that we need to

multiply this fraction by which is 15.

now 12 times 8 that's 96 5 times 12 we

know it's 60.

2 times 20 is 40 3 times 20 is 60

and 9 times 15

15 times 10 is 150

so if you take away 15 from that you'll

get 15 times 9 so that's 135

and 4 times 15 is 60.

now

96 minus 40

that's positive 56.

and 56 plus 135. let's go ahead

and add those two numbers the

old-fashioned way

five plus six is eleven carry over the

one one plus three plus five is nine

plus one

so the final answer is 191 divided by

sixty

in this lesson we're going to focus on

multiplying two fractions

whenever we need to multiply

multiply the numbers across the

fractions

3 times seven

is equal to twenty one

and five times two

is equal to ten

and so this is it

the answer is twenty 21 over 10. that's

all you need to do when multiplying

fractions

but sometimes the numbers may not be

that small

let's say if we have larger numbers

what should we do in this case

now we can multiply across we can

multiply 24 and 45

which will give us a big number

but do we really want to do that

when multiplying fractions with large

numbers

it's in your best interest

to break down the large numbers into

small numbers for instance

24

is basically six times four

27 is nine times three

forty-five is nine times five

and 30 is 6 times 5.

you want to break it in such a way that

you can cancel

some numbers here we can cancel a 5

because we have 1 on top and the other

on the bottom the same is true for the 9

and we can cancel a six

so therefore the final answer

is four over three

so we were able to get the final answer

without multiplying twenty four by forty

five

that step was necessary plus is going to

take some time and you need a calculator

doing it this way requires no use of a

calculator

try this one

multiply 56

divided by 77

by 35 over 40.

now 56 is 8 times 7

77 is 11 times 7

35 is 7 times 5

and 40 is 8 times 5.

so we can cancel an eight

we can cancel a seven

and we can cancel a five

leaving the final answer of seven over

eleven so now you know how to multiply

two fractions

in this lesson we're going to focus on

dividing two fractions

let's use eight over five as an example

and let's divide it by

twelve over seven

now perhaps you heard of the expression

keep change flip

it's useful when dividing fractions

keep the first fraction the same way

change division to multiplication and

flip the second fraction and now you can

do it

so 8 times 7

is 56 but we can simplify it before we

multiply

8 is basically 4 times 2

and

12 is four times three

so we could cancel a four

and now we can multiply

two times seven

is fourteen

and five times three is fifteen

so the final answer is fourteen over

fifteen

try this one what's four divided by

three

divided by

nine over five

so using the expression keep change flip

let's keep the first fraction the same

let's change division to multiplication

and let's flip the second fraction

now there's nothing to cancel so let's

multiply across 4 times 5 is 20

3 times 9 is 27

and so we can't reduce this fraction

that's the answer

now what if you see

a problem that looks like this

36 over 54

divided by

64 over 48

if you have a fraction written this way

what should you do

this expression is equivalent to saying

36 over 54

divided by 64 over 48

and then we can use the

keep

change flip principle let's keep the

first fraction the same

let's change division to multiplication

and then

let's flip the second fraction

and now let's simplify

so 36

is basically

nine times

four

fifty four

is nine times six

forty eight is sixteen times three

and sixty four

is sixteen times four

so right now we can cancel a nine

we can cancel a 16

and we can cancel a four

so what we have left over is three over

six

now three over six can be reduced

we can divide both numbers

by three

three divided by three

is equal to one six divided by three is

two

so the final answer is one over two

you