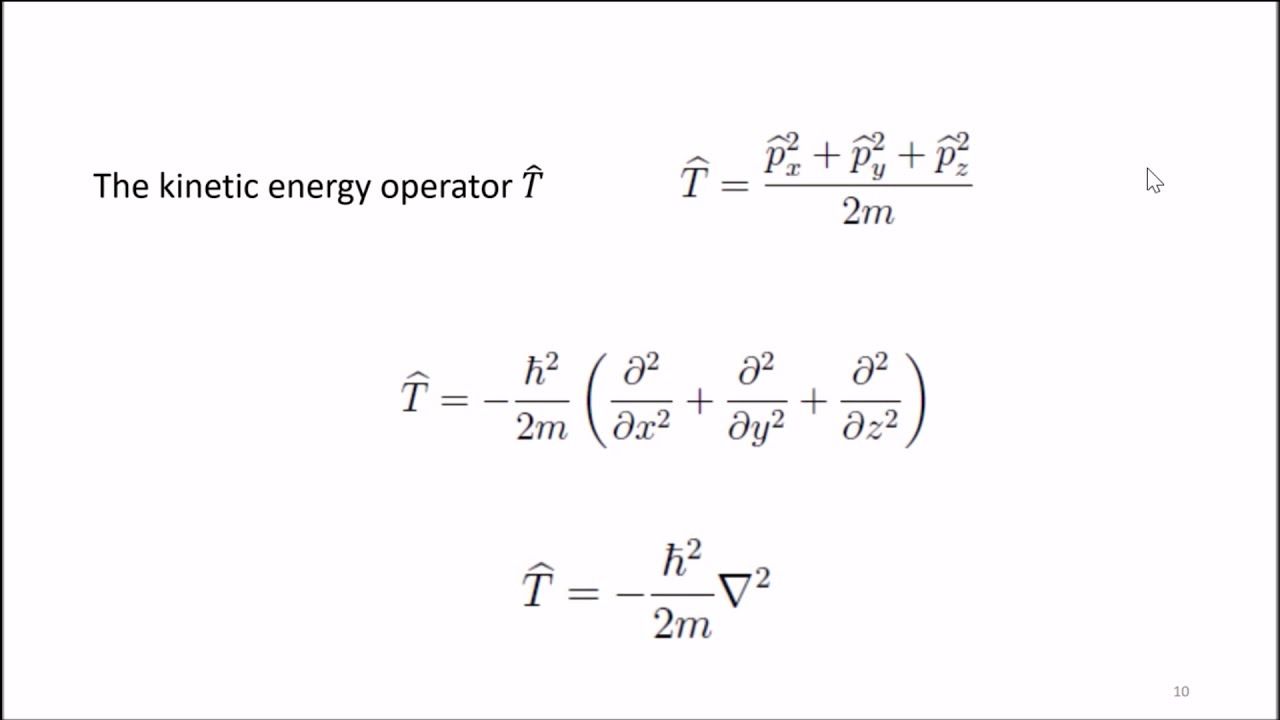
Wave functions in quantum mechanics

Summary

This educational video delves into the concept of wave functions in quantum mechanics, explaining their significance and connection to the position and momentum operators. It covers the derivation of wave functions, their role in the position and momentum representations, and the scalar product between states. The video also explores the transformation between position and momentum space through Fourier transforms, providing a foundational understanding of wave mechanics and its applications in 3D spatial dimensions.

Takeaways

• 🌊 Wave functions are a key concept in quantum mechanics, representing the position representation of quantum systems.
• 🎓 Students often first encounter quantum mechanics through wave functions, which are integral to understanding 3D spatial systems like potential barriers and the hydrogen atom.
• 📚 Wave functions are derived from the eigenvectors of the position operator, forming an orthonormal basis for state space.
• 🧮 The wave function \( \Psi(x) \) is defined as the expansion coefficient of a state vector in the position basis, essential for studying quantum systems.
• 🔄 The script explains the transition from position to momentum representation, highlighting the use of Fourier transforms in quantum mechanics.
• 🔢 The scalar product between two states in position representation is derived, emphasizing the foundational role of wave functions in quantum mechanics.
• 📉 The normalization of a wave function is discussed, showing how it relates to the probability interpretation of quantum mechanics.
• 🔄 The overlap matrix between position and momentum representations is explored, leading to the derivation of the transformation matrix.
• 🔗 The script connects the position and momentum wave functions through the Fourier transform, a fundamental result in wave mechanics.
• 📚 The generalization to three dimensions is briefly touched upon, showing how the principles apply to vector operators in quantum mechanics.

Q & A

What is a wave function in quantum mechanics?

A wave function in quantum mechanics is a mathematical description of the quantum state of a particle or system of particles. It is used to determine the probability distribution of a particle's position, momentum, and other properties.

Why are wave functions important in quantum mechanics?

Wave functions are important because they provide a way to calculate the probability of finding a particle in a particular location or state. They are fundamental to understanding quantum phenomena and are used in many quantum mechanical calculations.

What is the position representation in quantum mechanics?

The position representation is a way of expressing quantum states using wave functions that describe the probability distribution of a particle's position in space. It is one of the possible representations in quantum mechanics, leading to the formulation known as wave mechanics.

How are wave functions related to the position and momentum operators?

Wave functions are related to the position and momentum operators through eigenvalue equations. The position operator acting on a wave function gives the eigenvalue corresponding to a specific position, and similarly, the momentum operator acting on a wave function gives the eigenvalue corresponding to a specific momentum.

What is the significance of the commutator between position and momentum operators?

The commutator between the position (X) and momentum (P) operators, given by [X, P] = iħ, is significant because it represents the fundamental uncertainty principle in quantum mechanics. It shows that position and momentum cannot be precisely known at the same time.

How do you derive the scalar product between two wave functions?

The scalar product between two wave functions can be derived by expanding the state vectors in the position basis and using the orthonormality of the position eigenstates. The result is an integral over space of the product of one wave function and the complex conjugate of the other.

What is the normalization of a wave function and why is it important?

The normalization of a wave function is the process of ensuring that the integral of the absolute square of the wave function over all space is equal to 1. It is important because it ensures that the probabilities derived from the wave function sum to 1, which is a requirement for a valid probability distribution.

How can one transform from the position representation to the momentum representation?

One can transform from the position representation to the momentum representation using the overlap matrix, which is the integral of the position eigenstate times the momentum eigenstate. This leads to a Fourier transform relationship between the position space wave function and the momentum space wave function.

What is the role of the translation operator in quantum mechanics?

The translation operator in quantum mechanics is used to move a quantum state by a certain amount in space. It is used in the calculation of the overlap matrix between position and momentum eigenstates and is crucial for deriving the relationship between position and momentum representations.

How do wave functions help in understanding 3D quantum systems?

Wave functions help in understanding 3D quantum systems by providing a framework to describe the quantum states in three-dimensional space. They allow for the calculation of probabilities and the study of phenomena such as quantum tunneling and the behavior of particles in potential wells or barriers.

Outlines

00:00

🌌 Introduction to Quantum Mechanics and Wave Functions

The video begins with an introduction to quantum mechanics, focusing on wave functions. It explains that wave functions are a way to view quantum systems, particularly in the context of 3D spatial dimensions. The paragraph highlights the importance of wave functions in studying quantum mechanics due to their relevance in understanding systems like potential barriers, potential wells, and the hydrogen atom. The narrator outlines the plan for the video, which includes learning about wave functions as the position representation of state vectors, deriving known results from wave mechanics, and relating wave functions in real space to those in momentum space. The discussion starts with the position operator and momentum operator, their commutator, and how wave functions are related to these operators and their eigenstates.

05:02

📚 Deriving Scalar Product and Normalization from Wave Functions

This paragraph delves into the mathematical aspects of wave functions, starting with the eigenvalue equation for the position operator. It discusses the hermitian nature of the position operator and its orthonormal eigenstates. The concept of expanding any state vector in terms of this basis is introduced, leading to the definition of wave functions as the expansion coefficients in the position basis. The paragraph then moves on to discuss the scalar product between two states in the position representation, deriving the familiar expression from quantum mechanics. It also covers the normalization of a wave function, presenting the integral form that equates to the absolute square of the wave function.

10:02

🔄 Transition from Position to Momentum Representation

The focus shifts to transitioning from the position representation to the momentum representation. The paragraph introduces the overlap matrix, which is the bracket between position and momentum eigenstates. It encourages viewers to review the translation operator and its properties before proceeding. The translation operator's action on position eigenstates is discussed, leading to the calculation of the overlap matrix. The paragraph concludes with the derivation of a first-order differential equation for the bracket between position and momentum, setting the stage for solving it in the next part of the video.

15:03

🌐 Generalizing to Three Dimensions and Fourier Transforms

The final paragraph generalizes the concepts from one dimension to three dimensions, introducing the vector operators for position and momentum. It discusses the canonical commutation relations and how they relate to the eigenvalue equations for these operators. The paragraph also covers the orthonormality of the eigenstates and how an arbitrary state can be expanded in terms of these basis states. The video concludes with the derivation of the Fourier transform relationship between position and momentum wave functions, highlighting the integral involving a plane wave that connects these representations. The narrator summarizes the key takeaways from the video, emphasizing the foundational role of wave functions in quantum mechanics and their utility in studying 3D spatial problems.

Keywords

💡Wave Function

A wave function in quantum mechanics is a mathematical description of the quantum state of a particle or system of particles. It is used to determine the probabilities of the possible results of measurements, such as position, momentum, and energy. In the video, wave functions are introduced as a fundamental concept in quantum mechanics, particularly important for understanding quantum systems in 3D spatial dimensions. The wave function is defined as the expansion coefficient of a state vector in the position basis, and it is central to the formulation known as wave mechanics.

💡Position Representation

Position representation refers to a way of expressing quantum states in terms of position eigenstates. It is one of the possible representations in quantum mechanics, where the wave function is used to describe the state of a system. The video explains that wave functions are the position representation of state vectors, which means they describe the quantum state in terms of position eigenstates. This is crucial for understanding how quantum systems behave in space.

💡Momentum Representation

Momentum representation is another way of expressing quantum states, this time in terms of momentum eigenstates. It is complementary to the position representation and is useful for describing the state of a system in terms of its momentum. The video discusses how to relate wave functions in real space to wave functions in momentum space, which is essential for understanding the behavior of quantum systems in different contexts.

💡Eigenvalue Equation

The eigenvalue equation is a fundamental equation in quantum mechanics that relates an operator to its corresponding eigenvalue and eigenstate. In the context of the video, the eigenvalue equation for the position operator is discussed, which states that when the position operator acts on a position eigenstate, it yields the position eigenvalue. This equation is essential for understanding how wave functions are related to the position and momentum operators.

💡Hermitian Operator

A Hermitian operator is an operator that is equal to its adjoint, meaning its eigenvalues are real and its eigenstates form a complete orthonormal set. In the video, the position operator is described as a Hermitian operator, which allows for the formation of an orthonormal basis. This property is crucial for expanding any state vector in terms of this basis, which is a key step in defining wave functions.

💡Scalar Product

The scalar product, also known as the inner product, is a mathematical operation that takes two vectors and returns a scalar. In quantum mechanics, the scalar product between two wave functions is used to calculate the probability amplitudes. The video derives the scalar product between wave functions from the more general idea of scalar products between state vectors, which is a key concept in understanding quantum states.

💡Normalization

Normalization in quantum mechanics refers to the process of scaling a wave function so that its integral over all space equals one. This ensures that the probabilities of all possible outcomes of a measurement add up to one, which is a requirement in probability theory. The video explains how to normalize a wave function by integrating the absolute value squared of the wave function over all space.

💡Fourier Transform

A Fourier transform is a mathematical technique that transforms a function from one domain (like time or space) to another (like frequency). In the video, Fourier transforms are used to relate the position representation to the momentum representation. This is done by calculating the corresponding Fourier transforms, which is a general result familiar to those who know about wave mechanics.

💡Translation Operator

The translation operator in quantum mechanics is an operator that moves a quantum state by a certain amount in space. The video discusses the translation operator in the context of calculating the overlap matrix between position and momentum representations. The translation operator is used to derive the relationship between position and momentum wave functions, which is essential for understanding how quantum systems behave under spatial translations.

💡Commutator

In quantum mechanics, a commutator is an operator that measures the extent to which two observables (like position and momentum) do not commute, meaning the order in which they are measured affects the result. The video mentions the commutator of the position and momentum operators, which is a fundamental aspect of quantum mechanics. The non-zero commutator implies that position and momentum are conjugate variables, a key concept in understanding the uncertainty principle.

Highlights

Wave functions are a representation of quantum systems in the position basis.

Wave functions are fundamental in quantum mechanics and lead to wave mechanics.

Students often first encounter quantum mechanics through wave functions.

Wave functions are particularly useful for studying 3D systems like potential barriers and the hydrogen atom.

Wave functions are the expansion coefficients of state vectors in the position basis.

Position and momentum operators, X and P, are key to understanding wave functions.

Eigen kets of the position operator form an orthonormal basis for state space.

Any state vector can be expanded in the position basis using integration over position eigen kets.

The wave function in momentum space is defined similarly to the position space wave function.

Eigen kets of the momentum operator also form an orthonormal basis.

The scalar product between two states in position space is derived from the wave functions.

Normalization of a wave function is integral over position space of the absolute value squared of the wave function.

The transformation from position to momentum representation involves the overlap matrix.

The translation operator plays a crucial role in calculating the overlap matrix.

The overlap matrix between position and momentum eigen kets is proportional to e to the IPX over H bar.

The proportionality constant for the overlap matrix is determined to be 1 over the square root of 2 pi H bar.

Fourier transforms are used to relate wave functions in position and momentum representations.

Wave functions are a special case of representation in quantum mechanics, derived from state vectors and state space.

The video concludes with a generalization of the results to three dimensions, involving vector operators R and P.

Transcripts

hi everyone there is Professor am the

science many people who have only heard

about quantum mechanics in passing have

nonetheless heard about wave functions

but what is a wave function a wave

function is only one of many possible

ways of looking at a quantum system is

the so called position representation of

quantum mechanics and it leads to the

famous wave mechanics so why are wave

functions famous well there are two main

reasons why wave functions are famous

the first one is because many students

first learn about quantum mechanics in

terms of wave functions the second one

is because the language of wave

functions is most useful when studying

systems in 3d spatial dimensions these

include famous examples suggest

potential barriers potential wells all

the way to the hydrogen atom in this

video we will first of all learn that

wave functions are the position

representation of state vectors second

we will derive some known results from

wave mechanics such as the scalar

product between wave functions and third

will relate wave functions in real space

to wave functions in momentum space

let's get started to study wave

functions we need to start by looking at

the position operator X and the momentum

operator P and their commutator IH bar

this is because wave functions are

intimately related with these two

operators and their eigen ket's so let's

start by looking at the eigenvalue

equation for the position operator X hat

acting on the cat X gives us the

eigenvalue x acting on the cat x and to

be clear what I mean here by X hat is

the position operator by X I'm in the

position eigen value which is a quantity

that has unit of length and what I mean

by the cat X is the ket associated with

the eigenvalue x the position operator

is a hermitian operator and therefore

its eigen ket's form a basis that we can

choose to be orthonormal what that means

is that we can write the bracket between

X and X prime as equal to the Delta

function at X minus X prime once we have

defined the basis we can expand any ket

in our state space in terms of this

basis so let's do that let's pick a cat

sy and we expand it in the position

basis that we write it as an integral

over DX the expansion coefficient is the

bracket between X and soy and then

each of these is multiplied by the basis

cat X we are now ready to make one of

the most important definitions in the

whole of quantum mechanics we define the

wave function Phi of X as the expansion

coefficients of a cat side in the basis

formed by the eigen ket's of the

position operator wave functions are

fundamental in our study of quantum

mechanics and they form the formulation

known as wave mechanics my guess is that

most of you will have first learned

about quantum mechanics in terms of wave

functions what we can say here is that

wave functions are nothing more than a

special case of a representation they

are the representation of a state vector

psi corresponding to the eigen ket's of

the position operator so now we're able

to see how wave functions fit in the

more fundamental formulation of quantum

mechanics in terms of state vectors and

state space we can do something very

similar to what we have done for the

position representation in terms of the

momentum representation so we can look

at the eigenvalue equation for the

momentum operator P hat acting on the

ket P is equal to the eigenvalue p

acting on the ket P as before P hat

means the momentum operator P means the

momentum eigen value which has units of

mass times velocity and the ket P is the

ket associated with the eigen value P

the eigen ket's of P also form a basis

whose orthonormality condition is such

that the bracket between p and p prime

is equal to the Delta function of p

minus p prime we can expand an arbitrary

cat's-eye in the Pearson tation as an

integral over DP of the expansion

coefficient which is the bracket of p

with side times the basis ket P and we

can now define the wave function in

momentum space as sy bar of P as equal

to the expansion coefficient that is the

projection of the cat's-eye on the basis

P what we have learned so far in this

video are some of the most important

ideas in the whole of quantum mechanics

so do make sure that you understand

everything that we have discussed to

recap we have introduced the idea of

wave functions the wave function in the

position representation sigh of X is the

projection of a state vector psi onto

the position representation and the wave

function in the

mentum representation sidebar of P is

similarly the projection of a cat's eye

onto the basis P now that we have

introduced wave functions we are ready

to look at a number of operations in

state space in terms of wave functions

and to do that we will use the position

representation wave function Phi of X

the first property I want to discuss is

the scalar product between two states so

let's consider a first state psi in the

position representation integral over

the X bracket of X with side X and the

second state Phi in the position

representation as well integral over DX

prime bracket of X prime with Phi X

prime we can now calculate the scalar

product between these two states as the

bracket between sy and Phi and plugging

in the expansions in the position

representation we obtain integral over

DX sy X X integral over the X prime X

prime Phi X Prime rearranging this

expression we get integral with the ex

integral over the X Prime sy X X prime

Phi xx prime we recognize sy X as the

definition of the wave function star of

X X prime Phi as the wave function Phi

of X and xx prime as the Delta function

X minus X prime the Delta function makes

the integral of a DX prime very easy and

therefore we can write the scalar

product between psi and Phi as equal to

the integral over DX of Phi star X Phi X

those of you familiar with wave

mechanics will immediately recognize

this expression as the usual scalar

product between two wave functions but I

want to emphasize that in our case we

have derived it from more general ideas

based on scalar products between cats in

state space an easy next step we can

take now is to look at the normalization

of a wave function to do that we can see

that the norm of a cat which is the

scalar product of a cat sy with itself

and by using the formula we have just

the right we can write this down as the

integral over DX of side Star X Phi of X

and then we can rewrite this as the

integral of a DX of the absolute value

squared of Phi of X again this should be

formula very

Mille to those of you who know wave

mechanics so far we have looked at wave

functions both in the position and

momentum representations something that

is very useful when we do quantum

mechanics is to be able to go from one

representation to another so what I want

to do next is to look at how we can go

from the position representation to the

momentum representation to do that we

need to consider the overlap matrix

which in this case is the bracket

between X and P and if you need a

refresher about transforming from one

basis to another and about overlap

matrices take a look at the video linked

in the description before we try to

figure out what the overlap between X

and P is a word of encouragement it is a

bit tricky to get there but we will get

there so do bear with me

the first step we need to take is to

refresh our minds about the translation

operator there is a link to a full video

about the translation operator in the

description but let me just quote some

of the most important results that we

need for calculating the overlap matrix

the translation of a position eigen ket

by an amount alpha is given by a

translation operator T alpha which is

equal to e to the minus I alpha P over H

bar we can consider an infinitesimal

translation of minus epsilon to get T of

minus epsilon equals e to the I epsilon

P over H bar and we can Taylor expand

this exponential to obtain 1 plus I

Epsilon over H bar P plus a term of

order epsilon squared the action of the

translation operator T alpha on a ket X

is equal to another ket X plus alpha and

the corresponding expression in dual

space is such that the bra x times the

operator t alpha is equal to the bra X

minus alpha again if these results do

come at a surprise check the video link

in the description for more details now

that we have refreshed our mind by the

translation operator the first step we

need to take to calculate the overlap

between X and P is to look at the matrix

element of an infinitesimal translation

with respect to X and P so let's write X

T of minus epsilon P we then use the

results that we obtained here for the

dual space action of the translation

operator to rewrite this down as X plus

epsilon P we now copy the same matrix

element

x t- epsilon P but use instead the other

representation above in terms of the

Taylor expansion of the translation

operator to write X 1 plus I epsilon

over h-bar P plus a term of order

epsilon squared P putting this together

we obtain XP plus I epsilon over h-bar X

P hat P plus a term of order epsilon

squared one thing to note is that P hat

P is simply the eigen value P times the

ket P now that we have written down

these two expressions for the matrix

element of an infinitesimal translation

we are ready to set them equal to each

other and we can therefore rearrange

that equation to obtain eigen value P

bracket of X and P equals minus IH bar

and then we take the limit of epsilon

going to 0 of the rest of the terms that

depend on epsilon so we get the bracket

of X plus epsilon P minus the bracket of

X with P over epsilon the limit we have

written down is the definition of a

derivative and therefore we can write

this whole expression as equal to minus

IH bar the derivative with respect to X

of the bracket of X and P looking at the

left and at the right hand side of this

equation we see that we now have a first

order differential equation for the

bracket between X and P that we need in

our quest to transform from the position

to the momentum representations we can

solve this first order differential

equation by separation of variables so

we write d bracket of X with P over

bracket of X with P equals I over H bar

P DX we integrate both sides to obtain

the logarithm of the bracket between X

and P equals I over H power P X plus the

integration constant C and then we can

exponentiate both sides of the equation

to obtain that the bracket between X and

P is equal to n e to the IPX over H bar

the N in this expression is simply the

transformation of the integration

constant C where we exponentiated both

sides of the equation after quite a few

steps we have finally reached the

conclusion that the overlap matrix

between X and P is proportional to e to

the IPX over H bar and all we have left

to do now is to determine the

proportionality constant and let's start

with a fresh page

and we write down the final result we

obtained which is that the bracket

between X and P is equal to n times e to

the IPX over H bar to find the constant

n we'll start by looking at the

orthonormality condition in the X

representation the bracket of X with X

prime is equal to the Delta function of

X minus X prime and then we're going to

rewrite this bracket in the following

manner X 1 X prime equals x now we

insert the resolution of the identity in

the p representation so we write

integral of the DP of PP x prime and

operating through this gives us the

integral over DP of X P px prime the two

terms under the integral sign are simply

equal to the term above and it's complex

conjugate and we can therefore write the

whole thing as equal to absolute value

square root of n integral over DP of e

to the IPX minus X prime over H bar at

this point we must use one of the many

definitions of the Delta function which

tells us a delta function of X minus X

prime is equal to 1 over 2 pi integral

over D U of e to the IU X minus X Prime

and looking at our expression we see

that we have exactly this under the

integral sign if we make the

substitution u equals P over H bar we

can therefore evaluate the integral we

have to obtain M Squared 2 pi H bar

Delta function of X minus X Prime this

term we just obtained must be equal to

the Delta function above because both of

them are equal to the bracket between X

and X Prime we can therefore set them

equal to each other

and we obtain that the absolute value

squared of n is equal to 1 over 2 pi H

bar which in turn tells us that n is

equal to 1 over square root of 2 pi H

bar putting everything together we

finally can write the expression for the

overlap matrix between X and P which is

equal to 1 over square root of 2 pi H

bar times e to the IPX over H bar okay

so as I said at the beginning it would

take us a while to get here but finally

we have determined the transformation

matrix XP that allows us to go between

the position representation and the

momentum represented

so let's finish the job and let's write

sidebar of P equals the projection of

sion P which is equal to p1 sy which is

equal to P then we insert the resolution

of the identity in terms of X which is

integral of DX X X sine and then we

reexpress this whole thing as integral

over DX px X sine we can now collect the

fruit of our labor and we identify the

PX term as simply the conjugate of the

XP term above we also identify exercise

as simply the wave function Phi of X and

we can therefore write the whole thing

as 1 over square root of 2 pi H bar

integral over the X e to the minus IPX

over H bar Phi of X you should repeat

the same exercise for the converse

transformation but I'm just going to

write down the result which is that the

wave function of Phi of X which is equal

to the bracket between X and PSI is

equal to 1 over square root of 2 pi H

bar integral over DP of e to the IPX

over H bar sy bar P we are finally done

and we can say now that in order to go

from the wave function in the position

representation to the wave function in

the momentum representation or vice

versa

all we have to do is we have to

calculate the corresponding Fourier

transforms this is another general

result that will be familiar to those of

you who know about wave mechanics and

again we have reached this result by

using the more fundamental formalism of

state space and state vectors before we

conclude I very quickly want to

generalize the result we have obtained

in one I mentioned in terms of X and P

to three dimensions in terms of the

vectors R and P in three dimensions the

position operator R is a vector operator

made of three components which are X 1 X

2 and X 3 or equivalently X Y Z

similarly the momentum operator is also

a vector operator P and again it has

three components P 1 P 2 and P 3 or also

px py PZ putting these together we find

the canonical commutation relations

between these vector operators as

xj p k equals IH bar delta JK we can now

start looking at all the results we have

the right thing one i mentioned so the

eigenvalue equation for the position

operator is the operator are acting on

the ket R which gives you the eigen

value are acting on the cat are the

eigen ket's form a basis which is

orthonormal so the bracket between r and

r prime is equal to the Delta function

of R minus R prime and we can expand an

arbitrary cat's-eye in terms of the

position basis as the integral over the

r sy of our our website of r is the 3

dimensional wave function which is given

by the projection of the state psi on

the basis function r it works in exactly

the same way for the momentum operator

so p hat

acting on the ket P is equal to the

eigen value P acting on the ket P the

orthonormality relation reads bracket PP

prime equals Delta function of P minus P

prime we can expand the state sy in the

P basis as integral over DP sidebar of P

P and sy bar of T is the 3-dimensional

momentum wave function which is given by

the bracket between P and sy just like

in one dimension we can relate the

position and momentum wave functions

through the Fourier transform sy of R is

equal to 1 over 2 pi H bar to the power

of 3 hulls integral over DP e to the I P

dot R over H bar sidebar of P so let's

recap what we have accomplished in this

video we have started by looking at the

position operator X and the momentum

operator P whose commutator is IH bar

and we have defined representations

associated with the eigen ket's X and P

of these two operators these two

representations are very important in

quantum mechanics because they lead to

the formulation known as wave mechanics

which is the formulation by which most

students are first introduced to the

quantum world what we have seen is how

this formulation fits into the more

fundamental formulation based on state

space and we have identified the wave

function Phi of X as the representation

of a state side in the X faces

and the momentum space wave function

sidebar of P as the representation of

state sigh in the momentum basis having

defined wave functions from the

fundamental state space formulation of

quantum mechanics we can then derive the

usual results from wave mechanics such

as the scalar product between two states

PI and Phi which is the integral of a DX

of size star of X Phi of X and the

normalization of a cat

sy sy which is equal to the integral

over the X of the absolute value squared

of psi of X we have also been able to

relate the position representation to

the momentum representation and we have

found that the wave function psi of X is

related to the momentum space wave

function cipher of P through an integral

over a plane wave e to the IPX over H

bar which tells us that the momentum and

position wave functions are related to

each other via Fourier transform

in this video we have learned that wave

functions are simply a particular

representation of state vectors and we

have seen how they fit in the why the

formalism of quantum mechanics so what

next wave functions are extremely useful

in studying problems in 3d spatial

dimensions so we can now learn about

things like quantum tunneling of

particles all the way to the hydrogen

atom if you liked this video or if you

like to send me suggestions for future

videos subscribe