Circle Theorems IGCSE Maths

Summary

This educational session delves into the properties of a circle, defining it as a set of points equidistant from a central point. Key concepts covered include the radius, circumference, diameter, and the relationships between them. The session also explores tangents, secants, and chords, emphasizing the perpendicularity of the radius to the tangent and the bisector property of chords. It discusses angles in a semicircle being 90° and the relationship between angles at the center and on the circumference. The video concludes with insights on cyclic quadrilaterals, where opposite angles are supplementary, and the sum of all angles equals 360°.

Takeaways

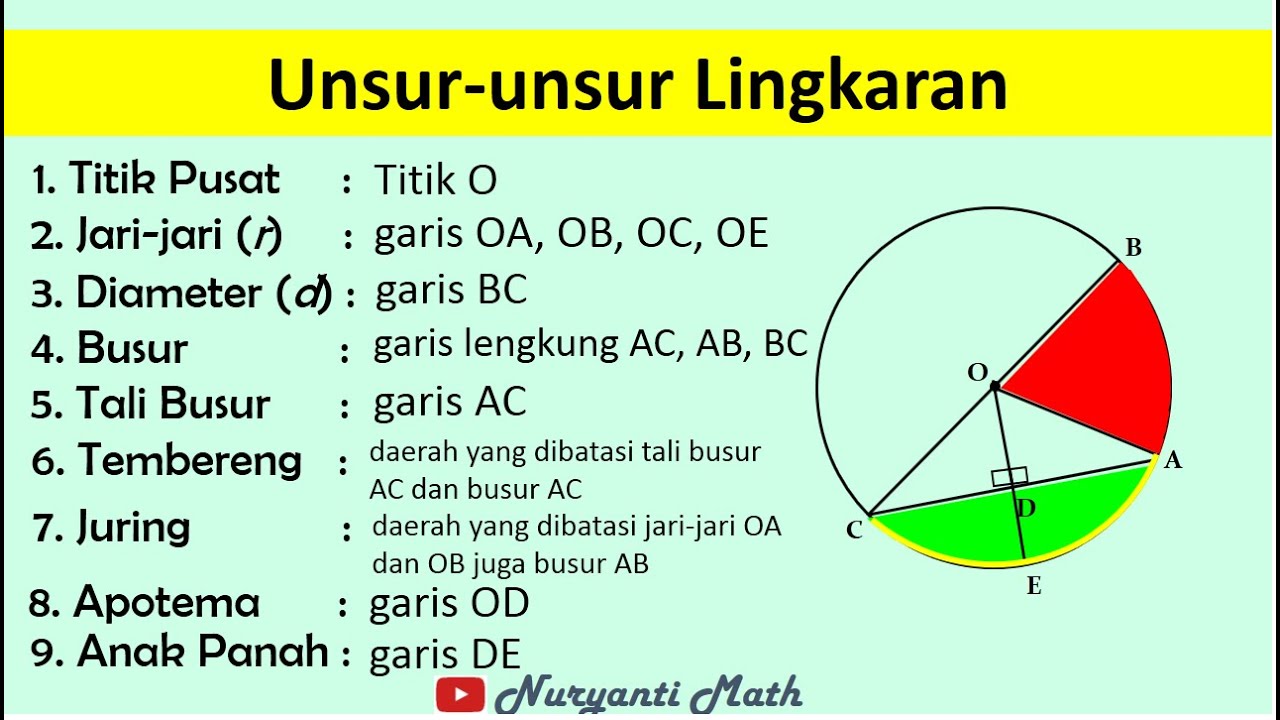
• 🔵 A circle is defined as a set of points equidistant from a fixed point known as the center.
• 📏 The radius of a circle is the distance from the center to any point on the circumference.
• 🌀 The circumference of a circle is calculated as \( 2\pi r \), where \( r \) is the radius.
• 📏 The diameter of a circle is the longest chord that divides the circle into two equal parts, and it is equal to twice the radius (\( D = 2R \)).
• 📐 A tangent to a circle is a line that touches the circle at exactly one point.
• 📐 A secant is a line that intersects a circle at two points, extending beyond the circle.
• ✂️ The property of equal chords in a circle states that if two chords are equal, their distances to the center are also equal.
• 🔼 The angle in a semicircle is always 90 degrees, reflecting the right angle property of a semicircle.
• 🔼 The angle between a tangent and a radius is always 90 degrees, indicating the perpendicular relationship.
• 🔄 The angle at the center of a circle subtended by an arc is twice the size of the angle on the circumference subtended by the same arc.

Q & A

What is the definition of a circle?

A circle is the set of all points that are equidistant from a fixed point, known as the center.

What is the term for the distance from the center of a circle to any point on its circumference?

The distance from the center of a circle to any point on its circumference is called the radius.

How is the circumference of a circle calculated?

The circumference of a circle is calculated using the formula C = 2πr, where r is the radius of the circle.

What is the term for a line that divides a circle into two equal parts?

A line that divides a circle into two equal parts is called the diameter, which is also the longest chord in a circle.

What is the relationship between the diameter and the radius of a circle?

The diameter of a circle is twice the length of its radius, expressed as D = 2R.

What is a tangent in the context of a circle?

A tangent is a line that touches the circle at exactly one point.

What is a secant in relation to a circle?

A secant is a line that intersects the circle at two points, extending beyond the circle.

What property ensures that a line drawn from the center of a circle to the midpoint of a chord is perpendicular to the chord?

The property that ensures a line from the center to the midpoint of a chord is perpendicular is known as the perpendicular bisector theorem.

How are the angles in a semicircle related?

Any angle inscribed in a semicircle is always a right angle, meaning it measures 90 degrees.

What is the relationship between the angle at the center of a circle and the angle on the circumference subtended by the same arc?

The angle at the center of a circle subtended by an arc is twice the size of the angle on the circumference subtended by the same arc.

What is a cyclic quadrilateral, and what is one of its properties?

A cyclic quadrilateral is a quadrilateral whose vertices all lie on a circle. One of its properties is that the sum of the opposite angles is supplementary, meaning they add up to 180 degrees.

Outlines

00:00

🌀 Introduction to Circles and Their Properties

This paragraph introduces the concept of a circle and its fundamental properties. A circle is defined as a set of points equidistant from a fixed point known as the center. The distance from the center to any point on the circle is called the radius. The circumference, or perimeter, of a circle is calculated as 2πr, where r is the radius. The paragraph also explains the terms cord and diameter, with the former being any line segment connecting two points on the circle and the latter being the longest possible cord, which passes through the center and divides the circle into two equal halves. The relationship between diameter (D) and radius (r) is given as D = 2r. The properties of tangents and secants are also discussed, with tangents being lines that touch the circle at exactly one point, and secants being lines that intersect the circle at two points. The paragraph concludes with a discussion on the properties of equal chords and the perpendicular bisector of a sector, stating that a line drawn from the center to the midpoint of a chord is perpendicular to the chord, and chords of equal length are equidistant from the center.

05:01

📐 Exploring Tangents, Angles, and Cyclic Quadrilaterals

The second paragraph delves deeper into the properties of tangents and angles within a circle. It explains that the length of a tangent from an external point to the circle is constant, leading to the conclusion that two triangles formed by a tangent and a radius are congruent. This congruence implies that angles subtended by the same arc are equal. The paragraph also discusses the angle in a semicircle, which is always 90 degrees, and uses this property to solve for angles in various geometric configurations. The concept of an external angle being equal to the sum of the two non-adjacent interior angles is also explored. The paragraph concludes with an explanation of the properties of angles at the center and on the circumference of a circle, stating that the angle at the center is twice that of the angle on the circumference subtended by the same arc. This knowledge is applied to solve problems involving angles in cyclic quadrilaterals, where the sum of opposite angles is supplementary.

10:01

🔄 Understanding Cyclic Quadrilaterals and Angle Properties

The final paragraph focuses on cyclic quadrilaterals and their properties. A cyclic quadrilateral is defined as a quadrilateral whose vertices all lie on a circle. The paragraph discusses two key properties of cyclic quadrilaterals: the sum of all interior angles equals 360 degrees, and the sum of opposite angles is supplementary (i.e., they add up to 180 degrees). Using these properties, the paragraph solves for unknown angles within a cyclic quadrilateral. It also revisits the concept of angles subtended by the same arc being equal, which is used to find the values of angles in different segments of the circle. The paragraph concludes by summarizing the properties of circles and their angles, and hints at upcoming videos that will cover past paper questions related to these topics.

Keywords

💡Circle

A circle is defined as the set of all points in a plane that are equidistant from a fixed point, known as the center. This concept is central to the video's theme, which is to explore the properties of circles. The script uses the circle to introduce other geometrical concepts such as radius, diameter, and circumference.

💡Center

The center of a circle is the fixed point from which all points on the circle are equidistant. It is a fundamental concept in the video, as it helps explain the formation of a circle and is used to define other properties such as radius.

💡Radius

The radius of a circle is the distance from the center to any point on the circumference of the circle. It is a key term in the script, used to define the size of a circle and in formulas such as the circumference, which is calculated as 2πr.

💡Circumference

Circumference refers to the perimeter or the total length of the circle's edge. In the video, it is explained as being related to the radius, with the formula for circumference being 2πr, where r is the radius of the circle.

💡Cord

A cord in the context of a circle is any line segment that connects two points on the circle. The script mentions that a cord that passes through the center and divides the circle into two equal parts is known as the diameter.

💡Diameter

The diameter of a circle is the longest possible cord, which passes through the center and divides the circle into two equal halves. The script explains that the diameter is twice the length of the radius, written as D = 2R.

💡Tangent

A tangent to a circle is a line that touches the circle at exactly one point. The video script discusses the property of tangents, stating that the tangent at any point is perpendicular to the radius at that point.

💡Secant

A secant is a line that intersects a circle at two distinct points. The script contrasts secants with tangents, explaining that while tangents touch the circle at one point, secants intersect it at two.

💡Perpendicular Bisector

In the video, the perpendicular bisector of a chord is a line that is perpendicular to the chord and passes through its midpoint. This concept is used to explain the symmetry and properties of circles, such as equal chords having equal distances from the center.

💡Angle in a Semicircle

The script states that any angle that is formed by a semicircle (a diameter) is always a right angle, or 90 degrees. This is a fundamental property of circles used to solve various geometric problems and is demonstrated in the video through examples.

💡Cyclic Quadrilateral

A cyclic quadrilateral is a quadrilateral where all four vertices lie on the circumference of a circle. The video discusses properties of cyclic quadrilaterals, such as the sum of opposite angles being supplementary (equal to 180 degrees).

Highlights

Definition of a circle as a set of points equidistant from a fixed point, known as the center.

Explanation of the radius as the distance from the center to the circumference of a circle.

Circumference of a circle is calculated as 2πr, where r is the radius.

Introduction to the concept of a cord in a circle, dividing it into two parts.

Definition of a diameter as the longest cord that divides the circle into two equal parts, being twice the radius.

Tangent is a line that touches the circle at a single point.

Secant is a line that intersects the circle at two points, extending beyond the circle.

Property of equal chords and the perpendicular bisector in a circle, dividing the chord into two equal parts.

The property that any angle in a semicircle is always 90 degrees.

Tangent lines from an external point to a circle are equal in length.

Angle between a tangent and the radius is always 90 degrees.

Angle at the center of a circle is twice the size of the angle on the circumference subtended by the same arc.

Property of angles in the same segment of a circle being equal.

Cyclic quadrilateral is defined by all vertices lying on the circumference of a circle.

Sum of all angles in a cyclic quadrilateral is 360 degrees.

Opposite angles in a cyclic quadrilateral are supplementary.

Practical application of circle properties to solve geometry problems.

Transcripts

in this session we will cover the topic

related to Circle and its

properties hello and welcome to the

session so today we are going to cover

Circle and the circle properties what is

circle circle is the set of point which

is always equid distance from the fixed

point and the fixed point is nothing but

the center okay so center of a circle

okay from the fixed point to all the

point which are equal distance if I will

try to draw a line this line is nothing

but all the distance between these two

point is known as

radius okay so radius is the distance

from Center to the circumference of a

circle okay now this whole length right

this whole length is known as

circumference okay or the perimeter we

can say circumference of a circle so

circumference is nothing but 2 into piun

into R where R is a radius okay now

there is a if you see this is one line

which is dividing the circle in two

parts this line is nothing but the cord

so cord is a line which divide the

circle in two parts okay now if you'll

see a cord which is passing through the

center it is dividing into two equal

parts right so so that cord or the

longest cord is known as

diameter okay so diameter is nothing but

it is a longest cord which divide the

circle in two equal parts and this is

the two times of the radius so if you'll

see r + r which is going to be the

diameter so D is equal 2 R it is the

relation between the diameter and the

radius now tangent tangent is a line

which touches the circle at a single

point if you if I draw a line like this

one if you'll see at this point it is

touching right so it touches the circle

at a single point so we can say this is

nothing but

tangent okay so tangent is a line which

touches the circle at a single point

okay now secant secant is a line it's a

extension of the cord but like like this

that means it is what intersecting at

two point so when it intersect at two

point we call it a secant okay so secant

is nothing but it is an extension of

a Cod but it intersect at two point

instead of touching like if you see it

is inside the circle it is what outside

it is intersecting at this point and

this point so we call it

a second okay now coming to the circle

properties equal cord and the

perpendicular bis sector okay so if

suppose if I'm going to draw a circle

consider this is a circle

okay if I draw a cord okay so any line

which is drawn from

cord to the circle if you'll see this

one right so this line is perpendicular

if this line is perpendicular then it

bisect the cord in two equal part okay

okay also if the circle is like consider

this is a circle I'm considering this

circle okay

now one cord is this one and just take

another cord is this one if cords are

equal

right okay say if cords are

equal then their distance is also same

from the center okay okay so equal cord

and the perpendicular bis sector if

these are the perpendicular bis sector

then it will divide into the two equal

part it will divide into the two equal

part this we call it radius this we call

it a radius okay in this scenario we can

take this as a radius and this as a

radius if you'll see here these two

triangles are congruent right so if

those two triangles are congruent that

means each part like this angle is

equals to this this angle is going to be

equals to this this angle and this angle

is going to be equal so this is one the

cord if a line drawn perpendicular from

cord uh from center of the circle to the

cord it will bisect the cord okay or in

other way we can say if a line which is

bisecting the

cord then it will be perpendicular to

the cord okay now next if you'll see

here tangent we already discussed right

tangent is the line which touches the

circle at a single point now any tangent

which is drawn from external point right

how many triangles we can draw two

triangles maximum we can draw two

triangles if you'll see and this tangent

length of the tangent is always going to

be same that means AC is equals to

BC okay so in this scenario if we'll see

AC and BC is equals to be same OB and O

A is radius so I'm going to write here

radius r

okay this and this is going to be same

so I'm going if it is X then this is

also going to be X right and this is

what a common right if you'll see this

is what common so both the triangles are

congruent if both the triangles are

congruent using rhs right angle rhs is

like right angle hypotenuse and one side

right angle is there if you'll see

hypoten is OC and one side is like OB

and O A we can consider right so in this

scenario both the triangles are

congruent if both the triangles are

congruent in that scenario each and

every part will be equal that means this

going to be equals to this right this

angle is going to be this one okay so if

you'll see we'll use the same property

over here this is 20 so I'm going to

write here this is going to be 20 if

this is X I'm going to write here x

now this is what radius and tangent is

always going to be 90° so this is going

to be 90 90 20 and X this is a right

angle triangle right so we can write

here x + 90 + 20 is = 180 x is going to

be 180 - 110 x = to 70° so like this way

we can identify the value of x angle in

a semicircle is always going to be 90°

if you see this is a Ab is a what cord

which is the longest cord which is

dividing into the two parts so this is

what AB this part is semicircle so angle

any angle if you draw it is always going

to be 90° angle in a semicircle is

always going to be 90° so this is 90

this is going to be 90 C1 and C2 is

going to be same so let's solve this

question if you'll see the first

question this is going to be what I

writing here this is 90° because angle

in a semicircle now this angle we know 9

20 + 45 so this angle we can suppose y

so

X sorry 45 + 90 + y is going to be 180 y

= 180 - 90 + 45

135 subtract it 80 - 5 so this is 5 7 -

3 is 5

55 okay uh 45 okay so 45 my bad so 4 45

so this angle is 45 so I can write here

this is 45 so X we have to identify X is

equal to 18 - 45 why because this is a

linear pair straight line so I can write

here x equal to 135 or we can use

directly property this is what external

angle this is what opposite to external

angle right external angle is equals to

the sum of the Interior opposite angle

that means 90 + 45 which is going to be

135 okay similarly here it is going to

be 90 so this is going to be 20 right

because 1 10 so this is going to be

70 90 70 and 20 like this way we can

write angle between the tangent and the

radius of the circle so angle between

the tangent tangent is always going to

be

90 okay tangent is always going to

perpendicular to the radius that means

this is 90° okay let's solve this one

this is going to be 90° this is going to

be

90° and this is what x so this is what a

quadrilateral x + 90 + 140 + 90 is going

to be 360 x is going to be 360 - 180 -

140 right 9090 180 and - 140 180 - 40

which is going to be 40 or we can

directly

write angle at the center of a circle

so angle at in this if you the angle

subtended at the center of a circle by

an arc this is one Arc which is subing

angle at the center is always twice the

size of the angle on the circumference

subtended by the same Ark so if it is

going to be X then this is going to be

2x if it is going to be 2x then this is

going to be X okay so this is our angle

at the center property so in this case

if you'll see o

this is going to be 40 I'm going to

write why 40 because this is what radius

this is what radius this is an isos

triangle so this angle we can find out

why because angle some property right so

40 40 and this is going to be 100 so

angle I'm writing a z so Z is going to

be 100 now angle at the center by this

Arc if you'll see it is making angle Z

which is 100 so X is going to be half of

this which is going to be

50 okay now we know X now this is what

if you see this and this is equal that

means this angle and this angle is going

to be equal so I am going to write here

x this is going to x y +

40 y +

40 again y + 40

because these two angle will be equal

right if it is 40 40 this is y so this

is also going to be y so y +

40 is equal to 180° so in this scenario

I can write here x 2 * of y + 40 isal to

180 x we know right 50 substitute It 2 y

+ 40 is going to be 180 -

50 y + 40 is going to be 130 / 2 which

is 65 so y = 25 so like this way we can

find out the value of y angle in the

same segment so angle in the same

segment are always equal so if you'll

see this is one Arc which is making

angle X and again this is also making

angle X so those are equal similarly

here if 33 this is what making 33 with

the help of this Arc right so this is B

also going to be 33 so like this way we

can use this property to identify the

value of angle in the same Arc okay now

if you'll see this angle how we can find

this angle and this angle is going to be

equal why because this is what opposite

angles right so if it is X then this is

going to be X right and again if I use

this property angle in the same segment

right

X then this is suppose y so y so this is

going to be Y and Y right so angle some

using this property we can identify the

value of missings okay so like this way

we can fine now if you see cyclic

quadrilateral what is cyclic

quadrilateral if all the point of a

quadrilateral if you see p q r s all the

vertices of a quadrilateral are lying on

the circum of a circle or on the circle

we can say cyclic quadal so what is

property of a cyc quadrilateral First

Property simple quadrilateral if it is

there then sum of all the angle is going

to be 360

PQ r + S equal to

360° another property of the CYCC

quadrilateral is opposite angle sum of

the opposite angle is going to be

supplementary that means p+ angle R is

going to be

180 angle S Plus angle Q is going to be

180 so simple case this is also a

example of cyc quad a + 100 is 180 so a

is going to be 80° and B is going to 180

minus

115 subtract it 65 so like this way we

can identify so this is all about this

circle and the properties of a circle I

will also come with another videos were

from the past paper questions please

share and subscribe thank you for

watching