Introduction to Sets - Elements and Subset of a Set | Grade 7 Math @MathTeacherGon
Summary
TLDRIn this educational video, the host, Mythic, introduces the concept of sets in mathematics, specifically for grade 7. Sets are defined as collections of items, with examples including the English alphabet and the colors of the rainbow. The video explains that each item in a set is called an 'element' and uses capital letters to denote sets. The host further discusses subsets, illustrating with examples how a set can be a subset of another if all its elements are contained within the parent set. The video is interactive, encouraging viewers to ask questions and request topics for future discussions.
Takeaways
- 📚 A set is defined as a collection or group of distinct objects, which can be numbers, people, letters, etc.
- 🌈 The English alphabet and the colors of the rainbow are given as examples of sets, illustrating the concept with familiar items.
- 🔤 Sets are named using capital letters, emphasizing the convention of using uppercase for set notation.
- 📝 Each object within a set is referred to as an 'element', and the script explains how to denote elements with specific symbols.
- 🗓️ An example of a set is provided, listing the days of the week, and explaining how each day is an element of that set.
- ❌ The script clarifies that not all collections are sets, using December as an example of something not included in the set of days in a week.
- 🔑 The concept of a subset is introduced, explaining that it is a set whose elements are all members of another set.
- 📐 The subset relationship is symbolized, with one symbol indicating subset and another indicating not a subset, providing a visual understanding of the relationship.
- 🔢 An example is used to demonstrate how to determine if one set is a subset of another, using sets A and B with numerical elements.
- ❌ The script also shows how to determine when a set is not a subset, using set C as an example where its elements are not found in set A.
Q & A
What is the definition of a set according to the video?
-A set is a collection or an arrangement of items, such as the English alphabets or the colors of the rainbow.
How are sets named in mathematics?
-Sets are named using capital letters, for example, Set A, Set B, etc.
What is another term for the items within a set?
-The items within a set are referred to as elements.
What symbol is used to denote an element of a set?
-The symbol for an element of a set is ∈, which means 'is an element of'.
Can you provide an example of a set from the video?
-An example of a set given in the video is the set of days in a week, which includes Monday, Tuesday, Wednesday, Thursday, Friday, Saturday, and Sunday.
What is the term for a set where all of its elements are also elements of another set?
-A subset is a set whose elements are all members of another set.
How is the relationship between a subset and its parent set represented?
-The relationship between a subset and its parent set is represented using the subset symbol ⊆.
Is it possible for a set to be a subset of itself?
-Yes, every set is a subset of itself because all elements of a set are also members of the same set.
What is the condition for Set B to be a subset of Set A?
-Set B is a subset of Set A if all elements of Set B are also elements of Set A.
Why is Set C not a subset of Set A according to the video?
-Set C is not a subset of Set A because not all elements of Set C are found within Set A.
How can you determine if a set is a subset of another set?
-You can determine if a set is a subset of another set by checking if every element of the first set is also an element of the second set.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

MATEMATIKA HIMPUNAN BILANGAN CACAH
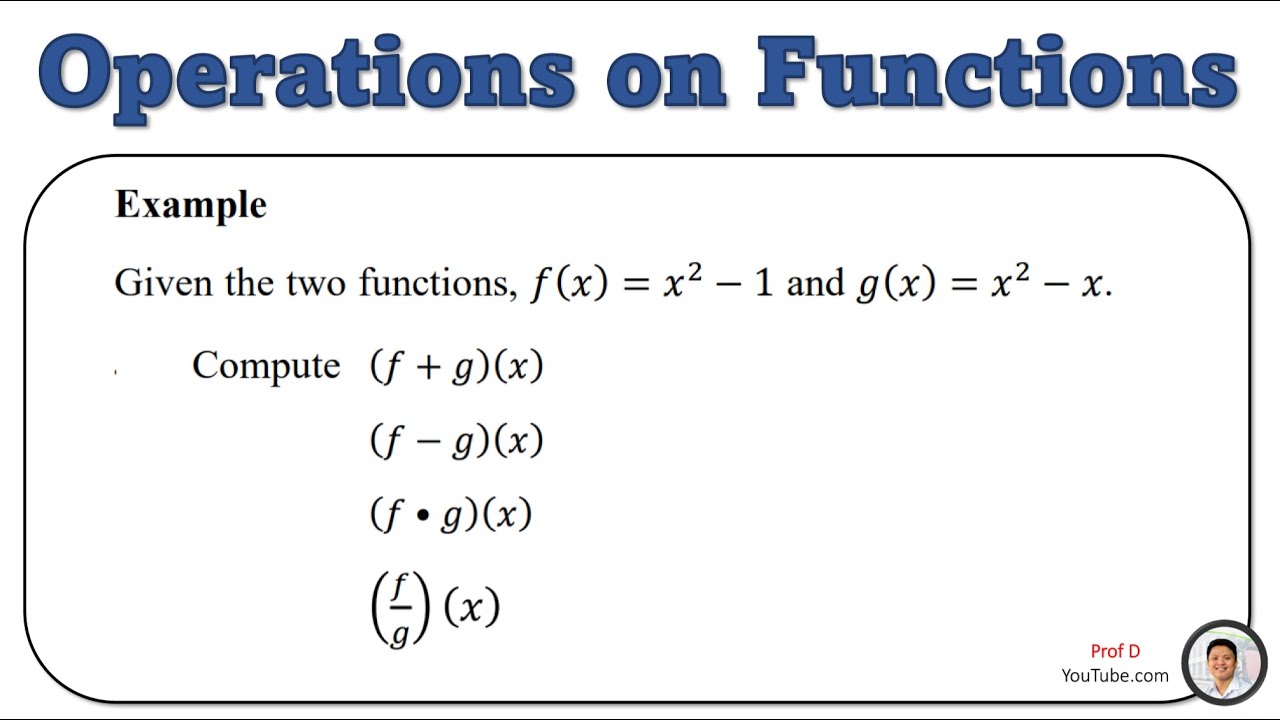
Grade 11 | Operations on Functions | General Mathematics

Pola Bilangan (5) | Barisan dan Deret Geometri

Konsep Persamaan Linier Satu Variabel Hal 97-109 Bab 3 PLSV Kelas 8 Kurikulum Merdeka Belajar
Introduction to Sets || Mathematics in the Modern World

SHS General Mathematics Q1 Ep1: Function
5.0 / 5 (0 votes)