Laplace Transform - First Shifting Theorem with Example | By GP Sir
Summary
TLDRIn this educational video, the instructor introduces the 'First Shifting Theorem' in the context of Laplace Transforms, focusing on its application for both direct and inverse transformations. The video simplifies the concept with straightforward examples, emphasizing the importance of understanding the theorem's implications rather than its proof. The instructor demonstrates how to apply the theorem to find the Laplace Transform of given functions and then invert them, using additional examples for clarity. The lecture aims to ensure comprehension and encourages students to engage with any lingering questions in the comment section. The video concludes with a call to action for viewers to share and subscribe to the channel.
Takeaways
- 📚 The video discusses the 'First Shifting Theorem' in the context of Laplace Transforms.
- 🔍 The theorem is used to find the Laplace Transform and its inverse.
- 📝 The presenter provides simple statements of the theorem without focusing on the proof.
- 📈 Three examples are given to demonstrate how to find the Laplace Transform using the theorem.
- 🔄 The 'First Shifting Theorem' is also applicable for inverse Laplace Transforms.
- 📑 The video includes examples to illustrate finding the inverse Laplace Transform.
- 🤔 The presenter assures that the concept is simple and that proofs will be taught when necessary.
- 👨🏫 The lecture aims to ensure understanding of the first shifting theorem for both Laplace and inverse Laplace Transforms.
- 💬 Students are encouraged to ask questions in the comment section if they have doubts.
- 📢 The presenter invites viewers to share and subscribe to the channel for more educational content.
Q & A
What is the 'First Shifting Theorem' in the context of Laplace Transform?
-The 'First Shifting Theorem' is a principle in Laplace Transform that allows for the simplification of finding the Laplace Transform of functions that are shifted in time. It is a simple concept that is crucial for solving problems involving time delays or advanced functions.
How does the 'First Shifting Theorem' simplify the process of finding Laplace Transforms?
-The 'First Shifting Theorem' simplifies the process by providing a direct formula to calculate the Laplace Transform of a function that is time-shifted. This avoids the need for complex integrations and makes it easier to handle functions with time delays.
What are the implications of the 'First Shifting Theorem' that students need to remember?
-Students need to remember that the 'First Shifting Theorem' allows them to easily find the Laplace Transform of functions that are shifted in time by a certain amount. The theorem provides a straightforward way to account for this time shift in the transform.
Is it necessary to understand the proof of the 'First Shifting Theorem' to use it effectively?
-While understanding the proof can be beneficial, it is not necessary for practical use. The focus should be on the application of the theorem to solve problems, and the proof can be studied when needed for a deeper understanding.
Can you provide an example of how the 'First Shifting Theorem' is applied to find the Laplace Transform?
-Sure, if you have a function f(t-a)u(t-a) where u(t) is the unit step function, the Laplace Transform using the 'First Shifting Theorem' would be e^{-as}F(s) where F(s) is the Laplace Transform of f(t).
What is the inverse process of the 'First Shifting Theorem'?
-The inverse process of the 'First Shifting Theorem' is used to find the inverse Laplace Transform of a function. It helps in determining the original time function when given its Laplace Transform, especially when the function has been shifted in the s-domain.
How does the 'First Shifting Theorem' assist in finding the inverse Laplace Transform?
-The 'First Shifting Theorem' assists in finding the inverse Laplace Transform by providing a method to shift the function back in time. It allows for the reconstruction of the original time-domain function from its Laplace Transform representation.
Can you give an example of using the 'First Shifting Theorem' for inverse Laplace Transform?
-If the Laplace Transform of a function is given as e^{-as}F(s), using the 'First Shifting Theorem' for inverse Laplace Transform, the original function can be found as f(t-a)u(t-a) where F(s) is the Laplace Transform of f(t).
What are the key takeaways from the lecture on the 'First Shifting Theorem'?
-The key takeaways include understanding what the 'First Shifting Theorem' is, how to use it to find Laplace Transforms of time-shifted functions, and how to apply it to find the inverse Laplace Transform. The lecture also emphasized the importance of knowing the implications of the theorem without necessarily focusing on the proof.
How can students ensure they understand the 'First Shifting Theorem' and its applications?
-Students can ensure their understanding by practicing with various examples, reviewing the theorem's implications, and asking questions in the comment section if they have doubts. Engaging with the material through practice and discussion is key to solidifying the concepts.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

LAPLACE TRANSFORM | MATHEMATICS | LECTURE 03 | First Shifting Theorem | PRADEEP GIRI SIR
Second Shifting Theorem | Type 2 Problems | t Shift | Heaviside Theorem | Laplace transform | Maths
PROPERTIES OF LAPLACE TRANSFORM
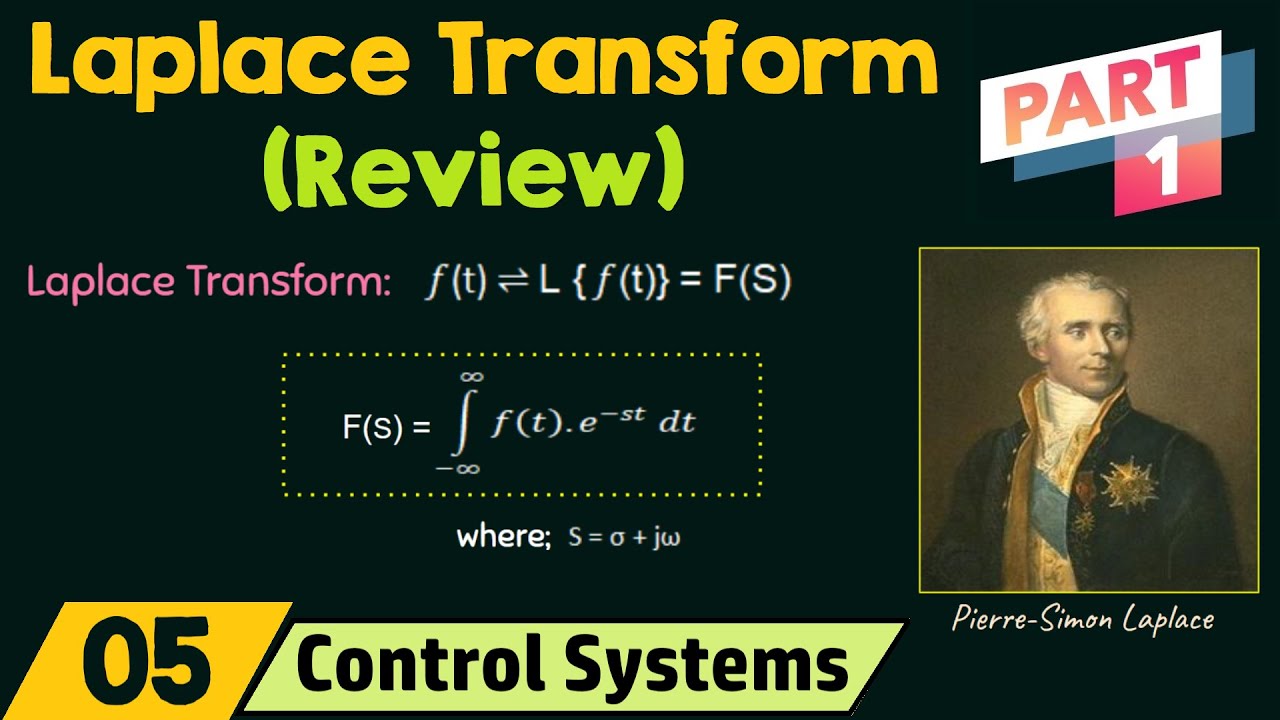
Review of Laplace Transform (Part 1)
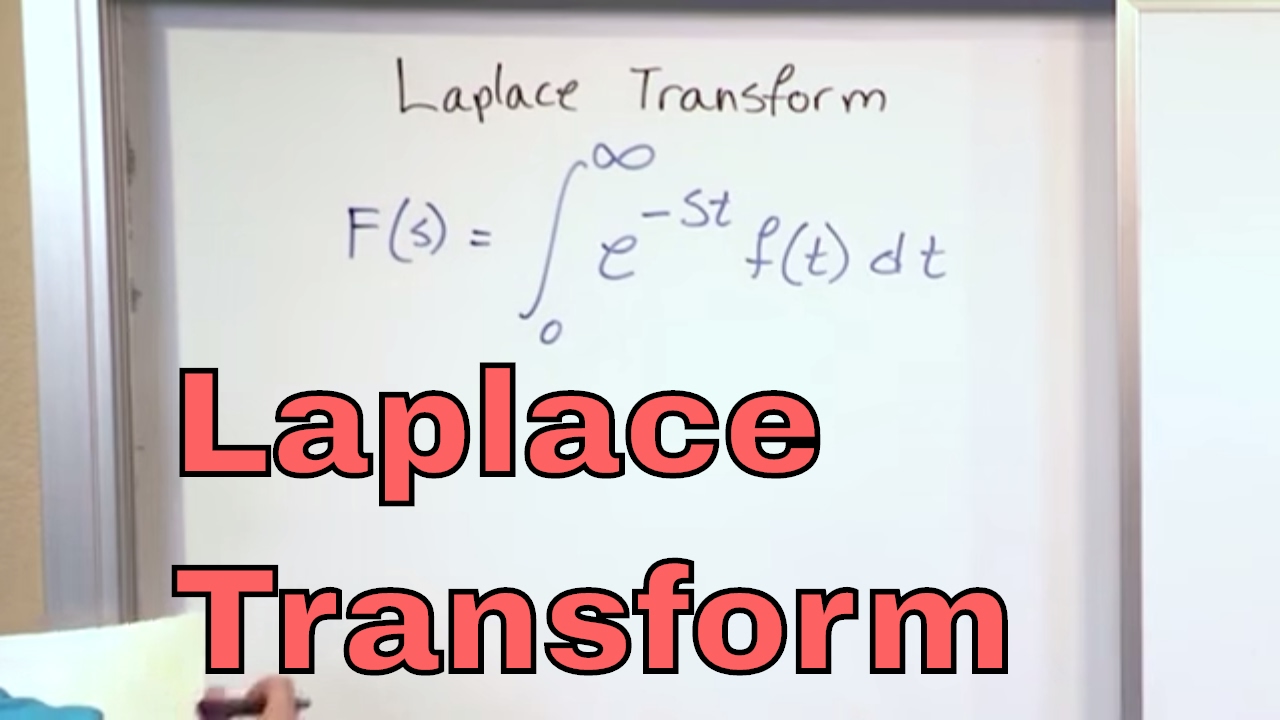
Lesson 1 - Laplace Transform Definition (Engineering Math)

Inverse Laplace Transform (Pecahan Parsial)
5.0 / 5 (0 votes)