กลศาสตร์วิศวกรรม มทร.อีสาน Centroid
Summary
TLDRThis video tutorial offers a detailed explanation on how to calculate the centroid of a composite shape. It covers the analysis of a problem involving a combination of a triangle, a square, and a circle. The instructor uses the composite body method, breaking down the shapes and determining their individual centroids and areas. A step-by-step calculation is provided, including the use of a table to organize and compute the necessary values. The video is aimed at engineering students studying statics and anyone interested in learning how to find centroids of complex shapes.
Takeaways
- 📘 The video is a tutorial focusing on exercise 1 of chapter 9, which is about analyzing and finding the centroid of a composite area.
- 📐 The problem presented involves calculating the centroid position of a composite shape defined by a rectangle, a triangle, and a circle.
- 🔢 The formulas used to find the centroid involve calculating the average X (X Bar) and Y (Y Bar) coordinates, which are the mean values of all X and Y coordinates of the shape.
- 📊 The tutorial uses the composite body method, which involves dividing the composite shape into simpler shapes and finding the centroid of each.
- 📏 The tutorial demonstrates how to calculate the centroid by breaking down the problem into steps, including defining the shape, assigning coordinates, and calculating areas and centroid coordinates for each part.
- 📋 The video explains the process of calculating the centroid using a tabular method, which involves organizing the data and calculations in a table for clarity.
- 📈 The tutorial covers how to compute the centroid coordinates for each individual shape (triangle, rectangle, and circle) by using geometric properties and formulas.
- 📝 The video script includes detailed calculations, such as multiplying the base and height for the area of triangles and rectangles, and using the radius for the area of the circle.
- ✅ The final step in the tutorial is to substitute the calculated values back into the formulas to find the overall centroid of the composite shape as given by the problem.
- 🔍 The video concludes by verifying the calculated centroid coordinates and marking the centroid point on the shape, which is the solution to the problem presented.
Q & A
What is the main topic discussed in the video script?
-The main topic discussed in the video script is the calculation of the centroid of a composite shape, which is part of a lesson on the analysis of areas and centroids.
What shapes are included in the composite shape for the centroid calculation?
-The composite shape includes a rectangle, a triangle, and a circular segment.
What method is used to calculate the centroid of each individual shape?
-The method used to calculate the centroid of each individual shape involves determining the x-bar and y-bar coordinates, which are the average x and y coordinates of the shape's vertices.
How is the centroid of the composite shape calculated?
-The centroid of the composite shape is calculated by using the composite body method, which involves breaking down the composite shape into individual shapes, calculating their centroids, and then finding the weighted average of these centroids.
What is the significance of the x-bar and y-bar values in the centroid calculation?
-The x-bar and y-bar values represent the average x and y coordinates of the centroids of the individual shapes that make up the composite shape. These values are used to find the overall centroid of the composite shape.
What is the purpose of calculating the area of each individual shape?
-The area of each individual shape is calculated to determine the weight of each shape in the composite body method, which is necessary for finding the weighted average of the centroids.
How is the x-coordinate of the centroid of the composite shape determined?
-The x-coordinate of the centroid of the composite shape is determined by summing the products of the x-bar values and the areas of the individual shapes, and then dividing by the total area of the composite shape.
What does the term 'composite body method' refer to in the context of the video script?
-In the context of the video script, the term 'composite body method' refers to a technique used to find the centroid of a complex shape by dividing it into simpler shapes, finding the centroids of these simpler shapes, and then calculating the overall centroid based on their weighted averages.
What is the significance of the negative values used in the calculation of the centroid?
-Negative values in the calculation of the centroid indicate that a portion of the shape is subtracted from the composite area, such as when a circular segment is removed from a rectangle.
How are the final coordinates of the centroid presented in the video script?
-The final coordinates of the centroid are presented as the x-bar and y-bar values, which are calculated based on the weighted averages of the individual shape centroids and their respective areas.
What is the educational value of the video script for students?
-The video script is educational for students studying engineering mechanics and those interested in learning how to calculate centroids of composite shapes as per the problem statement.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Finding the Centroid with First Moment of Area Example Problems - Structural Analysis
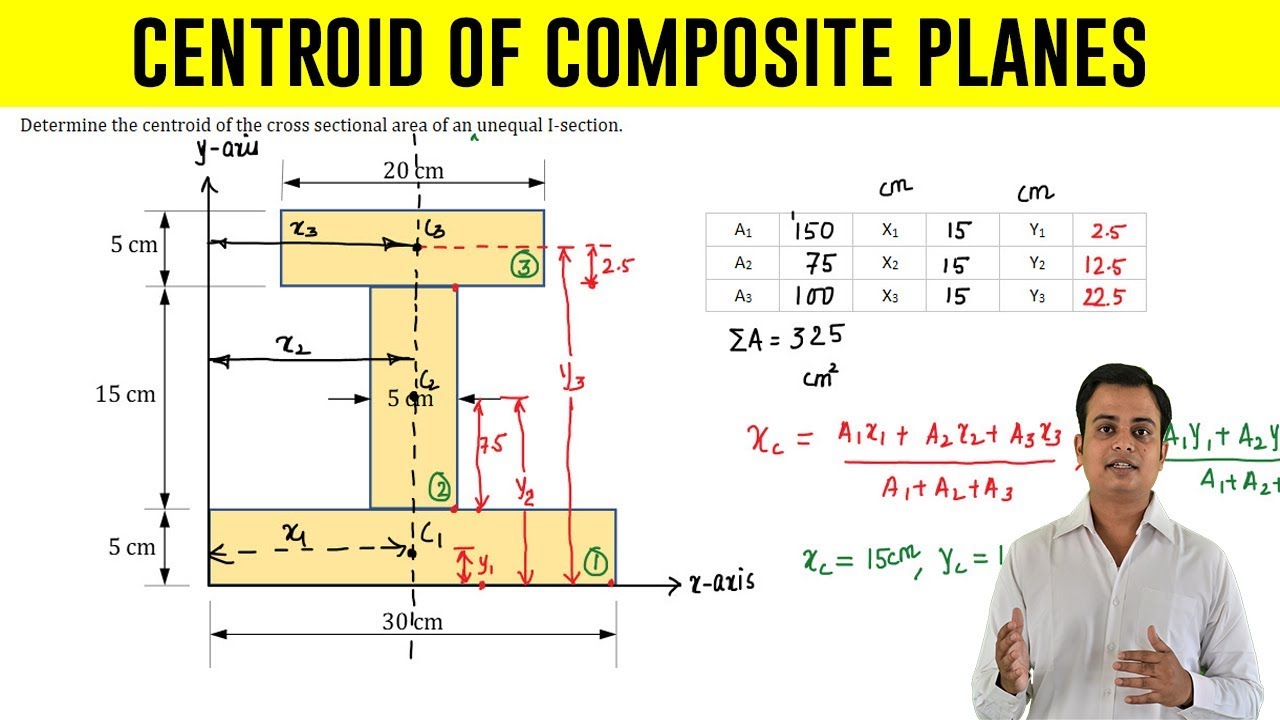
How to find Centroid of an I - Section | Problem 1 |
DIVISIÓN DE UN SEGMENTO EN UNA RAZÓN DADA

CARA MUDAH FUNGSI KOMPOSISI

Contoh Perhitungan Analisa Balok Lentur dengan Tekuk Lokal | SNI 1729-2015 Struktur Baja Lightboard

4.3a Density and Composite Mixture (MJ16 P12 Q13) | AS Density | Cambridge A Level Physics
5.0 / 5 (0 votes)