Probabilidades - Ejercicios Resueltos - Nivel 3
Summary
TLDRIn this video, Jorge from Mate Móvil explains how to calculate the probability of not rolling a '2' when rolling a die 'n' times. The process involves using the multiplication principle to determine the total possible outcomes (6^n) and the favorable outcomes (5^n, where no '2' appears). The final probability is calculated as (5/6)^n. Jorge also introduces a challenge problem to calculate the probability of rolling at least one '2' in 'n' rolls, providing an engaging explanation of probability concepts. The video is informative for those learning about probability and dice-related problems.
Takeaways
- 😀 The problem involves calculating the probability of not rolling a 2 when rolling a die n times.
- 😀 The approach uses the classical probability formula: favorable outcomes divided by total possible outcomes.
- 😀 The total number of possible outcomes when rolling n dice is 6^n, as each die has 6 possible outcomes.
- 😀 The principle of multiplication is applied to calculate the total possible outcomes when multiple events (dice rolls) occur.
- 😀 Each individual die roll has 6 possible results, represented as 6 raised to the power of n for n dice.
- 😀 To calculate favorable outcomes (cases where no 2 is rolled), each die can result in any of 5 possible outcomes (1, 3, 4, 5, or 6).
- 😀 The number of favorable outcomes is 5^n because each die has 5 possible results excluding the 2.
- 😀 The probability of no 2 being rolled in n dice throws is 5^n / 6^n, which simplifies to (5/6)^n.
- 😀 This problem illustrates a common probability technique involving the complement rule: finding the probability of an event happening by subtracting the probability of its complement.
- 😀 The video concludes with a challenge: calculate the probability of rolling at least one 2 in n dice rolls, with a hint provided for further study.
Q & A
What is the main problem being addressed in the video?
-The main problem is calculating the probability that no '2' will appear when a die is rolled 'n' times.
How do you calculate the total number of possible outcomes when rolling a die 'n' times?
-To calculate the total number of possible outcomes, you multiply the number of results from each die roll. Since each die has 6 possible outcomes, the total is 6 raised to the power of 'n', or 6^n.
What is the classical probability formula used in the video?
-The classical probability formula is the number of favorable outcomes divided by the total number of possible outcomes.
What is the number of favorable outcomes when calculating the probability that no '2' appears?
-The number of favorable outcomes is the number of results where '2' does not appear. For each die roll, there are 5 possible outcomes (1, 3, 4, 5, or 6), so the favorable outcomes are 5^n.
How do you calculate the probability that no '2' will appear in 'n' rolls?
-The probability is calculated by dividing the number of favorable outcomes (5^n) by the total number of possible outcomes (6^n). This results in the probability being (5/6)^n.
What mathematical principle is used to calculate the total number of outcomes when rolling multiple dice?
-The multiplication principle is used. If there are 'm' ways to perform the first event, 'n' ways to perform the second, and so on, the total number of outcomes is the product of these numbers.
What does the term 'n' represent in the video?
-'n' represents the number of times the die is rolled.
Why is 5^n used for favorable outcomes instead of 6^n?
-5^n is used for favorable outcomes because each die roll can result in one of five outcomes (1, 3, 4, 5, or 6), excluding the number '2'.
What happens if a '2' does appear in one of the rolls?
-If a '2' appears in any of the rolls, the calculation for favorable outcomes would not apply, as we are specifically calculating for the case where no '2' appears.
What is the final expression for the probability that no '2' will appear in 'n' rolls?
-The final expression is (5/6)^n, which represents the probability that no '2' will appear after rolling a die 'n' times.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео

Frekuensi Harapan

Probability explained | Independent and dependent events | Probability and Statistics | Khan Academy
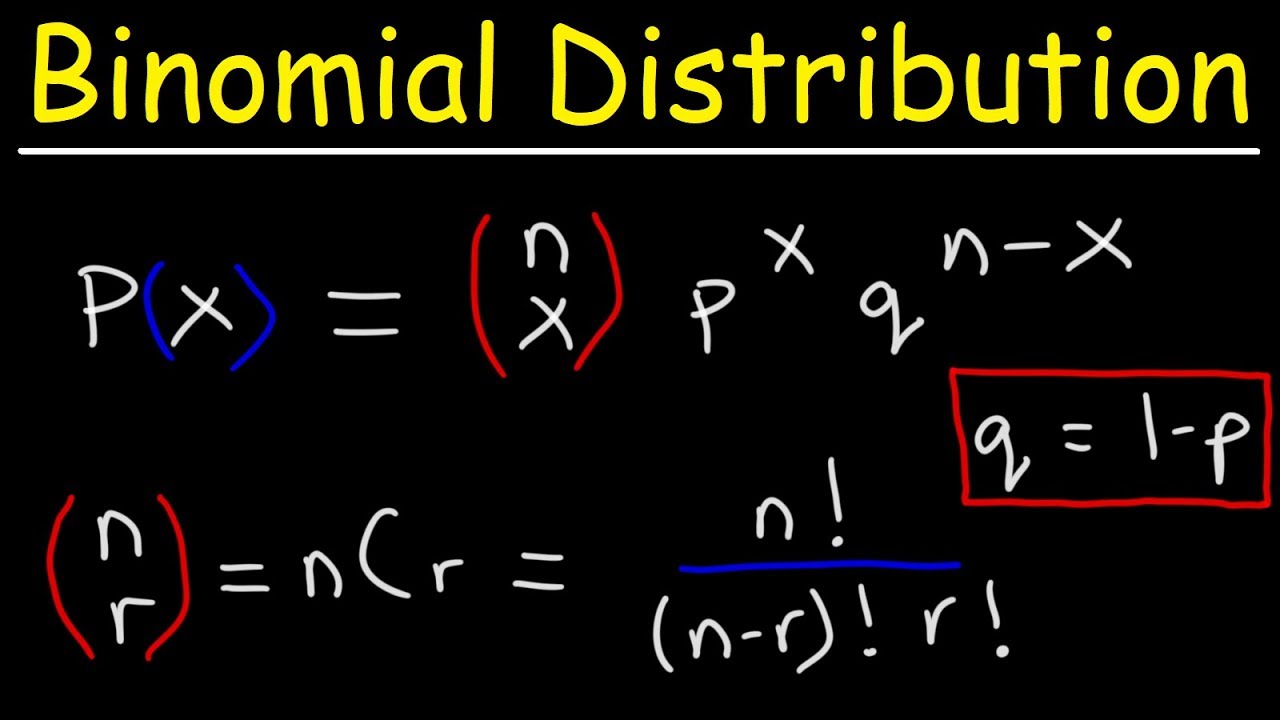
Finding The Probability of a Binomial Distribution Plus Mean & Standard Deviation

Ruang Sampel dan Peluang Suatu Kejadian (Materi dan Contoh Soal diserta Pembahasan)

Matematika Kelas 8 Bab 6 Peluang - Cara Menentukan Peluang - hal. 176 - 178 - Kurikulum Merdeka

Peluang Kejadian Saling Lepas dan Kejadian Saling Bebas
5.0 / 5 (0 votes)
