GCSE Maths - What are Inequalities? (Inequalities Part 1) #56
Summary
TLDRThis video provides a comprehensive introduction to inequalities, explaining the meanings of various symbols and how to represent them on a number line. The presenter clarifies concepts like 'less than' and 'more than,' as well as their inclusive counterparts, 'less than or equal to' and 'more than or equal to.' Key examples illustrate how to handle negative numbers and compound inequalities. The video concludes with a step-by-step demonstration of graphing inequalities, reinforcing the concepts with clear visuals and engaging explanations, making it accessible for students seeking to understand this fundamental math topic.
Takeaways
- 😀 Inequalities express relationships between numbers, using symbols like < and >.
- 📉 The less than sign (<) indicates that the value on the left is smaller than the value on the right.
- 📈 The greater than sign (>) indicates that the value on the left is larger than the value on the right.
- ⚖️ Symbols like ≤ and ≥ mean 'less than or equal to' and 'greater than or equal to,' respectively.
- 📝 An easy way to remember the less than symbol is to think of it as resembling an 'l' for 'less.'
- 🔄 The inequality x < 10 is equivalent to 10 > x, showing that the relationships can be reversed.
- ➖ Negative numbers can also be used in inequalities, such as x < -5, meaning x can be any number less than -5.
- 📊 Compound inequalities combine multiple conditions, such as 3 < x ≤ 7, indicating x is between 3 and 7.
- 🔵 When graphing inequalities, use open circles for values not included and filled circles for values that are included.
- 🖊️ Practicing how to represent inequalities on number lines is crucial for visual understanding.
Q & A
What does the symbol '<' signify in an inequality?
-The symbol '<' indicates that a variable, such as x, is less than a specific value. For example, x < 10 means x can be any number less than 10.
How can students remember the difference between '<' and '>'?
-One helpful way is to associate the less than sign '<' with the letter 'L', which stands for 'less'. The more than sign '>' does not resemble 'L', indicating it represents 'more'.
What does '≤' mean in an inequality?
-The symbol '≤' means 'less than or equal to'. For instance, x ≤ 10 means x can be any number less than 10, including 10 itself.
How is '≥' different from '>'?
-'≥' stands for 'more than or equal to', meaning that the variable can either be greater than or exactly equal to a specific value. In contrast, '>' means the variable must be strictly greater than that value.
Can inequalities include negative numbers?
-Yes, inequalities can include negative numbers. For example, x < -5 means x can be any value less than -5, such as -5.5 or -100.
What is a compound inequality?
-A compound inequality combines two inequalities. For example, 3 < x ≤ 7 means that x is greater than 3 and less than or equal to 7, indicating x must be between 3 and 7.
How can you represent inequalities on a number line?
-To represent inequalities on a number line, mark the relevant values, using open circles for values that cannot be included and closed circles for those that can be included. Connect the points to show the range.
What does the expression 'x < 10' imply about possible values for x?
-The expression 'x < 10' implies that x can be any value less than 10, such as 9, 8, 3, or -4.
In the inequality '3 < x ≤ 7', which whole numbers can x take?
-In the inequality '3 < x ≤ 7', the whole numbers x can take are 4, 5, 6, and 7. However, x cannot be 3.
What should you do when drawing a number line for the inequality '-3 ≤ x < 4'?
-When drawing a number line for '-3 ≤ x < 4', place a closed circle at -3 to indicate that x can equal -3, and an open circle at 4 since x cannot equal 4. Then connect these points to show the range of x.
Outlines

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифMindmap

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифKeywords

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифHighlights

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифTranscripts

Этот раздел доступен только подписчикам платных тарифов. Пожалуйста, перейдите на платный тариф для доступа.
Перейти на платный тарифПосмотреть больше похожих видео

Inequalities | Tagalog Tutorial Video
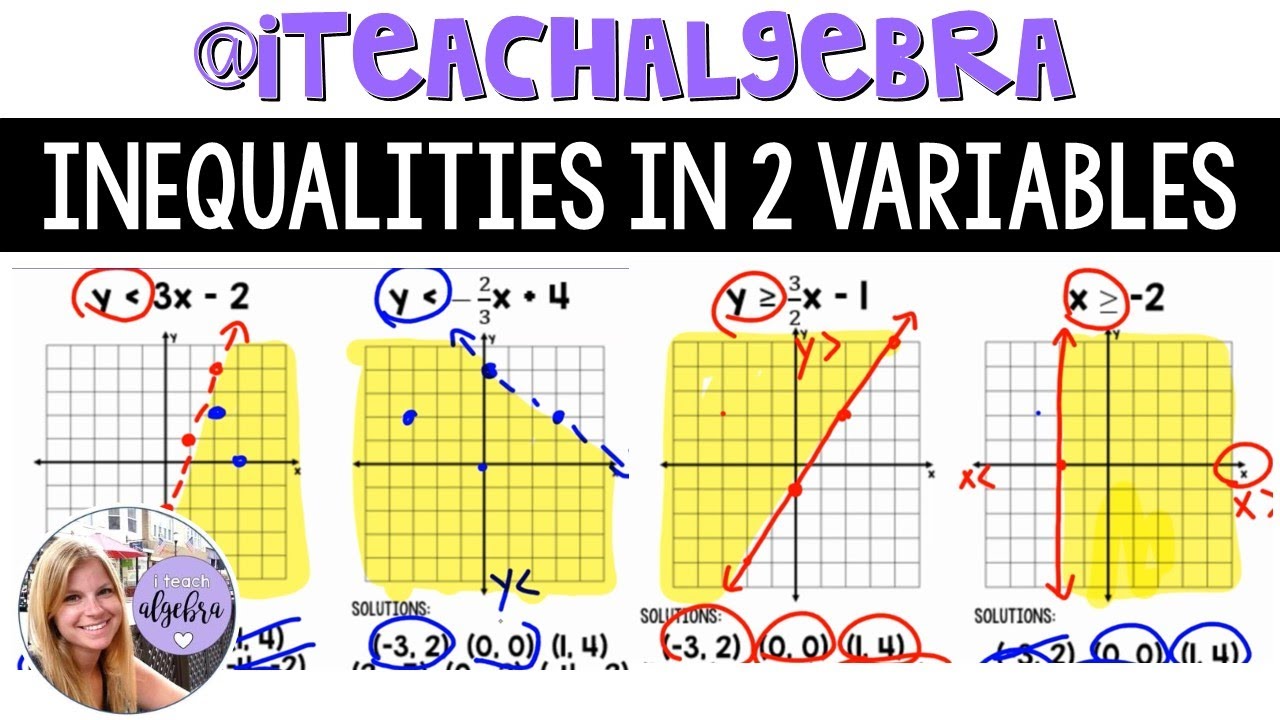
Algebra 1 - Graphing Inequalities in Two Variables

PENYAJIAN DATA DALAM BENTUK DIAGRAM GARIS || PENGOLAHAN DATA
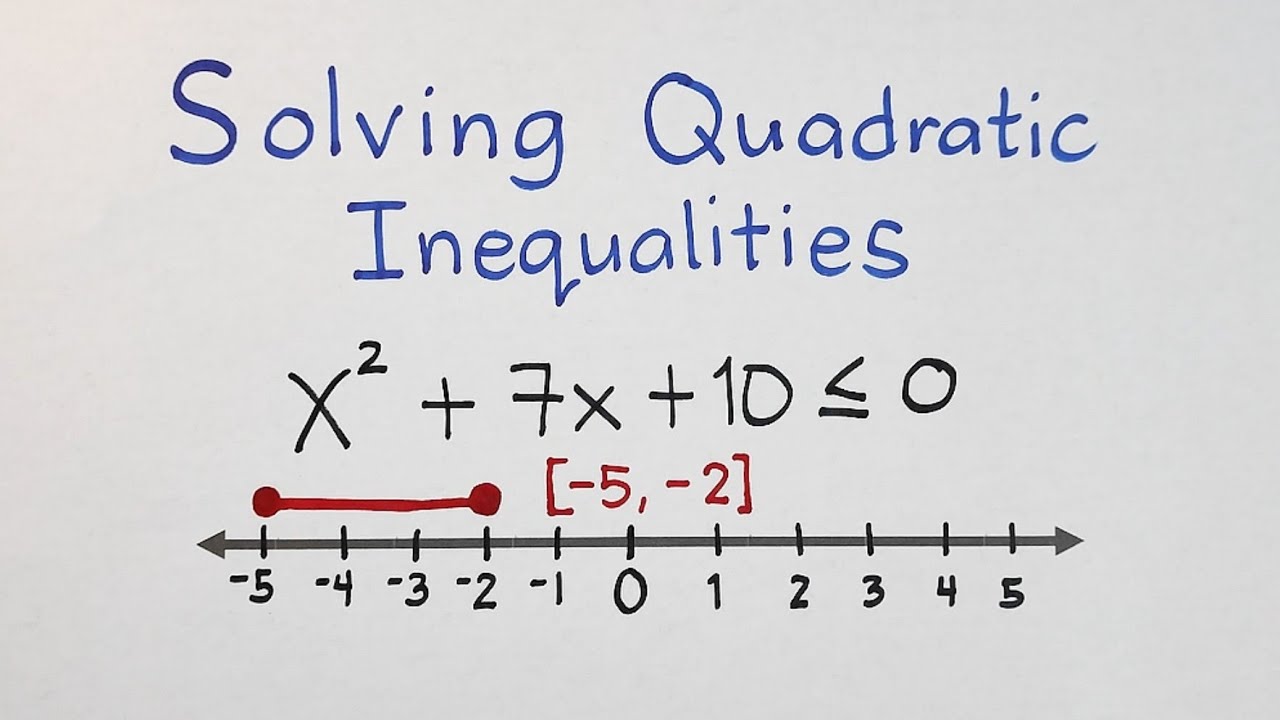
Solving Quadratic Inequalities - Grade 9 Math Teacher Gon
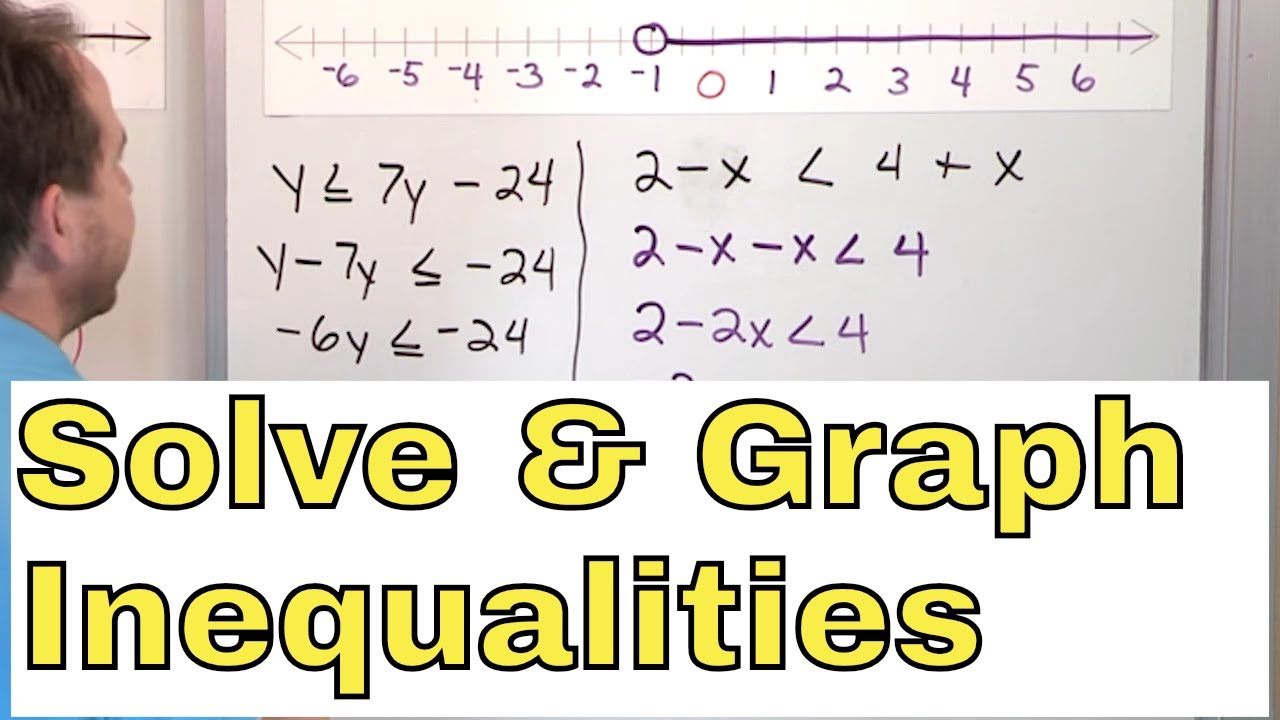
12 - Solving & Graphing Inequalities w/ One Variable in Algebra, Part 1
Graphing quadratic inequalities unit 2 day something
5.0 / 5 (0 votes)
