Dạng 1 Vận tốc của vật dao động điều hòa
Summary
TLDRThe video explains the concept of velocity and acceleration in harmonic motion. Using visual aids, it demonstrates how an object's harmonic motion can be seen as the projection of uniform circular motion onto an axis. The teacher elaborates on the velocity of harmonic oscillation, deriving its formula and discussing its properties. Key points include the relationship between velocity and acceleration, the significance of phase difference, and the concept of phase angle. The session concludes with important observations on harmonic motion, emphasizing its periodic nature and phase relationships.
Takeaways
- 😀 Harmonic motion can be visualized as the projection of uniform circular motion onto a horizontal axis.
- 📈 The speed of an object in uniform circular motion is constant in magnitude but changes in direction.
- 🔄 The velocity vector of an object in uniform circular motion is always tangent to its path.
- 📉 The velocity of harmonic motion can be derived by projecting the velocity of uniform circular motion onto the horizontal axis.
- 📐 The angle between the velocity vector and the x-axis in uniform circular motion is the phase angle of harmonic motion.
- 🔍 To find the projection, we calculate the angle formed by the velocity vector with the x-axis, which is the phase angle plus the angular displacement.
- 🔧 The formula for velocity in harmonic motion is v = -ωA sin(ωt + φ), where ω is the angular velocity, A is the amplitude, and φ is the phase angle.
- 🌀 The velocity of harmonic motion oscillates sinusoidally, indicating periodic changes in speed and direction.
- 🔄 Harmonic motion and its velocity are both described by sinusoidal functions, showing that they oscillate with the same frequency and period.
- 📊 The velocity and position of an object in harmonic motion are 90 degrees out of phase, meaning the velocity reaches its maximum when the position is zero and vice versa.
Q & A
What is the relationship between circular motion and simple harmonic motion (SHM)?
-Simple harmonic motion can be visualized as the projection of uniform circular motion onto one of the axes in the plane of motion. The projection of an object moving in a circle at constant speed onto a diameter of the circle exhibits simple harmonic motion.
How is the velocity of an object in uniform circular motion related to its velocity in SHM?
-The velocity of an object in SHM is the projection of the tangential velocity of the object in uniform circular motion onto the axis of motion. This projection changes direction but maintains a constant magnitude, resulting in simple harmonic motion.
What does the term 'omega' (ω) represent in the context of SHM and circular motion?
-In the context of SHM and circular motion, 'omega' (ω) represents the angular velocity, which is the rate of change of the angle through which the object moves in circular motion.
How can the velocity equation for SHM be derived from the properties of circular motion?
-The velocity in SHM can be derived by projecting the tangential velocity of the circular motion onto the axis of motion. The equation v = -ωA sin(ωt + φ) is obtained, where A is the amplitude, ω is the angular velocity, t is time, and φ is the phase constant.
What is the phase relationship between the displacement and velocity in SHM?
-The velocity in SHM leads the displacement by a phase of π/2 radians (90 degrees). This means when the displacement is at its maximum, the velocity is zero, and vice versa.
What is the significance of the negative sign in the velocity equation v = -ωA sin(ωt + φ)?
-The negative sign indicates that the velocity is in the opposite direction to the displacement. This reflects the fact that when the object is moving towards the equilibrium position, its velocity is directed towards the center, opposite to its displacement.
What happens to the velocity of the object in SHM at the equilibrium position?
-At the equilibrium position, the velocity of the object in SHM is at its maximum. This is because the restoring force is zero at this point, allowing the object to move at its highest speed.
How does the amplitude (A) affect the velocity in SHM?
-The amplitude (A) directly affects the maximum velocity in SHM. The larger the amplitude, the greater the maximum velocity, as v_max = ωA.
What is meant by 'phase constant' (φ) in SHM?
-The phase constant (φ) in SHM determines the initial position and velocity of the oscillating object at t = 0. It adjusts the phase of the sinusoidal function representing the motion.
Can the velocity in SHM be expressed using a cosine function?
-Yes, the velocity in SHM can be expressed using a cosine function by shifting the phase by π/2. For example, v = ωA cos(ωt + φ + π/2). This is mathematically equivalent and can sometimes simplify calculations.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

Dao động điều hòa: Chu kì. Tần số. Tần số góc. Vận tốc và gia tốc của vật dao động điều hòa

Simple Harmonic Motion(SHM) - Force, Acceleration, & Velocity at 3 Positions

Simple Harmonic Motion(SHM) - Graphs of Position, Velocity, and Acceleration
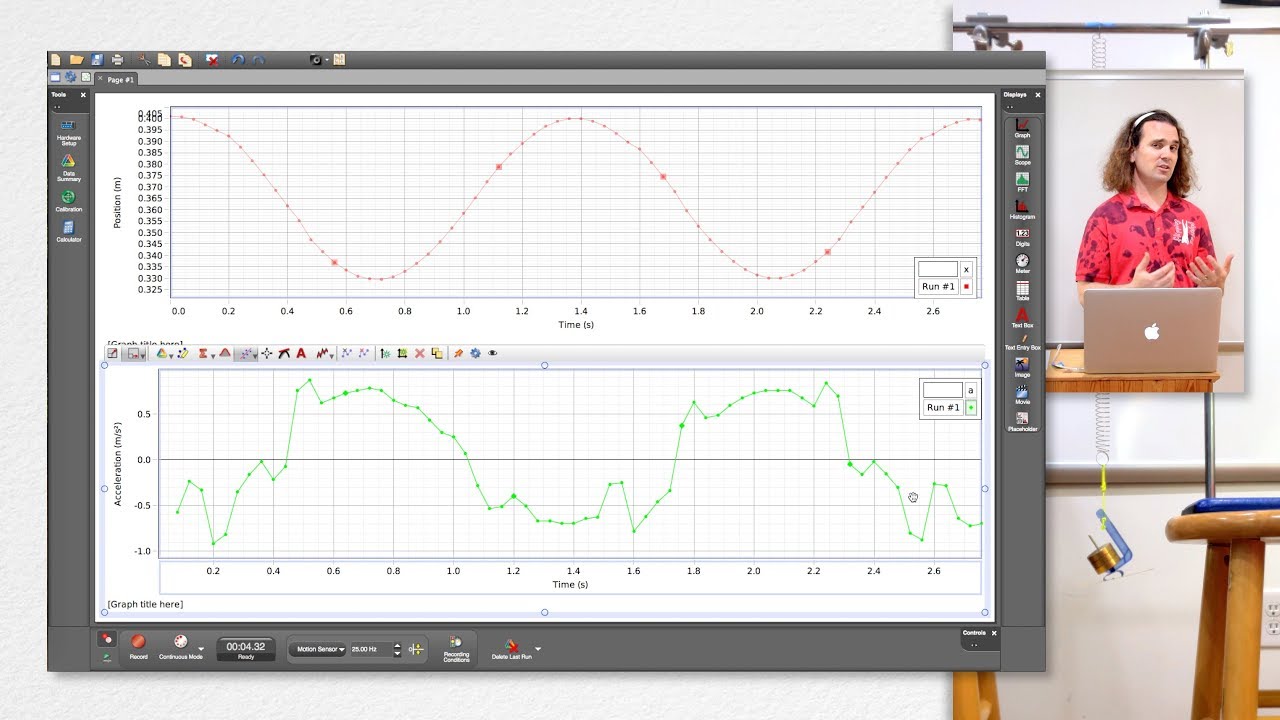
Demonstrating Position, Velocity, and Acceleration of a Mass-Spring System
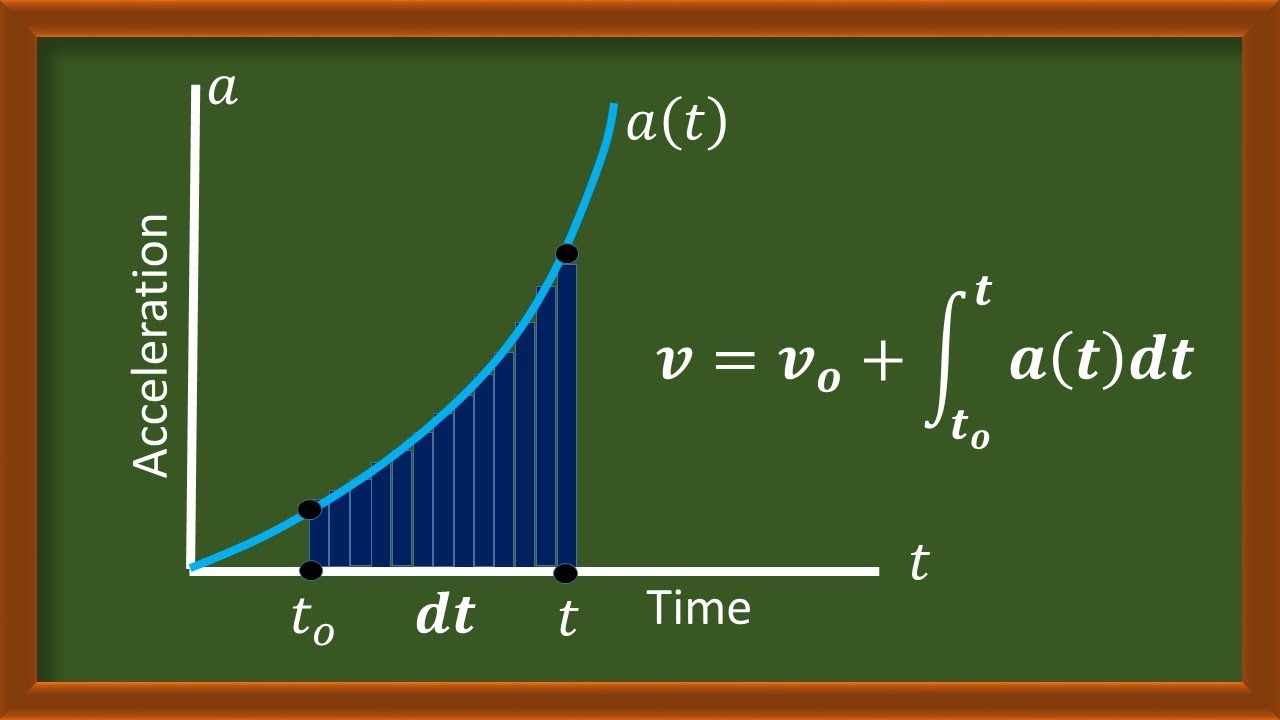
Kinematics: Acceleration Vs Time Graph

IGCSE Physics [Syllabus 1.2] Motion
5.0 / 5 (0 votes)
