dy/dx, d/dx, and dy/dt - Derivative Notations in Calculus
Summary
TLDRThis video explains the difference between 'dydx' and 'd/dx' in the context of derivatives. The speaker outlines how 'd/dx' is a command telling you to differentiate a function with respect to x, while 'dydx' is a statement indicating the derivative of y with respect to x. Examples are provided, including how to differentiate expressions like x³ + 4x² and y³ using the power rule. The video also touches on implicit differentiation, related rates problems, and how to differentiate with respect to different variables such as s and t. A pop quiz is included to reinforce the concept.
Takeaways
- 😀 The notation 'd/dx' is a command that tells you to differentiate a function with respect to x.
- 😀 'dydx' simply makes a statement about the derivative of y with respect to x.
- 😀 Differentiating a function like x³ + 4x using 'd/dx' yields 3x² + 4.
- 😀 The difference between 'd/dx' and 'dydx' is that one tells you to differentiate, while the other gives the result of the derivative.
- 😀 Implicit differentiation involves functions where the dependent variable (y) is itself a function of x, requiring an extra 'dy/dx' term.
- 😀 When differentiating a function like y³ with respect to x, you'll use the chain rule and multiply by 'dy/dx'.
- 😀 In related rates problems, you differentiate with respect to time (t), often yielding expressions like dx/dt or dy/dt.
- 😀 For example, differentiating x⁵ with respect to t gives 5x⁴ * dx/dt.
- 😀 Related rates problems are common in physics and calculus, where you analyze how multiple variables change with respect to time.
- 😀 A practical example involves differentiating r = x³ + t⁵ with respect to y, which involves the chain rule and understanding how each variable relates to y.
Q & A
What is the difference between dydx and d/dx?
-d/dx is a command that tells you to differentiate a function with respect to x, while dydx is a statement that indicates the derivative of y with respect to x.
When you see dydx, what does it represent?
-dydx represents the derivative of y with respect to x, meaning it shows how y changes as x changes.
What does the notation d/dx tell you to do?
-The notation d/dx tells you to differentiate the function inside the parentheses with respect to x.
How do you differentiate the function x³ + 4x² with respect to x?
-Using the power rule, the derivative of x³ + 4x² with respect to x is 3x² + 8x.
What is implicit differentiation?
-Implicit differentiation is when you differentiate equations where the variables are not explicitly isolated, such as when both x and y appear together in an equation.
How do you differentiate y³ with respect to x?
-To differentiate y³ with respect to x, you use the chain rule. The result is 3y² times dydx because y depends on x.
What happens when you differentiate with respect to a different variable, like s or t?
-When you differentiate with respect to a different variable, like s or t, you modify the notation to reflect that, such as dx/ds or dx/dt, and apply the corresponding derivative rules.
What does dx/dt represent?
-dx/dt represents the derivative of x with respect to time, often used in related rates problems where the rate of change of one variable depends on time.
How do you differentiate x⁴ + y³ with respect to time?
-To differentiate x⁴ + y³ with respect to time, you apply the chain rule: the derivative is 4x³ times dx/dt plus 3y² times dydt.
How do you find the derivative of r = x³ + t⁵ with respect to y?
-To differentiate r = x³ + t⁵ with respect to y, you use the chain rule. The result is 3x² times dx/dy + 5t⁴ times dt/dy.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

Complete Integration and Derivative Formulae List | Easy Trick to Learn| Engineering Mathematics 2

ĐẠO HÀM và ý nghĩa hình học (Derivative Intro) | Vật Lý Chill

dYdX on Arbitrum: How To Start Trading in Under 5 Minutes

CFA Level I Derivatives - Forward Contracts vs Futures Contracts
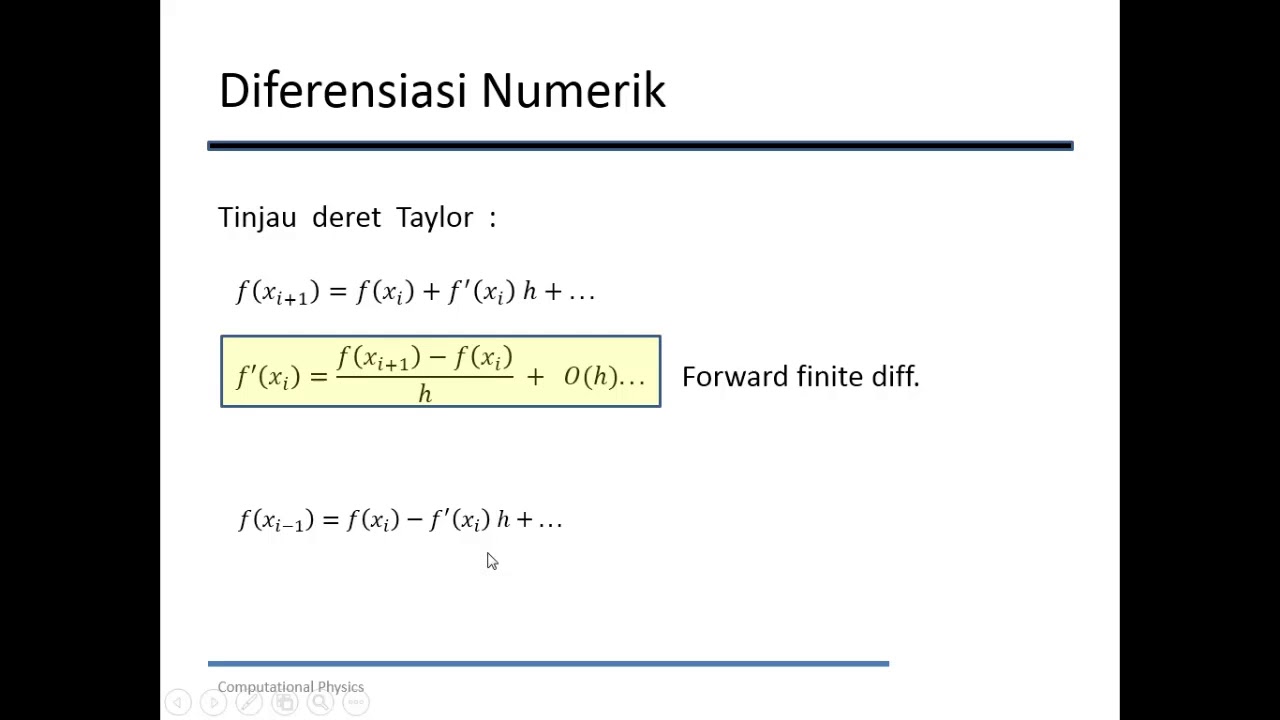
Diferensiasi Numerik
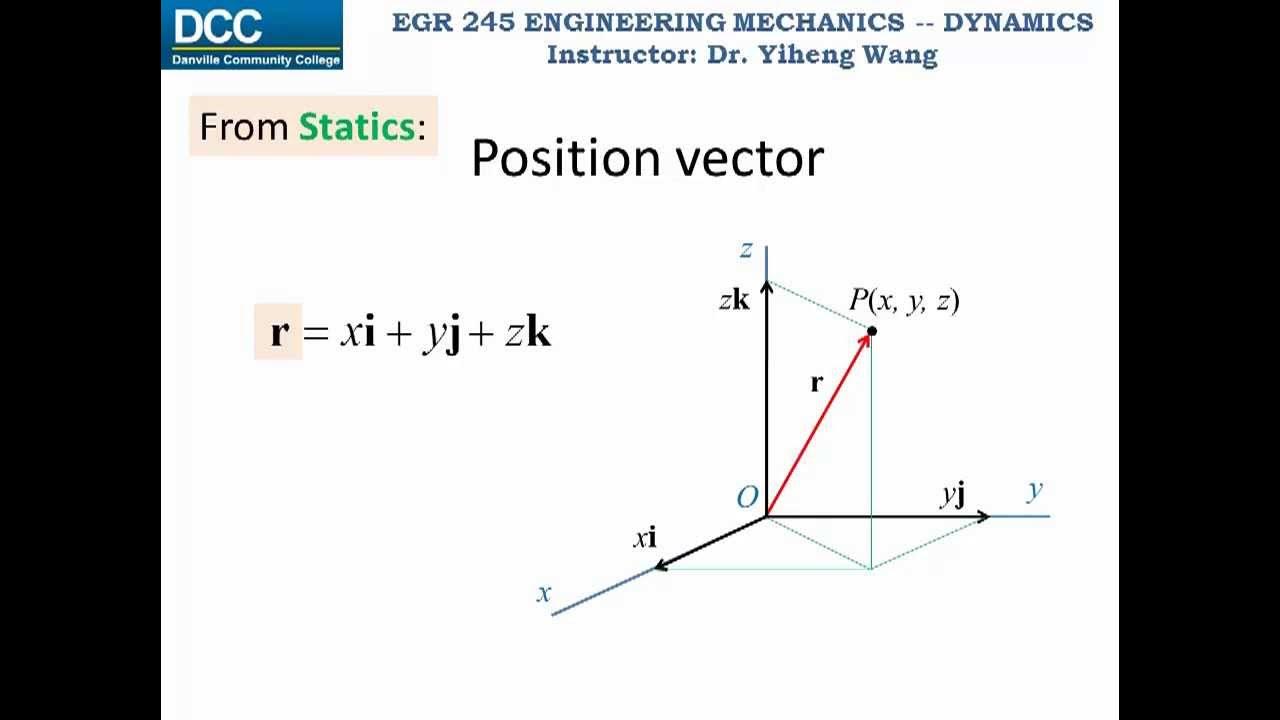
Dynamics Lecture 02: Particle kinematics, Rectilinear continuous motion part 1
5.0 / 5 (0 votes)
