How To Integrate Using U-Substitution
Summary
TLDRThis instructional video demonstrates the technique of u-substitution for integrating definite integrals. It guides viewers through identifying the u variable and its derivative, du, to simplify complex integrals. The script covers several examples, including integrating functions with trigonometric and exponential components, and emphasizes the importance of isolating dx to avoid mistakes. The video aims to help viewers master u-substitution by practicing with various problems, showcasing how to transform and integrate expressions step by step.
Takeaways
- 📚 U-substitution is a method for integrating definite integrals by identifying a suitable 'u' variable and its derivative 'du'.
- 🔍 To apply u-substitution, choose 'u' to simplify the integral by making 'du' cancel out with part of the original integral's expression.
- 📝 The process involves setting 'u' equal to an expression, finding 'du', and then solving for 'dx' in terms of 'du'.
- 🧩 Replace the original variable with 'u' and 'dx' with 'du/dx' to transform the integral into a new form that is easier to integrate.
- 📉 In the example of integrating 4x * (x^2 + 5)^3, set 'u' to x^2 + 5 and 'du' to 2x dx, and then solve for 'dx'.
- 📈 For the integral of 8 cos(4x) dx, set 'u' to 4x and 'du' to 4 dx, simplifying the integral to involve 'u' and 'du/4'.
- 🌟 The antiderivative of cosine is sine, so the integral of 8 cos(4x) dx simplifies to 2 sin(4x) + C.
- 📌 When the integral involves a complex expression, like x^3 e^{x^4}, choose 'u' to be the most complex part, x^4 in this case, to simplify the integral.
- 🔢 In the script, several examples are provided to illustrate the process, including integrals with trigonometric functions and exponentials.
- 🎯 The final step in each example is to replace 'u' back with its original expression in terms of 'x' to find the final antiderivative.
- 📝 The video emphasizes the importance of isolating 'dx' during the substitution process to avoid mistakes and simplify calculations.
Q & A
What is the main topic of the video?
-The main topic of the video is teaching how to integrate using u-substitution with a focus on definite integrals.
What is the first integral problem presented in the video?
-The first integral problem presented is to find the anti-derivative of 4x * (x^2 + 5)^3.
How does the video suggest to choose the u variable for u-substitution?
-The video suggests choosing the u variable to be something that when differentiated will cancel out terms in the integral, such as setting u = x^2 + 5 in the first example.
What is the purpose of finding du in u-substitution?
-The purpose of finding du is to replace dx with du/dx, which allows you to change the integral in terms of u and simplify the expression.
How do you solve for dx in the context of u-substitution?
-You solve for dx by isolating dx in the equation du = f(x)dx, for example, if du = 2x dx, then dx = du / 2x.
What is the antiderivative of u to the third power using the power rule?
-Using the power rule, the antiderivative of u^3 is u^4/4.
What is the second integral problem presented in the video?
-The second integral problem is to find the integration of 8 cos(4x) dx.
What is the antiderivative of cosine function?
-The antiderivative of the cosine function is sine, because the derivative of sine is cosine.
Can you give an example of a more complex integral problem from the video?
-An example of a more complex integral problem from the video is integrating x^3 e^{x^4}.
What is the final step in solving an integral using u-substitution?
-The final step in solving an integral using u-substitution is to replace the u variable back with its original expression in terms of x.
How does the video handle the case when the u variable and dx cannot be directly canceled out?
-The video demonstrates solving for x in terms of u when the u variable and dx cannot be directly canceled out, as shown in the examples involving 3x + 2 and 4x - 5.
What is the importance of isolating dx during the u-substitution process?
-Isolating dx is important to avoid mistakes and to clearly see how to replace dx with du/dx in the integral.
How does the video illustrate the process of u-substitution with trigonometric functions?
-The video illustrates the process with an example of integrating sin^4(x) cos(x) dx by setting u to the function with the higher exponent, which is sin(x) in this case.
What is the final answer for the integral of the square root of 5x + 4 as presented in the video?
-The final answer for the integral of the square root of 5x + 4 is (2/15)(5x + 4)^(3/2) + C.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

LENGKAP Integral tak tentu, integral tertentu, integral subtitusi dan integral parsial
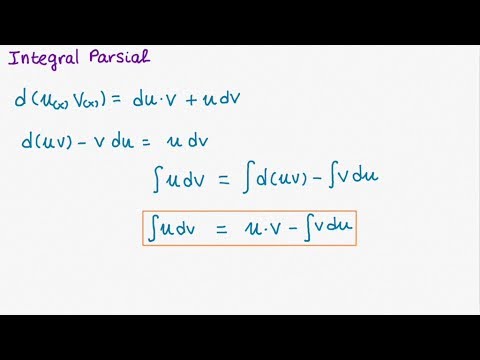
M202 Kalkulus : Metode Integral Parsial
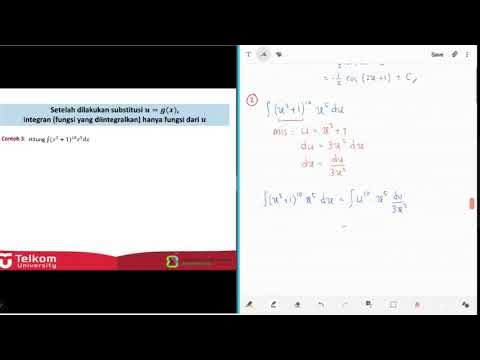
Kalkulus | Integral (Part 2) - Integral Substitusi [2]

MATEMATIKA Kelas 11 - Integral Tak Tentu | GIA Academy

INTEGRAL LIPAT 2 #KALKULUS 2

Konsep Dasar Integral Fungsi Aljabar (Integral Part 1) M4THLAB
5.0 / 5 (0 votes)
