Bakit Kailangan ng Calculus Para sa Time Rates? Differential Calculus Explained In Tagalog/Filipino
Summary
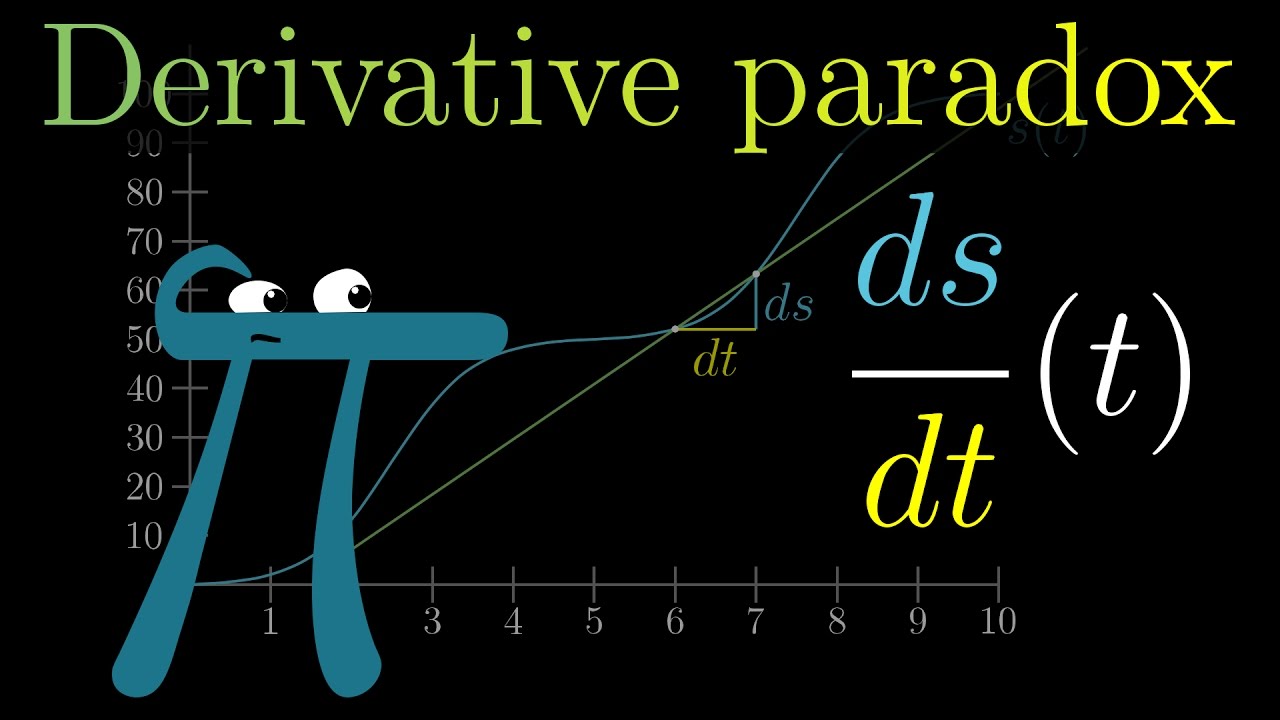
TLDRThis transcript explores the concept of derivatives and time rates, explaining how changes in one quantity, such as calories burned or steps taken, can be analyzed with respect to time. The speaker delves into the mathematical definition of derivatives as slopes, illustrating how they represent changes in variables over time. Using the example of steps and calories, the discussion highlights how rates of change, computed through derivatives, help quantify real-world phenomena like calorie expenditure in relation to physical activity. The content aims to clarify the relationship between time, reference points, and rate changes, offering insights into mathematical concepts applied to everyday actions.
Takeaways
- 😀 Derivatives represent changes in a quantity with respect to time or another reference.
- 😀 A derivative is the slope of a function, showing how one variable changes in relation to another.
- 😀 Time rates refer to how a quantity changes over time, which is a key concept in calculus and physics.
- 😀 The example of calories burned per number of steps illustrates how rates of change can be applied to real-world scenarios.
- 😀 In the example, delta x represents the change in the number of steps, while delta y represents the change in calories burned.
- 😀 The formula for the rate of change between calories burned and steps is given as delta b over delta s (change in calories over change in steps).
- 😀 The derivative of a function can be seen as how one variable changes in response to another variable over time.
- 😀 Time and reference variables change simultaneously in real-world applications, as seen in the example of steps and calories.
- 😀 The speaker emphasizes that derivatives happen at the same time in real-world calculations, such as for calories burned while walking.
- 😀 Derivatives with respect to time highlight how we can model continuous changes over time, especially in dynamic systems.
Q & A
What is the main concept discussed in the video script?
-The main concept discussed is derivatives and how they represent the rate of change of one quantity with respect to another, particularly in the context of time and reference points such as the number of steps and calories burned.
What does a derivative represent in mathematical terms?
-In mathematical terms, a derivative represents the slope of a function, which defines how a quantity changes in relation to another variable, such as time or distance.
How are derivatives applied in real-world situations in the script?
-The script applies derivatives to a real-world situation where the number of steps taken (s) is related to the number of calories burned (b), using the derivative of b with respect to s (db/ds) to measure the rate of change in calories burned as steps increase.
What is the significance of the formula 'db/ds' in the context of the script?
-'db/ds' represents the rate of change in calories burned (b) with respect to the number of steps (s). It quantifies how many calories are burned per step taken.
How does the concept of time relate to the derivative discussed in the video?
-The concept of time is related to the derivative in that the rate of change in quantities like steps and calories burned is considered with respect to time. This is expressed in terms of derivatives like ds/dt or dy/dt, which describe how quantities change over time.
What does the equation 'db/ds = 1' imply?
-'db/ds = 1' implies that for every step taken, one unit of calories is burned. This is a simplified example used to illustrate how derivatives work in a practical context.
What role does the reference point play in the concept of derivatives?
-The reference point in derivatives serves as the basis for measuring change. In the script, this could refer to time, steps, or calories burned, and the derivative measures how these quantities change relative to one another.
How does the speaker transition from discussing steps and calories to time in the video?
-The speaker transitions by discussing how changes in steps (s) and calories burned (b) are related to time, introducing time as a reference point for measuring these changes. The derivative with respect to time (ds/dt or dy/dt) is introduced as a way to understand how these quantities evolve over time.
What is meant by 'computational time rates' in the context of the script?
-Computational time rates likely refer to the rate at which computations are performed to track changes over time. This could involve using mathematical formulas or algorithms to calculate how quantities like steps and calories change as time progresses.
Why are derivatives important in understanding real-time changes?
-Derivatives are important because they allow us to quantify and understand how a quantity changes in real-time. By measuring rates of change, such as how many calories are burned per step or how steps are taken over time, derivatives provide a way to model and predict behavior in dynamic systems.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード5.0 / 5 (0 votes)