HOW TO USE THE MIDPOINT FORMULA!
Summary
TLDRThis lesson on the midpoint formula introduces the concept of a midpoint as the point that divides a line segment into two equal parts. Using examples, it demonstrates how to find the midpoint on the coordinate plane using the formula with given endpoints. The first example finds the midpoint of line segment AB, while the second example involves determining the unknown endpoint when given the midpoint and one endpoint. Additionally, a visual method using slope is introduced to further understand the relationship between points, enhancing comprehension of the midpoint concept.
Takeaways
- 😀 The midpoint of a line segment is the point that divides the segment into two equal halves.
- 😀 The midpoint formula for two points (x1, y1) and (x2, y2) is: Midpoint = ((x1 + x2) / 2, (y1 + y2) / 2).
- 😀 The midpoint is located exactly halfway between the two endpoints of a line segment.
- 😀 Example 1 demonstrates finding the midpoint of points A(7, 3) and B(5, 5), yielding the midpoint (6, 4).
- 😀 In the first example, the x-coordinates and y-coordinates of the endpoints are averaged to find the midpoint.
- 😀 Example 2 involves using the midpoint formula to find a missing endpoint when the midpoint and one endpoint are given.
- 😀 For Example 2, given midpoint M(-3, -4) and endpoint T(-6, ?), solving the midpoint formula gives the missing endpoint N(0, 1).
- 😀 To solve for the missing x-coordinate in Example 2, the equation (-6 + x) / 2 = -3 is solved for x = 0.
- 😀 For the missing y-coordinate in Example 2, the equation (-9 + y) / 2 = -4 is solved for y = 1.
- 😀 The visual method of using slope helps to verify the coordinates by plotting points and repeating slopes from the midpoint.
Q & A
What is a midpoint?
-A midpoint is the point that divides a line segment into two equal halves.
How do you calculate the midpoint of a line segment?
-To calculate the midpoint of a line segment connecting two points (x1, y1) and (x2, y2), use the midpoint formula: M = ((x1 + x2) / 2, (y1 + y2) / 2).
What are the coordinates of points A and B in Example 1?
-In Example 1, the coordinates of point A are (7, 3) and point B are (5, 5).
What is the midpoint of line segment AB from Example 1?
-The midpoint of line segment AB, with points A(7, 3) and B(5, 5), is M(6, 4).
What is the significance of congruent halves in relation to midpoints?
-Congruent halves refer to the two segments created by the midpoint, indicating that both segments are equal in length.
What information is given in Example 2 about the midpoint and endpoint?
-Example 2 provides the midpoint M(-3, -4) and one endpoint T(-6, 9) to find the other endpoint N.
How do you determine the x-coordinate of endpoint N in Example 2?
-To find the x-coordinate of endpoint N, set up the equation -6 + x = -6, which solves to x = 0.
What is the y-coordinate of endpoint N in Example 2?
-The y-coordinate of endpoint N is found by solving -9 + y = -8, which gives y = 1.
How can you visually verify the position of endpoint N?
-You can visually verify endpoint N by plotting it and constructing the line segment TN, ensuring that the midpoint M is correctly located.
Where can viewers find more math tips related to this topic?
-Viewers can follow the Instagram account @mashupmaath for free daily math tips and infographics.
Outlines

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードMindmap

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードKeywords

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードHighlights

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレードTranscripts

このセクションは有料ユーザー限定です。 アクセスするには、アップグレードをお願いします。
今すぐアップグレード関連動画をさらに表示

GEO.1.2

PONTO MÉDIO DE UM SEGMENTO | Geometria analítica | FÓRMULA E EXERCÍCIOS

Midpoint of a Line Segment (2nd) Second Quarter Grade 8 Matatag Revised K-12 Tagalog Math Tutorial
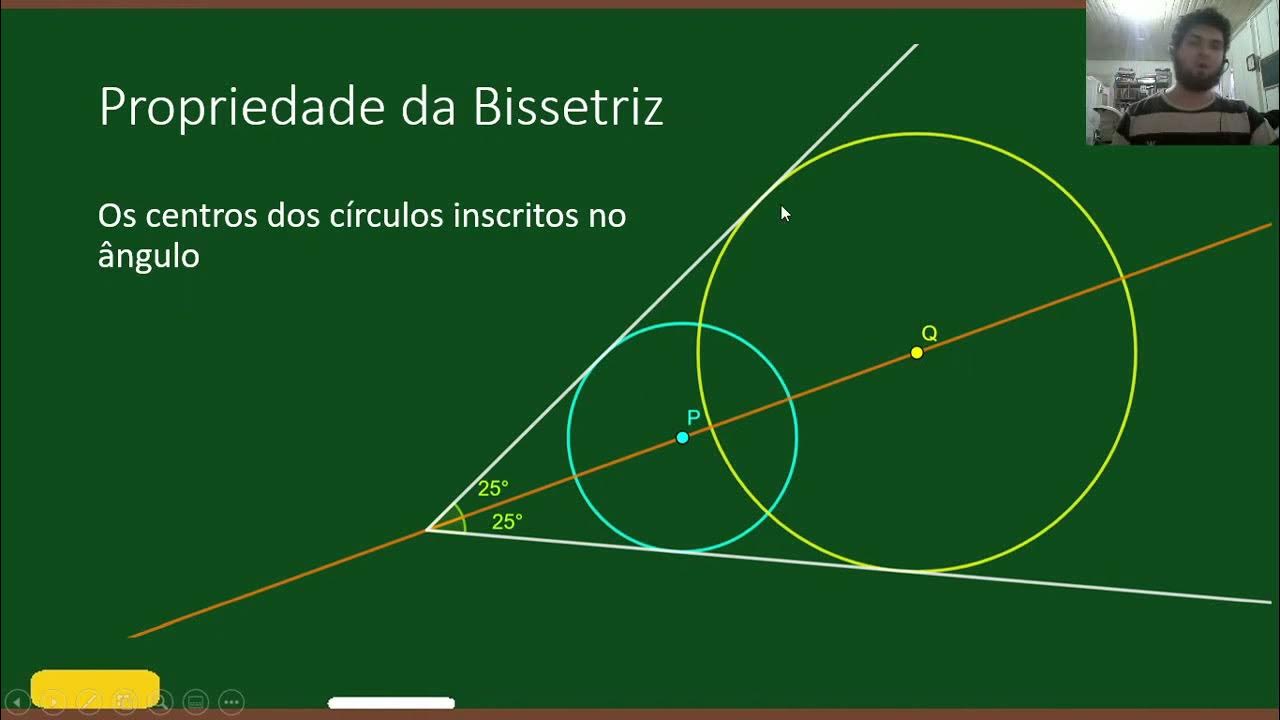
Mediatriz e Bissetriz

VEKTORRECHNUNG Mittelpunkt berechnen – Grundlagen, Mitte von zwei Vektoren
Perpendicular Bisector Finding the Equation
5.0 / 5 (0 votes)
