Adding and subtracting polynomials | Algebra Basics | Khan Academy
Summary
TLDRIn this video, the process of simplifying a polynomial expression is demonstrated. The problem involves the expression '16x + 14 - (3x^2 + x - 9)', and the explanation begins by distributing the negative sign across the terms within the parentheses. The next step involves combining like terms: the quadratic term, linear terms, and constant terms. After simplifying, the final expression is '-3x^2 + 15x + 23'. The video effectively breaks down the steps of polynomial simplification, making it easier to understand for learners.
Takeaways
- 😀 The expression to simplify is 16x + 14 - (3x^2 + x - 9).
- 😀 Begin by removing the parentheses without altering the order of operations.
- 😀 Distribute the negative sign to each term inside the parentheses: -3x^2, -x, and +9.
- 😀 The highest degree term is -3x^2, as x is raised to the second power.
- 😀 Combine the x terms: 16x - x = 15x.
- 😀 The constant terms are 14 and 9, which sum to 23.
- 😀 The simplified polynomial after combining like terms is -3x^2 + 15x + 23.
- 😀 When simplifying, always ensure to distribute signs correctly, especially when subtracting terms.
- 😀 The order of operations is crucial in simplification, especially when parentheses are involved.
- 😀 The goal is to rewrite the expression as a simplified polynomial by combining like terms.
- 😀 The final result shows that the expression simplifies into a quadratic form with no like terms left.
Q & A
What is the first step in simplifying the expression 16x + 14 - (3x^2 + x - 9)?
-The first step is to remove the parentheses and distribute the negative sign across the terms inside, which changes the signs of the terms. The expression becomes 16x + 14 - 3x^2 - x + 9.
What happens to the term 3x^2 when the negative sign is distributed?
-When the negative sign is distributed, the positive 3x^2 becomes -3x^2.
How do you simplify the linear terms (16x and -x)?
-You combine the like terms 16x and -x by subtracting 1 from 16, which gives 15x.
What happens when you combine the constant terms (14 and 9)?
-When you combine the constants 14 and 9, you get 23.
What is the highest degree term in the expression after simplifying?
-The highest degree term is -3x^2, which is the second-degree term because x is raised to the power of 2.
What is the simplified polynomial after combining all terms?
-The simplified polynomial is -3x^2 + 15x + 23.
Why does the expression 14 + 9 become 23 instead of another number?
-The expression 14 + 9 is a simple addition of constants, and the sum of 14 and 9 is 23.
Why do we distribute the negative sign to each term in the parentheses?
-We distribute the negative sign to each term to ensure that the signs of the terms inside the parentheses are properly changed, following the rules of algebra.
Is the order of operations important when simplifying this expression?
-Yes, the order of operations is important. First, you simplify inside the parentheses (if needed) and then handle the distribution of the negative sign before combining like terms.
What do we call terms that have the same degree, like 16x and -x?
-Terms that have the same degree are called 'like terms.' In this case, both 16x and -x are linear terms (degree 1), so they can be combined.
Outlines

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraMindmap

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraKeywords

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraHighlights

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraTranscripts

Esta sección está disponible solo para usuarios con suscripción. Por favor, mejora tu plan para acceder a esta parte.
Mejorar ahoraVer Más Videos Relacionados
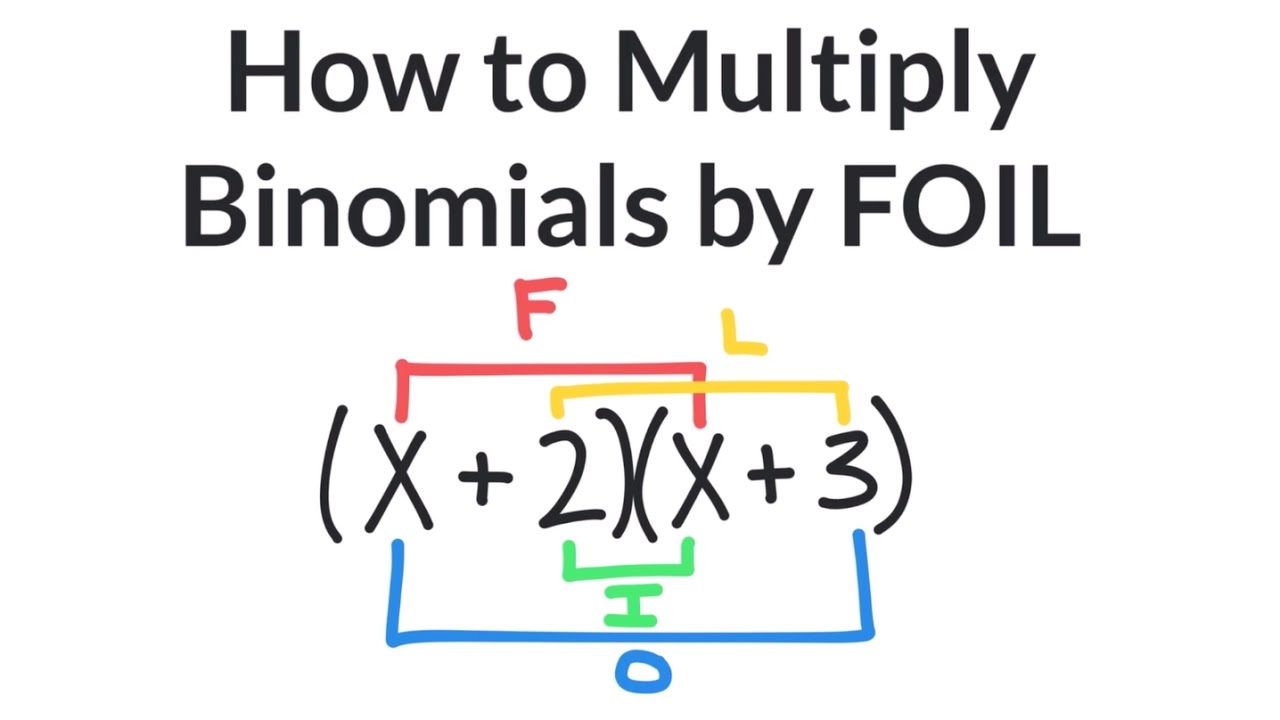
How to Multiply Binomials Using FOIL
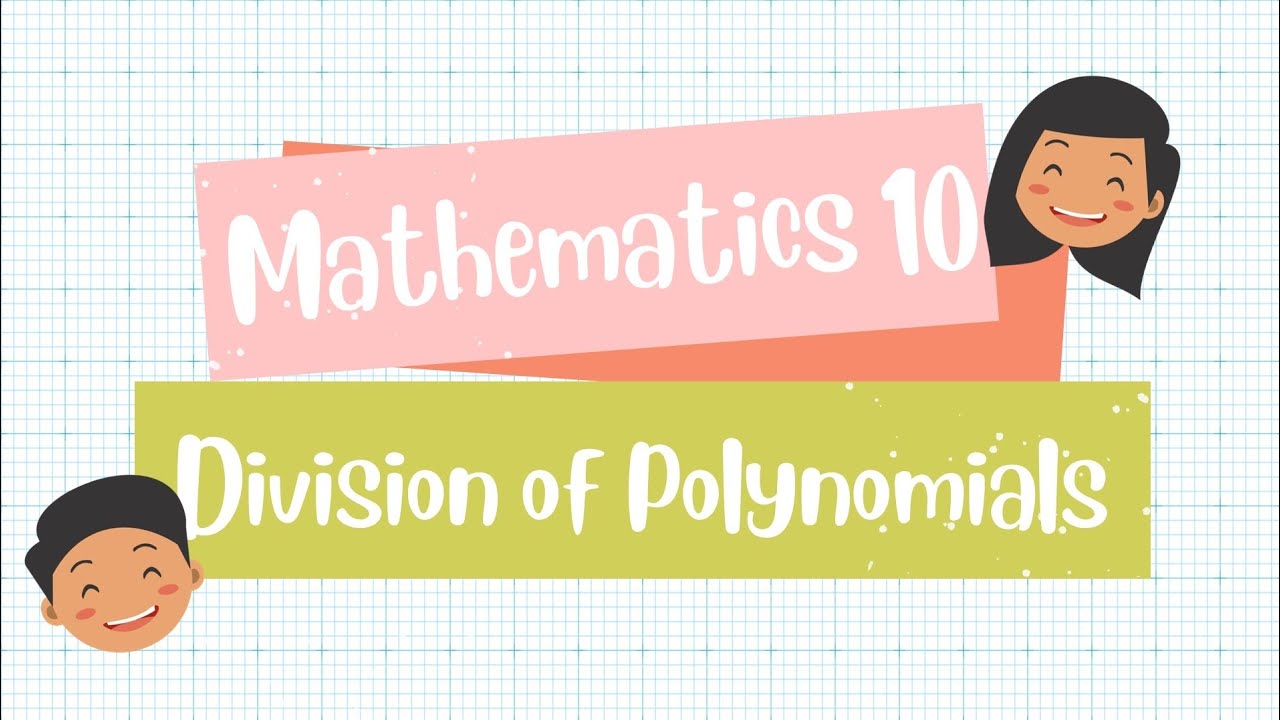
Division of Polynomials (Long Division of Polynomials)
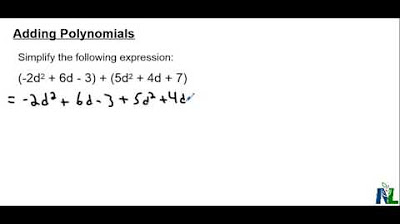
Adding Polynomials Horizontally
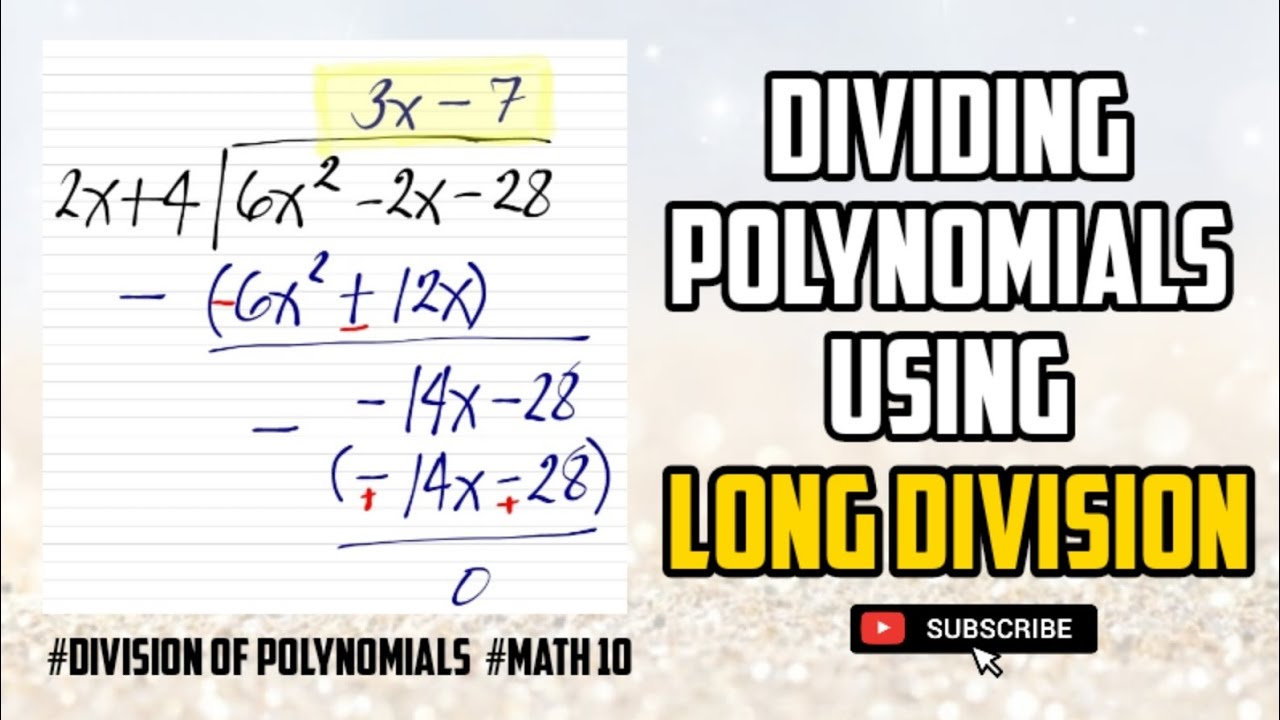
How to Divide Polynomials Using LONG DIVISION | Math 10
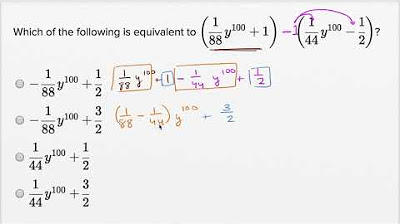
Operations with polynomials — Basic example | Math | SAT | Khan Academy
Illustrate Polynomial Functions | Second Quarter | Grade 10 MELC
5.0 / 5 (0 votes)
