FACTORING DIFFERENCE OF TWO SQUARES || GRADE 8 MATHEMATICS Q1
Summary
TLDRThis educational video teaches viewers how to identify perfect squares and the concept of a difference of two squares in algebra. It demonstrates the process of factoring such expressions by providing step-by-step examples, including rewriting perfect squares in exponential form and applying the formula for factoring differences of squares. The video aims to clarify these mathematical concepts with clear explanations and practical demonstrations, ensuring that viewers can apply these techniques in their own problem-solving.
Takeaways
- 📚 The video aims to teach viewers how to identify if an expression is a difference of two squares and how to factor it completely.
- 🔍 It starts by explaining the concept of perfect squares and how to determine if a number is a perfect square by multiplying a number by itself.
- 📉 The script provides examples of numbers that are and are not perfect squares, such as 25, 81, 144, and 100 being perfect squares, while others like 4 and 88 are not.
- 📝 Perfect squares are then rewritten in exponential form, for instance, 25 is rewritten as 5 squared (5^2).
- 📈 The script introduces the formula for a difference of two squares as x^2 - y^2 and explains it can be factored into (x + y)(x - y).
- 📚 It clarifies that the difference of two squares is the product of the sum and difference of the terms x and y.
- 📝 The video provides a step-by-step method to factor expressions like x^2 - y^2 by identifying and using the square roots of x and y.
- 🔢 Examples are given to demonstrate the factoring process, such as 9x^2 - 100, which is factored into (3x + 10)(3x - 10).
- 📉 The video also covers how to handle expressions with variables and exponents, like m^2 - 4, which is factored into (3m + 2)(3m - 2).
- ❗ It emphasizes the importance of correctly identifying the square roots and the sum and difference of the terms for accurate factoring.
- 👍 The video concludes by encouraging viewers to like, subscribe, and hit the bell button for more educational content.
Q & A
What is the main topic of the video?
-The main topic of the video is to identify whether an expression is a difference of two squares and to factor the difference of two squares completely.
What is a perfect square and how can you identify it?
-A perfect square is a number that can be expressed as the product of an integer with itself. You can identify a perfect square by checking if the square root of the number is an integer.
How is the number 25 expressed in exponential form?
-The number 25 is expressed in exponential form as 5 squared, or 5^2, because 5 multiplied by 5 equals 25.
What is the difference of two squares in algebraic terms?
-In algebraic terms, the difference of two squares is expressed as x^2 - y^2, where x and y are real numbers or algebraic expressions.
What is the factored form of the difference of two squares?
-The factored form of the difference of two squares is the product of the sum and difference of the terms, which is (x + y)(x - y).
Can you provide an example of factoring a difference of two squares from the video?
-An example from the video is factoring 9x^2 - 100, which can be rewritten as (3x)^2 - 10^2 and then factored into 3x + 10 and 3x - 10.
What is the process for rewriting a perfect square in exponential form?
-The process involves taking the square root of the perfect square to find the base number and then expressing it as that base number squared, or using the exponent 2.
How does the video demonstrate the factoring of non-perfect squares?
-The video demonstrates that non-perfect squares cannot be factored as a difference of two squares because they do not have an integer square root.
What is the factored form of the expression 4x^2 - 81 according to the video?
-The factored form of the expression 4x^2 - 81 is (2x + 9)(2x - 9), after rewriting it as a difference of two squares.
Can the expression -49x^8 + 25 be factored as a difference of two squares?
-Yes, the expression -49x^8 + 25 can be factored as a difference of two squares, resulting in (5 - 7x^4)(5 + 7x^4).
What is the importance of recognizing a difference of two squares in algebra?
-Recognizing a difference of two squares is important in algebra because it allows for the simplification and factoring of expressions, which can be useful in solving equations and other mathematical problems.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

FATORAÇÃO RESUMÃO | FATOR COMUM , AGRUPAMENTO, TRINÔMIO QUADRADO E DIFERENÇA ENTRE DOIS QUADRADOS
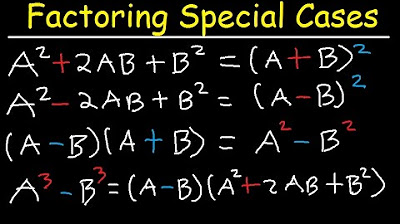
Factoring Binomials & Trinomials - Special Cases

NÚMEROS QUADRADOS PERFEITOS - 8º ANO

Grade 8 Math Q1 Ep2: Factoring Special Products
Solving Quadratic Equations by Extracting the Square Roots by @MathTeacherGon

Factorización por Diferencia de cuadrados. Con fracciones | Video 2 de 3.
5.0 / 5 (0 votes)
