Radial wave function, radial probability, radial probability distribution graph

Summary

In this video, the concept of radial wave functions and radial probability functions in quantum mechanics is explained. The radial wave function describes the electron's probability distribution based on its distance from the nucleus, with variations for different orbitals such as 1s, 2s, and 2p. The discussion covers how radial wave functions change with distance and how they are linked to energy levels. The video also explores radial probability densities and how they help determine the likelihood of finding an electron in a specific region, illustrating these concepts through graphical representations and mathematical formulas.

Takeaways

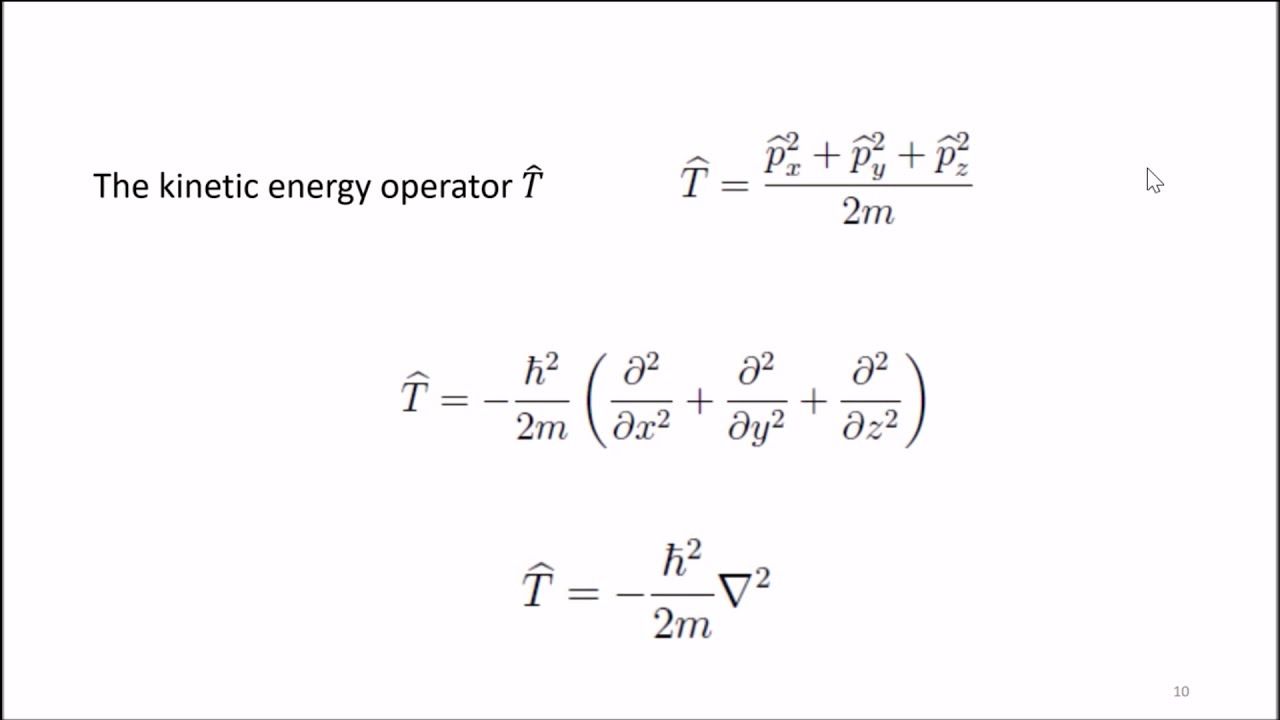
• 😀 The wave function from the Schrödinger equation provides two main results: the wave function (ψ) and energy (E).
• 😀 The wave function can be separated into two parts: the radial wave function, which depends on distance (r), and the angular wave function, which depends on quantum numbers l and m.
• 😀 Radial wave functions represent the relationship between the electron's distance from the nucleus and the probability of finding the electron at that distance.
• 😀 For 1s orbital, the radial wave function is highest near the nucleus and decreases as the distance increases.
• 😀 The 2s orbital graph shows a radial wave function that first decreases, then crosses zero (a node) before increasing again, indicating regions where the electron cannot be found.
• 😀 A node is a point where the probability of finding an electron is zero, and the graph of the radial wave function crosses from positive to negative at these points.
• 😀 The 3s orbital also exhibits nodes and its radial wave function graph is similar to that of 2s but with additional features due to its higher energy level.
• 😀 Radial probability density is represented by r² (the square of the radial wave function), which shows the probability density of finding an electron at a given distance.
• 😀 Radial probability distribution takes into account the volume of a spherical shell, giving a probability of finding an electron in a small region of space (dr).
• 😀 The radial probability distribution graph for 1s shows a peak at approximately 0.529 Å (Bohr's radius) and decreases thereafter, with a non-zero probability of finding an electron even at infinite distances.

Q & A

What are the two parts that the wave function 's' is separated into?

The wave function 's' is separated into two parts: the radial wave function and the angular wave function.

What is the primary difference between the radial wave function and the angular wave function?

The radial wave function depends only on the distance from the nucleus (r), while the angular wave function depends on the quantum numbers 'l' (orbital angular momentum) and 'm' (magnetic quantum number).

What happens to the radial wave function as the distance from the nucleus increases?

As the distance from the nucleus increases, the radial wave function decreases.

What is a node in the context of the radial wave function?

A node is a point where the probability of finding an electron is zero. It is the point where the radial wave function crosses from positive to negative or vice versa.

What is the difference between the radial wave function and the radial probability density?

The radial wave function is the function representing the wave's amplitude, while the radial probability density is the square of the radial wave function, representing the probability of finding an electron at a certain distance from the nucleus.

How is the radial probability distribution function calculated?

The radial probability distribution function is calculated by multiplying the radial probability density (r²) by the volume of a spherical shell (dr), which gives the probability of finding an electron in a small region around the nucleus.

What does the graph for radial probability distribution show for the 1s orbital?

For the 1s orbital, the radial probability distribution increases as the distance from the nucleus increases, reaches a maximum, and then decreases. It never reaches zero, meaning there is always some probability of finding the electron at any distance.

How does the radial probability distribution for the 2s orbital differ from that of the 1s orbital?

The radial probability distribution for the 2s orbital has two maxima, with one at a smaller radius and the other at a larger radius. There is a node between these maxima where the probability of finding an electron is zero, which is not present in the 1s orbital.

Why does the 2s orbital spend more time near the nucleus than the 2p orbital?

The 2s orbital spends more time near the nucleus than the 2p orbital because the 2s orbital has a smaller second maximum (closer to the nucleus) compared to the 2p orbital, which places a greater probability closer to the nucleus.

What is the significance of the distance of maximum probability for the 2s and 2p orbitals?

For the 2s orbital, the maximum probability appears at approximately 0.8 Å, and for the 2p orbital, the maximum probability appears at approximately 2.1 Å. These distances are larger than that for the 1s orbital, indicating that electrons in 2s and 2p orbitals are, on average, farther from the nucleus than those in 1s orbitals.