Geometria analítica - Inclinação e coeficiente angular Equação da reta sabendo ponto e a declividade
Summary
TLDRThis video script focuses on teaching the concept of the slope (or coefficient angular) and how to calculate the equation of a line in geometry. It explains how to find the slope (m) using two methods: from the graph, based on the angle of inclination, and from two given points on the line. The script also covers how to determine the equation of a line when provided with a point and the slope, showing step-by-step solutions to practical examples. Viewers are encouraged to watch the full playlist for further learning on analytical geometry.
Takeaways
- 😀 The coefficient 'm' represents the angular coefficient and is crucial for calculating the slope of a line.
- 😀 The slope can be calculated in two ways: using the angle of inclination or by applying the formula with two points on the line.
- 😀 The line equation in slope-intercept form is represented as Y = mX + b, where 'm' is the slope and 'b' is the y-intercept.
- 😀 The angle of inclination increases counterclockwise as you move along the line.
- 😀 The y-intercept ('b') is the point where the line crosses the y-axis.
- 😀 The angular coefficient 'm' can also be found by taking the tangent of the angle formed between the line and the x-axis.
- 😀 To find the slope between two points, use the formula: (Y2 - Y1) / (X2 - X1).
- 😀 If given a point and an angle, the slope can be directly calculated using the tangent of the given angle.
- 😀 For a 45° angle, the tangent value is 1, meaning the slope of the line is 1.
- 😀 The equation of a line passing through a known point with a known slope can be written as (Y - Y1) = m(X - X1), where (X1, Y1) is the known point.
Q & A
What is the coefficient angular (m) in the context of the script?
-The coefficient angular, denoted by 'm', represents the slope or inclination of a line. It describes how steep a line is relative to the x-axis.
How can you find the coefficient angular (m) using the tangent of an angle?
-The coefficient angular (m) can be found by using the tangent of the angle of inclination formed between the line and the x-axis. Specifically, m equals the tangent of that angle.
What does the coefficient linear (n) represent in a line's equation?
-The coefficient linear (n) is the y-intercept of the line, which is the point where the line crosses the y-axis.
What is the general equation of a line when you know a point and the slope?
-The general equation of the line is given by the formula: Y - y1 = m(x - x1), where m is the slope and (x1, y1) is a known point on the line.
How do you calculate the coefficient angular (m) when two points are given?
-To calculate m using two points, subtract the y-values (y2 - y1) and the x-values (x2 - x1), then divide the difference in y by the difference in x: m = (y2 - y1) / (x2 - x1).
What happens if you are given a single point and an angle instead of two points?
-If you are given a single point and an angle, you can find the coefficient angular by using the tangent of the given angle of inclination, with m equal to the tangent of that angle.
What is the importance of knowing notable angles like 45 degrees in this context?
-Notable angles, such as 45 degrees, are important because their tangents are well-known values. For instance, the tangent of 45 degrees is 1, making it easier to calculate the slope of a line with that inclination.
How can you determine the equation of a line if the slope and a point on the line are known?
-If the slope (m) and a point (x1, y1) on the line are known, you can substitute these values into the point-slope formula: Y - y1 = m(x - x1), and then simplify to get the equation of the line.
What does the script suggest about learning geometry analytically?
-The script encourages watching additional lessons in the playlist for a deeper understanding of analytical geometry, especially if you find concepts like the coefficient angular challenging.
What is the general procedure when solving for the equation of a line given a point and slope?
-The general procedure involves substituting the given point and slope into the point-slope equation of the line, simplifying the equation, and if needed, converting it to the general form of the line equation.
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen
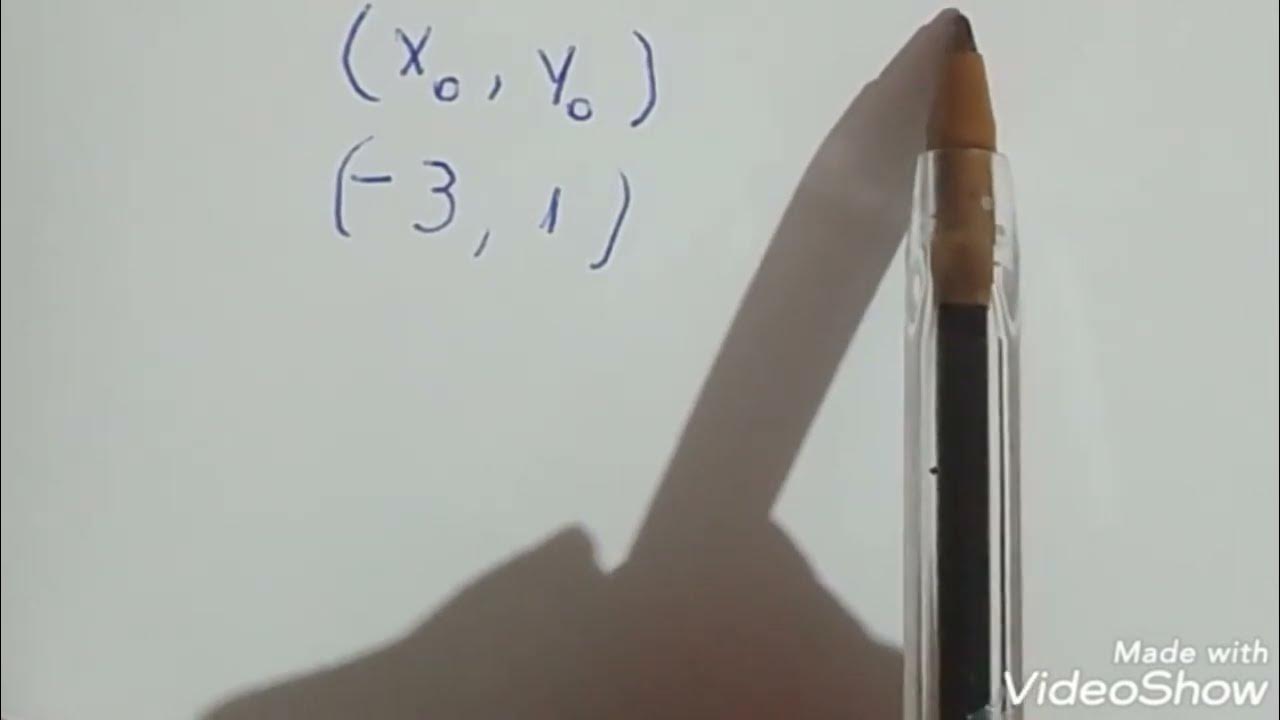
Questão de Geometria Analítica - equação da reta

Taxa de Variação da Função Afim

Introduction to point-slope form | Algebra I | Khan Academy

Pengertian Kemiringan Hal 235-238 Bab 5 Persamaan Garis Lurus Kelas 8 Kurikulum Merdeka Belajar
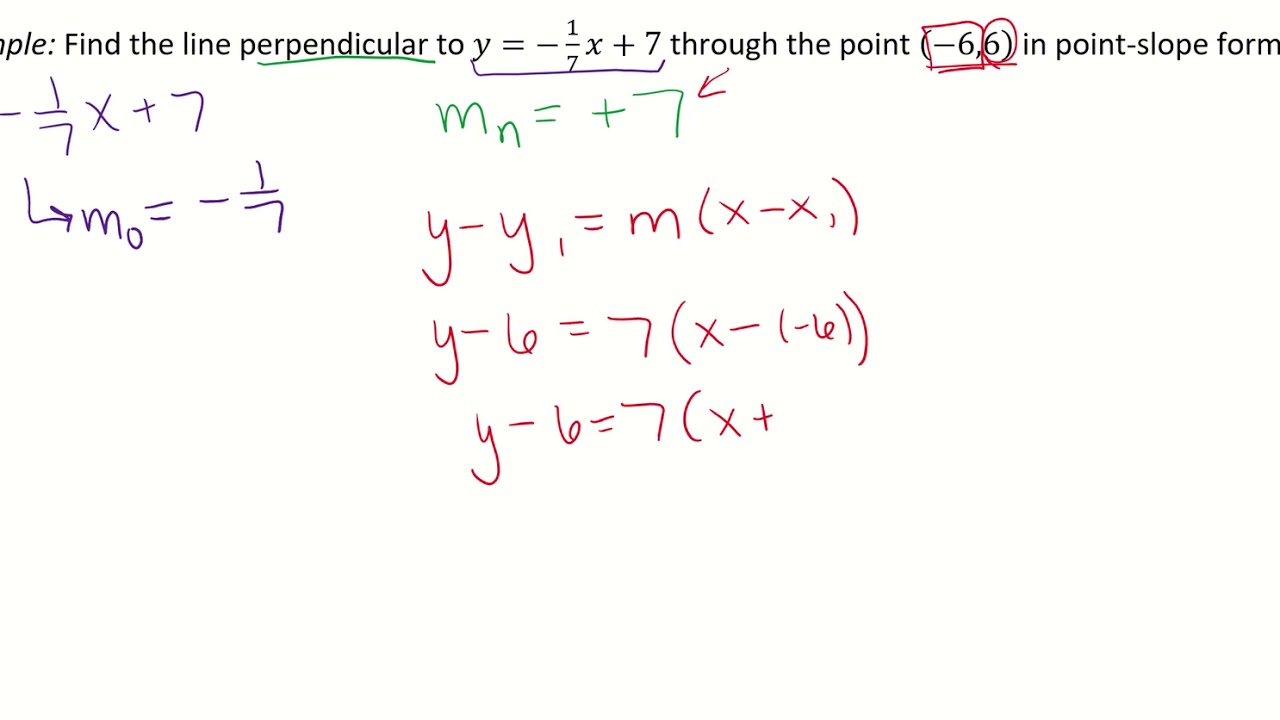
Lesson 4-1, Video 5; Perpendicular Line 2

FUNÇÃO DO 1º GRAU: DEFINIÇÃO E GRÁFICO
5.0 / 5 (0 votes)
