Matematika SMA - Trigonometri (6) - Koordinat Polar, Koordinat Kutub dan Kartesius (A)
Summary
TLDRIn this engaging video, Chalid explores the concept of polar coordinates in trigonometry, explaining how to convert between polar and Cartesian systems. He provides clear examples to illustrate the calculations for finding coordinates in both systems, including specific problems involving radius and angles. The lesson culminates in a formula for calculating the distance between two polar points, enhancing viewers' understanding of trigonometric relationships. With practical exercises and visual aids, this video is a valuable resource for students seeking to master the fundamentals of polar coordinates.
Takeaways
- 😀 Polar coordinates are expressed as (r, α), where r is the radius and α is the angle from the x-axis.
- 😀 Cartesian coordinates are expressed as (x, y), representing the standard coordinate system.
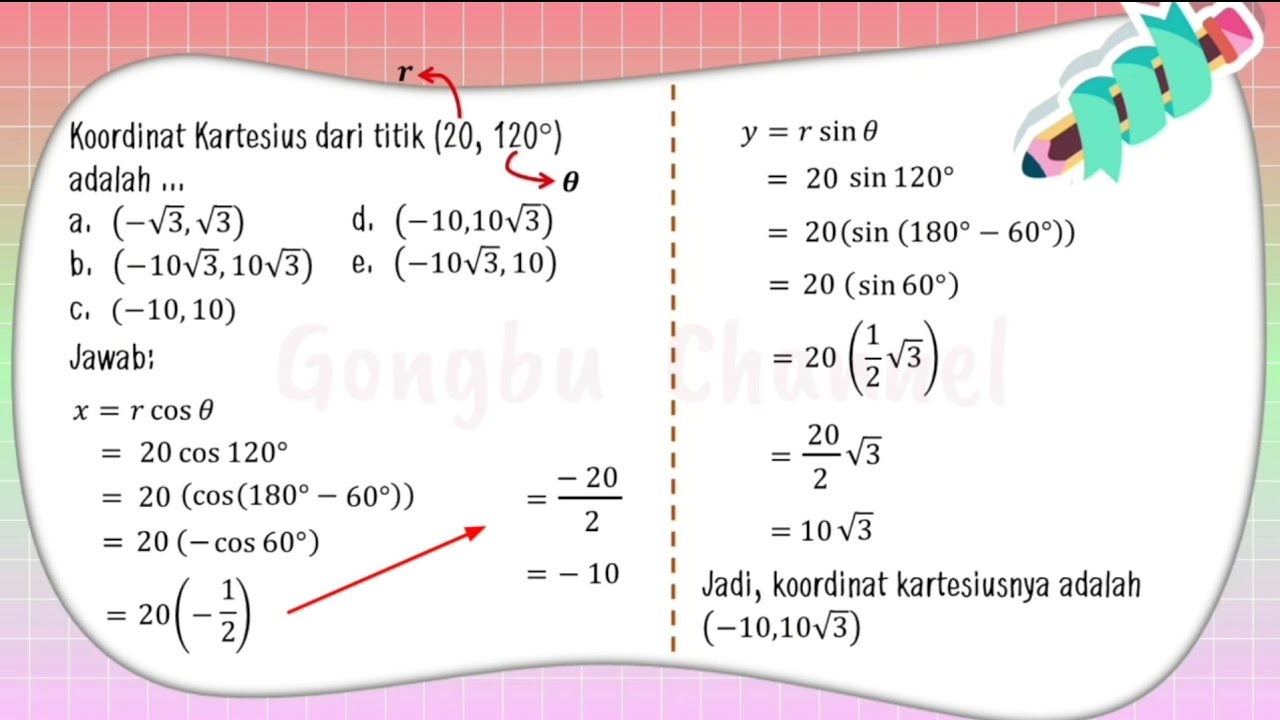
- 😀 The conversion from polar to Cartesian coordinates uses the formulas: x = r * cos(α) and y = r * sin(α).
- 😀 The conversion from Cartesian to polar coordinates uses the formulas: r = √(x² + y²) and α = tan⁻¹(y/x), with adjustments for the quadrant.
- 😀 Example 1 demonstrates how to convert polar coordinates (12, 45°) to Cartesian coordinates, resulting in (6√2, 6√2).
- 😀 Example 2 shows how to convert Cartesian coordinates (-4, 4) to polar coordinates, yielding (4√2, 135°).
- 😀 Example 3 illustrates the conversion of Cartesian coordinates (√3, -1) to polar coordinates, resulting in (2, 330°).
- 😀 The quadrant of the angle α is determined by the signs of x and y values, affecting the calculation of the angle.
- 😀 The distance between two points in polar coordinates can be calculated using the formula: P = √(R1² + R2² - 2*R1*R2*cos(α2 - α1).
- 😀 Viewers are encouraged to engage with the content by liking, sharing, and subscribing for more educational resources.
Q & A
What are polar coordinates?
-Polar coordinates are a way of representing points in a plane using a distance from a reference point (radius) and an angle from a reference direction (usually the positive x-axis).
How do you convert polar coordinates to Cartesian coordinates?
-To convert from polar coordinates (r, α) to Cartesian coordinates (x, y), you can use the formulas: x = r * cos(α) and y = r * sin(α).
What is the significance of the angle α in polar coordinates?
-The angle α in polar coordinates indicates the direction of the point from the origin, and it determines in which quadrant the point lies based on the signs of x and y.
How is the radius (r) in polar coordinates calculated from Cartesian coordinates?
-The radius r can be calculated using the Pythagorean theorem: r = √(x² + y²), where x and y are the Cartesian coordinates.
What are the steps to find polar coordinates from Cartesian coordinates?
-To find polar coordinates from Cartesian coordinates (x, y), first calculate the radius r = √(x² + y²). Then, find the angle α using α = tan⁻¹(y/x) and adjust it according to the quadrant.
In which quadrant does the angle α lie when both x and y are positive?
-When both x and y are positive, the angle α lies in the first quadrant.
What formula is used to determine the angle α in different quadrants?
-The formula used is α = tan⁻¹(y/x), and then you adjust based on the quadrant: add 180° for the second quadrant and 360° for the fourth quadrant.
How do you find the distance between two points in polar coordinates?
-The distance P between two points in polar coordinates (R1, α1) and (R2, α2) can be calculated using the formula: P = √(R1² + R2² - 2 * R1 * R2 * cos(α2 - α1).
What is the relationship between the angle α and the quadrant in which a point lies?
-The angle α helps determine the quadrant: if both x and y are positive, α is in the first quadrant; if x is negative and y is positive, it's in the second quadrant; if both are negative, it's in the third; and if x is positive and y is negative, it's in the fourth.
What example was given for converting coordinates between polar and Cartesian?
-An example discussed was converting the polar coordinate A(12, 45°) to Cartesian coordinates by calculating x and y as x = 12 * cos(45°) and y = 12 * sin(45°), resulting in Cartesian coordinates (6√2, 6√2).
Outlines

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenMindmap

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenKeywords

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenHighlights

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenTranscripts

Dieser Bereich ist nur für Premium-Benutzer verfügbar. Bitte führen Sie ein Upgrade durch, um auf diesen Abschnitt zuzugreifen.
Upgrade durchführenWeitere ähnliche Videos ansehen

Konversi Koordinat Cartesius ke Koordinat Kutub/ Polar dan Sebaliknya

Coordenadas Polares ¿Qué son? EXPLICACIÓN COMPLETA

Polar Coordinates Basic Introduction, Conversion to Rectangular, How to Plot Points, Negative R Valu
TRIGONOMETRI (Konversi Koordinat Kutub Ke Koordinat Kartesius)

Vector Algebra (LEC -33) Polar coordinate system || Polar coordinate system || in Hindi ||

Bentuk Polar dan Eksponen dari Bilangan Kompleks
5.0 / 5 (0 votes)
