Factoring Polynomials using Greatest Common Monomial Factor
Summary
TLDRThis educational video script introduces grade 8 students to the concept of factoring polynomials using the greatest common monomial factor (GCMF). It explains the process of identifying factors and GCMF through examples with numbers and algebraic expressions. The script also covers prime factorization and demonstrates how to apply these techniques to factor binomials and polynomials, including the difference of cubes and sum of cubes, with the aim of simplifying complex expressions for easier understanding.
Takeaways
- 📚 The lesson is focused on factoring polynomials using the greatest common minimal factor, also known as the greatest common factor (GCF) or greatest common monomial factor (GCMF).
- 🔢 A factor is defined as a number or algebraic expression that divides another number or expression evenly with no remainder.
- 🌰 Examples are given to illustrate how to find the factors of numbers such as 20 and 10, and then identify their greatest common factor (GCF).
- 🔍 The script introduces the concept of prime factorization as a method to find the GCF of algebraic expressions, which involves breaking down numbers into their prime factors.
- 📝 The greatest common monomial factor (GCMF) is explained as the common factor with the smallest exponent for variables in algebraic expressions.
- ✂️ The process of dividing each term of a polynomial by the GCMF to find the other factors is described, using examples such as 6x + 3x^2 and 12x^3y^5 - 20x^5y^2z.
- 📉 The importance of identifying common factors in polynomials is emphasized to simplify the expression and make factoring easier.
- 📚 The script provides a step-by-step approach to factoring polynomials, including dividing each term by the GCMF and simplifying the result.
- 📈 The concept of exponents is applied when finding GCMF, where the variable with the smallest exponent is chosen for the factor.
- 📝 The script also covers how to handle terms without common variables or coefficients in the process of finding the GCMF.
- 🔚 The lesson concludes with a reminder to check the factored form by multiplying the factors to see if they yield the original polynomial, and a teaser for upcoming lessons on additional factoring techniques.
Q & A
What is the definition of a factor in mathematics?
-A factor is a number or algebraic expression that divides another number or expression evenly with no remainder.
What are the factors of 20?
-The factors of 20 are 1, 2, 4, 5, 10, and 20.
How do you find the greatest common factor (GCF) of two numbers?
-You list all the factors of each number and find the greatest factor that is common to both numbers.
What is the GCF of 20 and 10?
-The GCF of 20 and 10 is 10.
What is the greatest common monomial factor (GCMF) and when is it used?
-The GCMF is the greatest common factor of algebraic expressions or monomials, and it is used when dealing with polynomials or expressions that contain variables.
How does the method of prime factorization help in finding the GCMF of algebraic expressions?
-Prime factorization breaks down the numbers into their prime factors, making it easier to identify and align the common factors in the expressions.
What is the GCMF of 4x^3 and 8x^2?
-The GCMF of 4x^3 and 8x^2 is 4x^2.
How do you factor a polynomial using the GCMF method?
-You find the GCMF of the terms in the polynomial, divide each term by the GCMF, and then multiply the GCMF by the resulting factors.
What is the factored form of 6x + 3x^2 using the GCMF method?
-The factored form of 6x + 3x^2 is 3x(2 + x).
What is the GCMF of 12x^3y^5 - 20x^5y^2z and how is it used to factor the expression?
-The GCMF of 12x^3y^5 - 20x^5y^2z is 4x^3y^2. It is used to divide each term and find the remaining factors, resulting in the factored form 4x^3y^2(3y^3 - 5x^2z).
How does the script differentiate between the GCF and GCMF?
-The script differentiates by using the term GCF for numbers and GCMF for algebraic expressions or monomials, emphasizing the variable with the smallest exponent in the GCMF.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة

Factoring Greatest Common Monomial Factor

Factor Polynomials with GCMF Video

Grade 8 Math Q1 Ep1: Factoring Polynomials

Common Monomial Factoring - Polynomial Factoring - Grade 8 Math
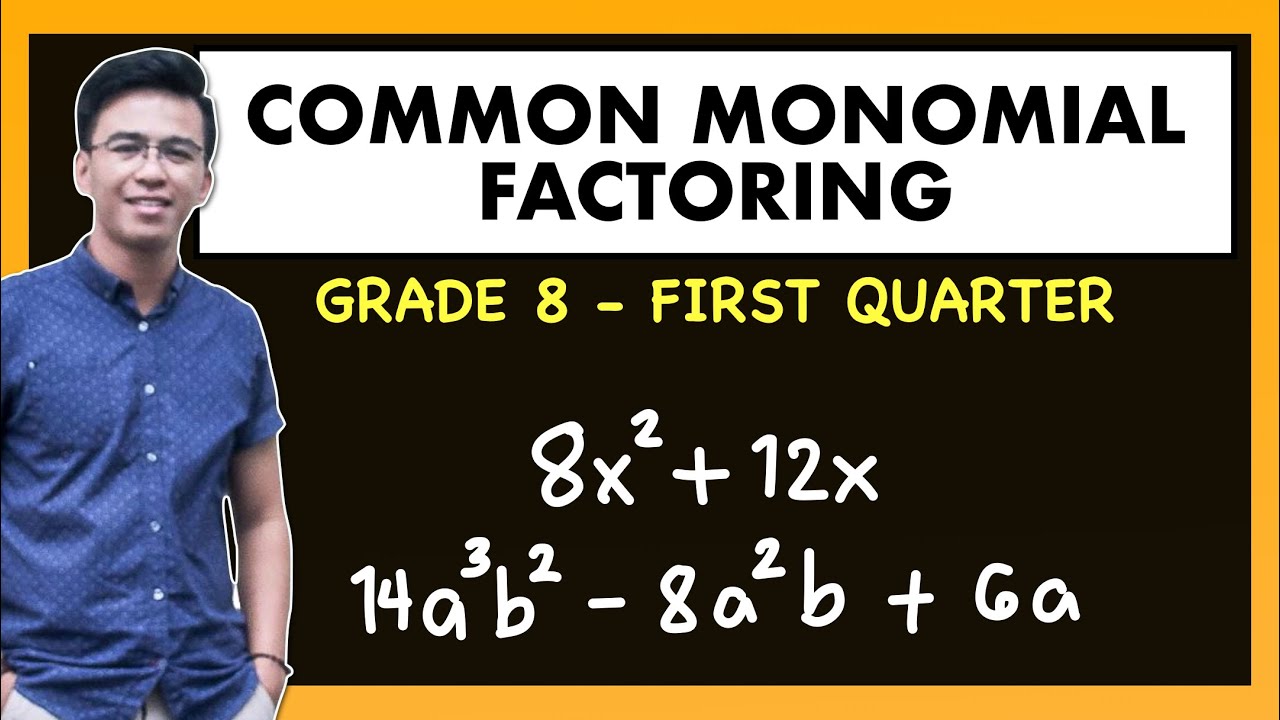
Factoring Part 1 - Common Monomial Factoring | Grade 8 Q1 @MathTeacherGon
Factoring Cubic Polynomials- Algebra 2 & Precalculus
5.0 / 5 (0 votes)
