MINI-LESSON 1: Breaking down intuitively the concept of standard deviation. Why pple don't get it.

Summary

The speaker discusses the common misunderstandings in statistics, particularly the misuse of standard deviation versus mean absolute deviation. He clarifies that standard deviation, often misconstrued as an average daily movement, actually weights larger observations more heavily due to squaring deviations. The talk delves into the statistical consequences of fat tails and challenges the intuitiveness of standard deviation, highlighting its limitations in non-linear environments and the importance of understanding statistical metrics correctly.

Takeaways

• 📊 The speaker emphasizes the misunderstanding of statistical concepts, particularly the concept of correlation, and how it's often misinterpreted by even practicing statisticians.
• 🔍 The script discusses the misuse of standard deviation as a metric for understanding variability, pointing out that it is not an intuitive measure of how much something moves on average.
• 🧐 The speaker clarifies that standard deviation is often confused with mean absolute deviation, highlighting the difference in how they treat large and small observations.
• 📚 Reference is made to the speaker's work in 'Statistical Consequences of Fat Tails,' where the issues with standard deviation and its relation to fat tails are explored in detail.
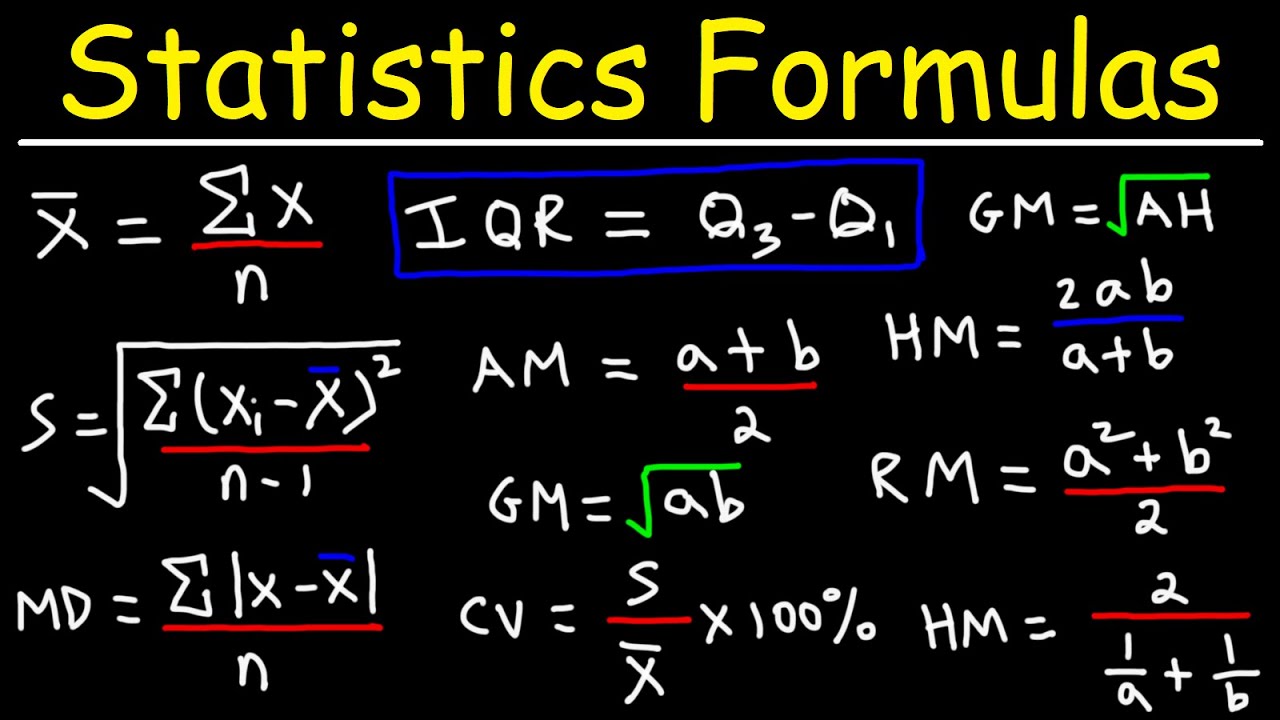
• 📉 The script explains the mathematical formula for standard deviation, emphasizing the squaring of deviations which disproportionately weights larger observations.
• 🤔 The speaker questions why standard deviation is preferred over mean absolute deviation, especially since people often have the latter in mind when they think about variability.
• 📈 The ratio of standard deviation to mean absolute deviation is shown to be related to the fat tails of a distribution, with different values for Gaussian and other distributions.
• 🌡️ An example is given about temperature changes, illustrating how people naturally think about variability without squaring deviations, contrary to the standard deviation calculation.
• 🤷‍♂️ The absurdity of standard deviation is demonstrated with an example where one outlier greatly influences the result, while the mean absolute deviation remains small.
• 🔄 The script points out that standard deviation is not additive, showing that it does not behave intuitively when combining observations.
• 🔗 The concept of correlation is introduced towards the end, with the speaker promising to further discuss its misconceptions and failures in non-linear environments in future talks.

Q & A

What is the main issue discussed in the script regarding the understanding of statistical concepts?

The script discusses the misunderstanding of statistical concepts, particularly the misuse and misinterpretation of standard deviation and correlation by even practicing statisticians.

What is the common misconception about standard deviation according to the speaker?

The common misconception is that people often think of standard deviation as the average amount something moves every day, rather than understanding it as a measure of the dispersion of a set of values.

How does the speaker define standard deviation in the context of the script?

The speaker defines standard deviation as the square root of the average of the squared differences from the mean, emphasizing that it gives more weight to larger observations due to the squaring of deviations.

What is the difference between standard deviation and mean absolute deviation as discussed in the script?

Standard deviation involves squaring the deviations from the mean, giving more weight to larger observations, while mean absolute deviation is the average of the absolute deviations from the mean without squaring, treating all deviations equally.

Why does the speaker argue that standard deviation is not an intuitive metric for understanding data?

The speaker argues that standard deviation is not intuitive because it is not additive and does not correspond to what people typically have in mind when they think about how much something changes on average.

What is the significance of the ratio between standard deviation and mean absolute deviation in the context of fat tails?

The ratio between standard deviation and mean absolute deviation corresponds to the fat tails of a distribution, with higher ratios indicating more pronounced fat tails, which can distort statistical analysis.

Why does the script mention the importance of understanding the difference between standard deviation and mean absolute deviation?

Understanding the difference is crucial because it highlights the potential for misinterpretation in statistical analysis, especially when dealing with distributions that have fat tails, which are not accurately represented by standard deviation.

What is the speaker's view on the use of standard deviation in the context of Gaussian distribution?

The speaker acknowledges that standard deviation makes sense for a Gaussian distribution because it aligns with the distribution's properties, such as having about 67.8% of observations within one standard deviation from the mean.

How does the script illustrate the problem with using standard deviation as a measure of change, using the example of temperature changes?

The script uses the example of temperature changes to show that people intuitively think about changes in terms of absolute values rather than squared deviations, which is what standard deviation calculates.

What is the concept of 'beta' mentioned in the script, and why does it fail in a non-linear environment?

The 'beta' mentioned in the script is a normalized measure of correlation, typically calculated as the correlation divided by the standard deviation of one of the variables. It fails in a non-linear environment because it assumes a linear relationship, which may not hold true in all cases.

What does the speaker suggest about the use of standard deviation and correlation in statistical analysis?

The speaker suggests that the use of standard deviation and correlation can lead to misunderstandings and misinterpretations, especially when they do not align with the intuitive understanding of changes or relationships in data.