BAHAS KONSEP PERSAMAAN GARIS LURUS DARI AWAL! (Episode 1)

Summary

In this video, the concept of straight line equations is explored in detail, beginning with the basics of gradients and their significance in understanding line slopes. The presenter explains how to calculate the gradient and derive line equations using either a given gradient and point or two known points on the line. Various methods for finding line equations are demonstrated, including quick techniques for special cases. The video also touches on how to read line equations, determine intercepts, and solve for specific points on the graph, making it a comprehensive guide to mastering straight line equations.

Takeaways

• 😀 Understanding the equation of a straight line is crucial, and it involves concepts such as the line, gradient, and coordinates.
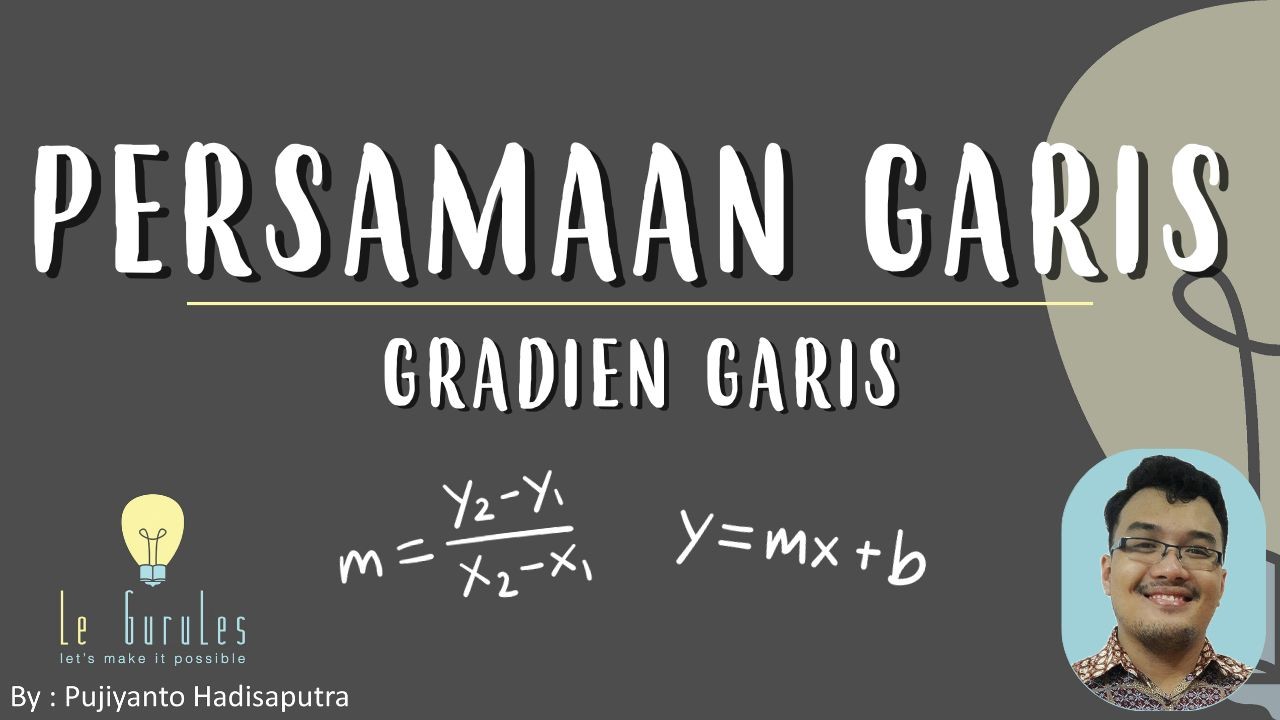
• 😀 The gradient (m) represents the slope of the line and is calculated as the change in y divided by the change in x (Δy/Δx).
• 😀 Positive and negative gradients correspond to lines sloping upward and downward, respectively, across different quadrants of a graph.
• 😀 The gradient is steeper when the line is closer to the y-axis, and the greater the distance, the smaller the gradient.
• 😀 There are two main ways to determine the equation of a line: using the gradient and a known point, or using two points the line passes through.
• 😀 The equation of a line can be derived using the point-gradient formula: y - y1 = m(x - x1), where m is the gradient and (x1, y1) is a point on the line.
• 😀 If two points are given, the gradient can be calculated by finding the difference in y-values (Δy) and x-values (Δx), then equating the two gradients for consistency.
• 😀 When calculating the equation of a line, you can simplify the formula by substituting values for the coordinates and gradient into the point-gradient form.
• 😀 For quick calculations, use shortcuts to find the gradient: it’s the coefficient of x in the equation of the line when rearranged into y = mx + c form.
• 😀 The vertical line (x = a) has an undefined gradient, while the horizontal line (y = b) has a gradient of 0, highlighting the difference in behavior between line types.

Q & A

What are the key components of a straight line equation?

The key components of a straight line equation include the line itself, the gradient (slope), and the intercept, which is often denoted as 'b' in the equation y = mx + b.

What is the definition of the gradient in a straight line?

The gradient (or slope) of a straight line, denoted by 'm', is defined as the ratio of the change in the y-coordinate to the change in the x-coordinate, often expressed as Δy/Δx.

How do positive and negative gradients differ geometrically?

A positive gradient results in an upward sloping line from left to right, while a negative gradient produces a downward sloping line. These lines appear in different quadrants: positive gradients in the 1st and 3rd quadrants, and negative gradients in the 2nd and 4th quadrants.

What is the formula for determining the equation of a line when the gradient and a point on the line are known?

When the gradient 'm' and a point (x1, y1) on the line are known, the equation of the line can be written as: y - y1 = m(x - x1).

How is the gradient determined when two points on the line are known?

The gradient between two points (x1, y1) and (x2, y2) is calculated as (y2 - y1) / (x2 - x1). This gradient is used in the line equation.

How do you handle the case when both x and y values are given for two points?

When both x and y values for two points are known, the gradient is calculated by finding the difference in y-values divided by the difference in x-values, then this gradient is used in the line equation.

What is the process for finding the equation of a line given the gradient and a specific point?

If the gradient 'm' and a point (x1, y1) are known, substitute the values into the equation y - y1 = m(x - x1), simplify, and rearrange the terms to find the full equation of the line.

How do you quickly find the equation of a line when one point is on the x-axis and the other is on the y-axis?

In this case, the equation can be derived quickly by noting that the x-intercept is the x-coordinate where y = 0, and the y-intercept is the y-coordinate where x = 0. Use the point-slope form to simplify the equation.

How do you interpret the constant term in a straight line equation?

The constant term, often denoted 'c' in the equation y = mx + c, represents the y-intercept, which is the point where the line crosses the y-axis (when x = 0).

What happens to the gradient when the line is vertical or horizontal?

For a vertical line, the gradient is undefined because the change in x is zero, leading to division by zero. For a horizontal line, the gradient is zero, as there is no change in the y-values.