Identidades trigonométricas resoluções de exercícios
Summary
TLDRIn this educational script, the speaker walks through various trigonometric identities, focusing on proving and simplifying key formulas involving sine, cosine, secant, and tangent functions. Through a series of exercises, the speaker demonstrates how to manipulate equations and verify fundamental relationships like secant and tangent identities. The explanations are detailed and aim to clarify the core concepts of trigonometry for students. The video emphasizes the importance of understanding these identities, using both algebraic manipulation and geometric principles to derive and confirm essential trigonometric formulas.
Takeaways
- 😀 Trigonometric identities, like secant squared and tangent squared, are proven through mathematical manipulation and relationships between various trigonometric functions.
- 😀 The proof of secant squared being equal to 1 + tangent squared involves working with basic trigonometric identities and understanding their fundamental relationships.
- 😀 The process of proving an identity involves choosing one side and transforming it to match the other side using known trigonometric identities.
- 😀 The importance of using the Pythagorean identity (sin²x + cos²x = 1) is emphasized in simplifying trigonometric expressions.
- 😀 The proof for the identity 1 + tangent squared = secant squared relies on manipulating sine and cosine functions to show equivalence.
- 😀 The process of isolating one function, like cosine or sine, and using it to derive relationships is a key strategy in trigonometric proofs.
- 😀 The identity involving secant, tangent, and cosine functions is frequently used to transform and simplify expressions.
- 😀 Another identity discussed is the relationship between the cosecant and secant functions, which can be derived through algebraic manipulation of sine and cosine.
- 😀 Fractions and common denominators play a significant role in proving trigonometric identities, especially when working with secant and cosine functions.
- 😀 The script highlights the importance of verifying trigonometric identities by performing algebraic operations and ensuring that both sides of the equation are equivalent.
Q & A
What is the main goal of the exercise discussed in the transcript?
-The main goal is to prove various trigonometric identities, such as showing that secant squared x equals 1 plus tangent squared x, and verifying other similar identities.
What trigonometric identity is demonstrated at the beginning of the transcript?
-At the beginning, the identity being demonstrated is secant squared x equals 1 plus tangent squared x, which is a fundamental trigonometric identity.
What method is used to prove the identity involving secant and tangent functions?
-The method involves starting with one side of the identity, transforming it using known trigonometric relations (such as sine squared x plus cosine squared x equals 1), and simplifying both sides to show they are equal.
How is the identity secant squared x = 1 + tangent squared x proven in the transcript?
-The proof starts by expressing secant squared x in terms of sine and cosine, then using the identity sine squared x + cosine squared x = 1, and simplifying to show that secant squared x is indeed equal to 1 + tangent squared x.
What other identities are introduced in the transcript aside from secant and tangent?
-Other identities introduced include the relationships involving sine, cosine, and cosecant, such as sine squared x + cosine squared x = 1, and how to manipulate these to prove various trigonometric expressions.
What is the role of sine and cosine in these trigonometric identities?
-Sine and cosine play a key role in simplifying and proving trigonometric identities. For instance, the relationship sine squared x + cosine squared x = 1 is used to rewrite and simplify expressions involving secant, tangent, and cosecant functions.
What does the 'fundamental relationship' mentioned in the transcript refer to?
-The fundamental relationship refers to the identity sine squared x + cosine squared x = 1, which is essential in deriving and proving other trigonometric identities.
What is the significance of the example where secant and cosecant functions are involved?
-The example demonstrates how secant and cosecant can be expressed in terms of sine and cosine, and how these expressions can be manipulated to prove more complex trigonometric identities.
How does the transcript address the relationship between sine, cosine, and tangent?
-The transcript emphasizes how sine, cosine, and tangent are interconnected, with specific focus on how sine squared x and cosine squared x relate to tangent and secant functions, enabling the proof of various identities.
What mathematical principle is frequently applied in the proof steps in the transcript?
-The principle of manipulating trigonometric functions using algebraic identities, particularly the Pythagorean identity sine squared x + cosine squared x = 1, is frequently applied to simplify and prove trigonometric expressions.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة

Identitas Trigonometri - Part 1

Identitas Trigonometri: Identitas Kebalikan, Perbandingan dan Pythagoras - SMA Kelas 10

[Math 20] Lec 3.4 Fundamental Identities (1 of 2)

Identitas Trigonometri | Matematika Wajib Kelas X

Identidades trigonométricas, Identidades de simetría e identidades de suma y resta.
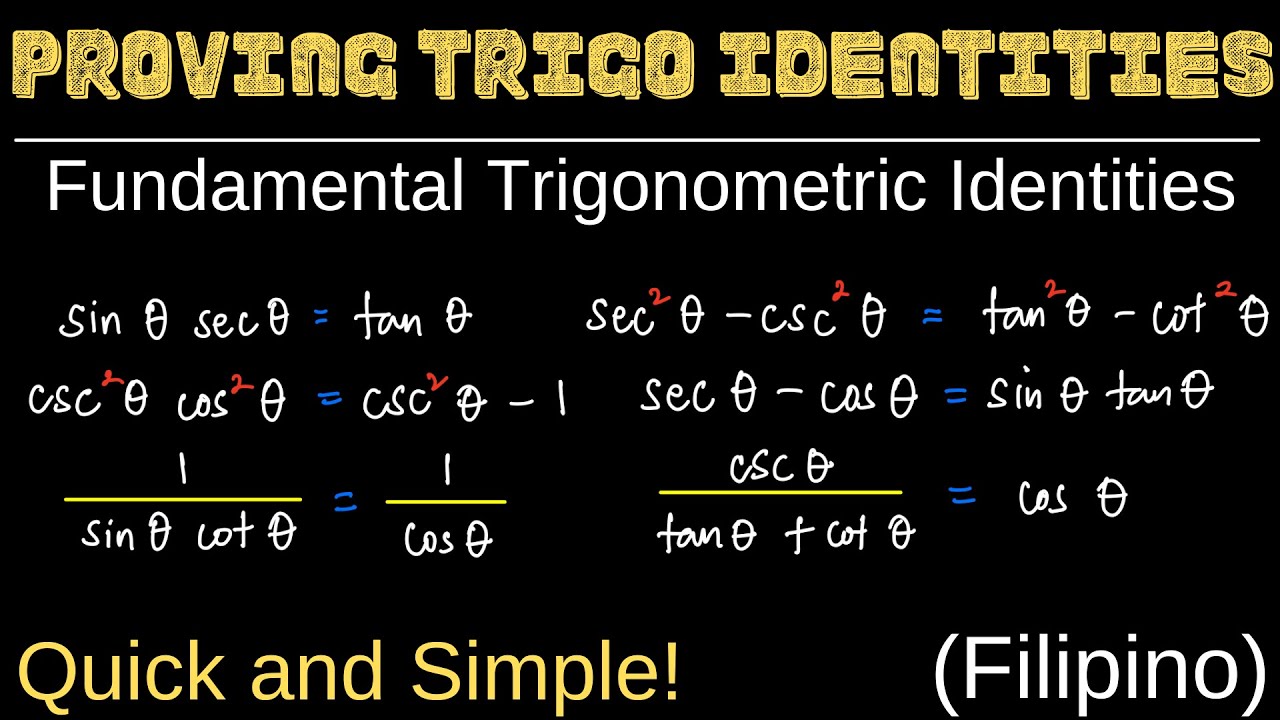
Proving Trigonometric Identities | Fundamental Trigonometric Identities | Formulas | Sample Problems
5.0 / 5 (0 votes)
