Kesebangunan & Kongruensi (1) - Model dan Skala, Perbandingan Foto - Matematika SMP
Summary
TLDRIn this educational video, the host teaches viewers about similarity and congruence, focusing on scale drawings and models. The content covers how to use scale to represent real-life objects, including buildings, toys, and maps. The video also walks through various examples involving calculating distances, areas, and actual sizes based on scale, with step-by-step solutions for practical problems. The host provides clear explanations and encourages viewers to engage by subscribing to the channel and sharing the video to help others learn. The goal is to help viewers understand how scale works and apply it to real-world scenarios.
Takeaways
- 😀 Scale drawings represent real-life objects by maintaining proportional relationships, making them easier to visualize and understand.
- 😀 A scale model or drawing is based on a ratio between the model's dimensions and the actual object's dimensions, such as 1:60 or 1:200.
- 😀 The scale of a drawing can be calculated using the formula: Scale = Map distance / Real-life distance, ensuring the model accurately represents reality.
- 😀 It's essential to convert units consistently when working with scales (e.g., converting kilometers to centimeters or meters to centimeters) for accurate results.
- 😀 Real-life distances or dimensions can be determined from a scale drawing by using cross multiplication, with the scale acting as a conversion factor.
- 😀 To find the area or volume of objects in scale, you may calculate the dimensions of the model first, then apply the scale to get the actual measurements.
- 😀 A scale drawing can be used to estimate actual distances, such as calculating the true length of a river or the height of an object like a building.
- 😀 When using scale for practical applications like cost estimation, such as calculating the cost of installing grass in a garden, accurate measurements and unit conversions are crucial.
- 😀 To calculate real-life areas from scaled drawings, one can either calculate the dimensions first or find the area of the scaled version and then apply the scale factor.
- 😀 Always ensure that the scale factor is correctly squared when dealing with area calculations, as the relationship between dimensions and area is quadratic.
- 😀 Understanding scale and proportionality is fundamental in fields like architecture, engineering, and mapmaking, allowing for accurate representation and calculations.
Q & A
What is a scale model, and how is it used in real life?
-A scale model is a representation of an object, such as a building or toy, that maintains the same proportions as the original item. In real life, scale models are commonly used in architecture, toy design, and map creation to represent large or complex structures in a manageable size.
What is the purpose of using a scale in drawings and maps?
-The purpose of using a scale in drawings and maps is to create a proportional relationship between the drawing and the actual size of the object or area. This allows large or geographically distant places to be represented in a much smaller, manageable format while maintaining accuracy.
How do you calculate the scale of a map or drawing?
-To calculate the scale, divide the distance on the map by the corresponding actual distance. The scale can be written as a ratio (e.g., 1:800,000), showing the relationship between map units and actual units.
In the example with a 3.5 cm distance representing 28 km on a map, what is the scale of the map?
-The scale of the map is 1:800,000. This is calculated by converting 28 km into centimeters (28 km = 2,800,000 cm) and dividing the map distance (3.5 cm) by the actual distance (2,800,000 cm). The resulting ratio is 1:800,000.
How do you calculate the actual distance of a river when the map distance and scale are known?
-To calculate the actual distance, multiply the map distance by the scale factor. For example, if the map distance is 4.3 cm and the scale is 1:800,000, multiply 4.3 by 800,000 to find the actual distance of the river, which is 34.4 km.
If a house is built with a scale of 1:1500, and the model’s length is 15 cm and width is 10 cm, how can you find the actual dimensions?
-To find the actual dimensions, multiply the model dimensions by the scale factor. For length, 15 cm × 1500 = 22,500 cm, which is 225 meters. For width, 10 cm × 1500 = 15,000 cm, which is 150 meters.
How do you calculate the area of a house in real life when given the model's dimensions and scale?
-You can either first convert the model dimensions to real dimensions and then calculate the area, or you can calculate the area of the model and then scale it up. If the model's dimensions are 15 cm by 10 cm, the real dimensions will be 225 meters by 150 meters. The real area would then be 225 m × 150 m = 33,750 m².
What are the two methods for calculating the real area of an object based on its scale model?
-The first method involves converting the model’s dimensions to real dimensions and then calculating the area. The second method involves calculating the model’s area and scaling it up by the square of the scale factor, as area is a two-dimensional measure.
In the case of a tree on a TV screen, how do you calculate its real height if the scale is not provided?
-To calculate the real height of the tree, first determine the scale of the TV image by comparing the known height of a building (70 meters) and its corresponding height on the TV (15 cm). Then use this scale to find the real height of the tree based on its height on the TV (1 cm).
How do you calculate the cost of installing grass in a garden based on the scale model?
-To calculate the cost, first convert the scale dimensions of the garden to real dimensions by using the scale ratio. For example, if the garden’s model dimensions are 12 cm by 8 cm, the real dimensions are 48 meters by 32 meters. Then, find the area to be covered by grass (3/4 of the total area) and multiply it by the cost per square meter to find the total cost.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة

Kekongruenan dan Kesebangunan [Part 3] - Kesebangunan Bangun Datar
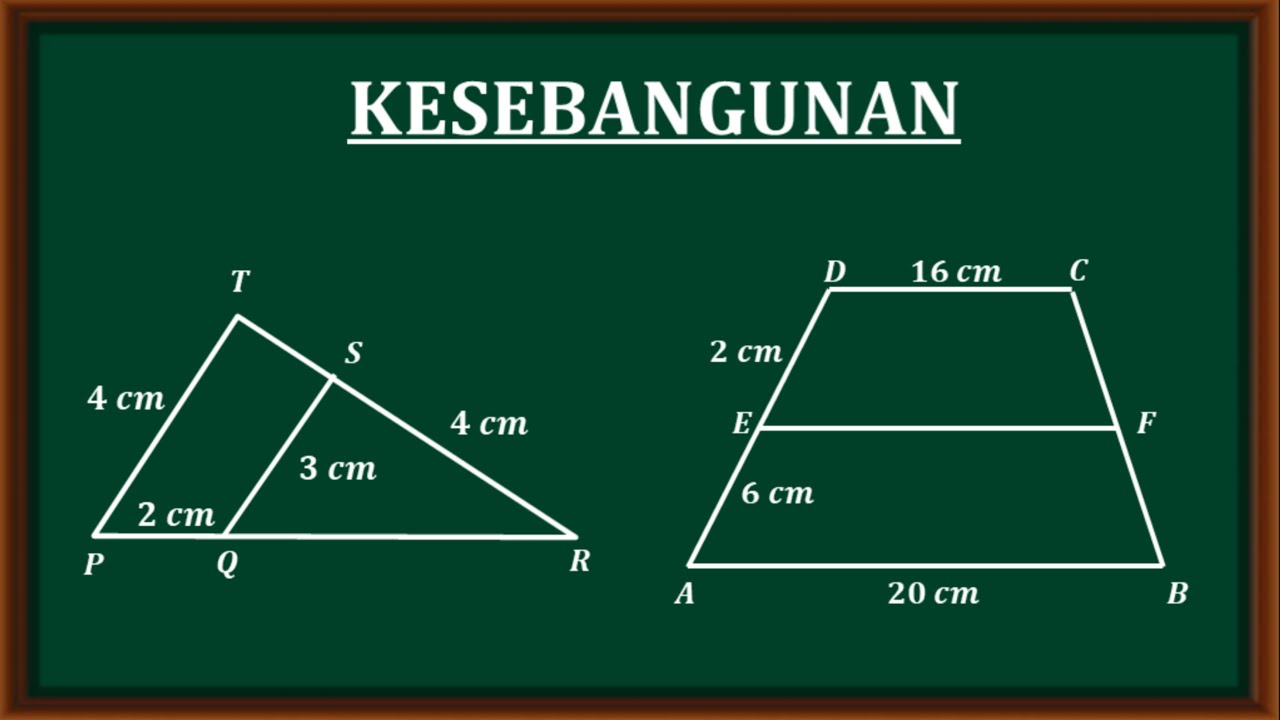
Kesebangunan - soal dan pembahasan materi kesebangunan matematika tingkat SMP kelas ix

Kesebangunan & Kongruensi (6) - Rumus Kongruensi, Pembuktian Kongruensi - Matematika SMP

Mental Models Montage: Parkinson's Law + Pareto 80/20 + Bike-shedding + First Things First + MORE !
Triangles | Introduction | Chapter 6 | "लक्ष्य" 2025

KEKONGRUENAN (Materi Kelas 9 SMP)
5.0 / 5 (0 votes)
