Transformasi Geometri Bagian 4 - Dilatasi (Perkalian) Matematika Wajib Kelas 11
Summary
TLDRIn this video, the concept of geometric dilation is explained, highlighting how it changes the size of shapes while maintaining their form. The presenter covers the fundamental aspects of dilation, such as the scale factor (k), and demonstrates how shapes are either enlarged or reduced based on this factor. Examples include how to calculate the new coordinates of a point after dilation using both matrix multiplication and a simplified method. The video also explains dilation with a center not at the origin and provides a detailed example involving the dilation of lines and curves. The concept is thoroughly explored with various scenarios to aid understanding.
Takeaways
- 😀 Dilatation (scaling) is a geometric transformation that changes the size of an object while keeping its shape and orientation the same.
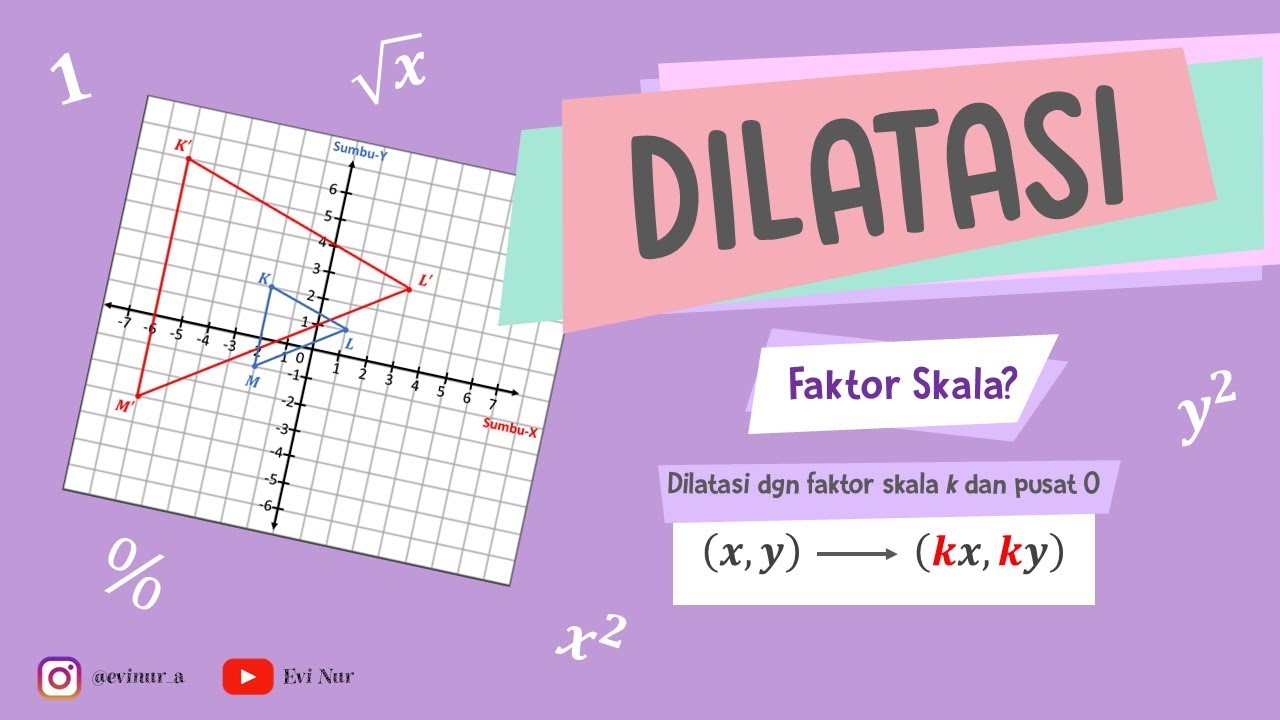
- 😀 Dilatation involves a scaling factor (k) that determines how much an object is enlarged or reduced. For example, if k=2, the object’s size will double.
- 😀 The center of dilation is the point from which all scaling transformations are measured. Commonly, it is at the origin (0,0), but it can be any point.
- 😀 If the scaling factor (k) is greater than 1, the object will be enlarged. If k is between 0 and 1, the object will be reduced in size.
- 😀 If the scaling factor is negative, it not only changes the size but also flips the object, resulting in a reflection across the center of dilation.
- 😀 To find the image of a point after dilation, multiply the coordinates of the point by the scaling factor (k).
- 😀 The dilation of a triangle, for example, can be calculated by extending the distance between each vertex and the center of dilation by a factor of k.
- 😀 A point (x, y) dilated by a factor k from the origin can be computed using the formula (x', y') = (kx, ky).
- 😀 If the center of dilation is not at the origin, the coordinates are first adjusted by subtracting the center’s coordinates, then multiplied by the scaling factor, and the center’s coordinates are added back.
- 😀 When dilating a line or curve, the process is similar: apply the dilation transformation to each point along the line or curve, and substitute the transformed coordinates into the equation.
Q & A
What is dilatation in geometry?
-Dilatation is a transformation that changes the size of an object while preserving its shape. It involves scaling the object either by enlarging or reducing its size based on a center of dilation and a scale factor.
What are the key components of a dilatation transformation?
-The key components of dilatation are the center of dilation and the scale factor. The center of dilation is the point from which the object is scaled, and the scale factor determines how much the size changes.
How does a scale factor greater than 1 affect the dilated object?
-When the scale factor (k) is greater than 1, the object is enlarged, meaning the distances from the center of dilation to the points on the object are increased.
What happens when the scale factor is between 0 and 1?
-If the scale factor is between 0 and 1 (i.e., 0 < k < 1), the object is reduced. This means the distances from the center of dilation to the points are scaled down.
What is the effect of a negative scale factor on an object?
-A negative scale factor results in a reflection of the object across the center of dilation, while also scaling its size. For example, a scale factor of -1 reflects the object without changing its size, while a scale factor of -2 would reflect and enlarge the object.
How do you determine the new coordinates of a point after dilation with center at the origin?
-To find the new coordinates of a point after dilation with the center at the origin (0, 0), multiply the original coordinates (x, y) by the scale factor k. The new coordinates will be (kx, ky).
How do you calculate the new coordinates when the center of dilation is not the origin?
-When the center of dilation is not the origin, you first subtract the coordinates of the center of dilation from the original point, apply the scale factor, and then add back the coordinates of the center of dilation to get the new coordinates.
What is the process for dilating a line or curve?
-The process for dilating a line or curve is similar to dilating points. You adjust the equation of the line or curve based on the scale factor and the center of dilation, applying the dilation to the coordinates or terms in the equation.
What is the difference between dilating a point and dilating a line?
-Dilating a point involves scaling its distance from the center of dilation, while dilating a line involves adjusting the equation of the line based on the scale factor and center of dilation. Both processes require applying the scale factor and potentially a translation step if the center is not at the origin.
How can dilatation be used to solve practical problems in geometry?
-Dilatation can be used to solve problems involving the resizing of objects, such as scaling a shape to fit into a particular space, determining how the size of an object changes when distances are scaled, or analyzing geometric patterns that involve scaling transformations.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآن5.0 / 5 (0 votes)