Cara Menghitung Skala Peta/ Geografi Kelas X/ Kurikulum Merdeka
Summary
TLDRThis video from the 'Kita Pintar' YouTube channel explains how to calculate map scales in geography. It covers several methods for determining scales, such as comparing two maps, using actual distances, and utilizing contour lines. The presenter walks through detailed formulas, examples, and practice problems, ensuring a clear understanding of each concept. The video also touches on astronomical lines and the conversion between units for accuracy in scale determination. The content is ideal for students looking to master map scale calculations through various real-world scenarios and examples.
Takeaways
- 🗺️ The video teaches how to calculate map scales in geography.
- 📐 The first method involves comparing two maps with different scales using the formula P2 = (D1 / D2) * P1.
- 🔢 When comparing two maps, the known elements are usually the distance and scale of the first map, and the task is to find the scale of the second map.
- 🧮 Example: If P1 is 1:50,000, D1 is 2 cm, and D2 is 4 cm, then the scale for P2 would be 1:25,000.
- 🛤️ The second method uses the actual distance by applying the formula S = JP / JS, where JP is the distance on the map, and JS is the real-world distance.
- 🌍 Example: If JP is 8 cm and JS is 160 km (converted to 16,000,000 cm), the map scale would be 1:2,000,000.
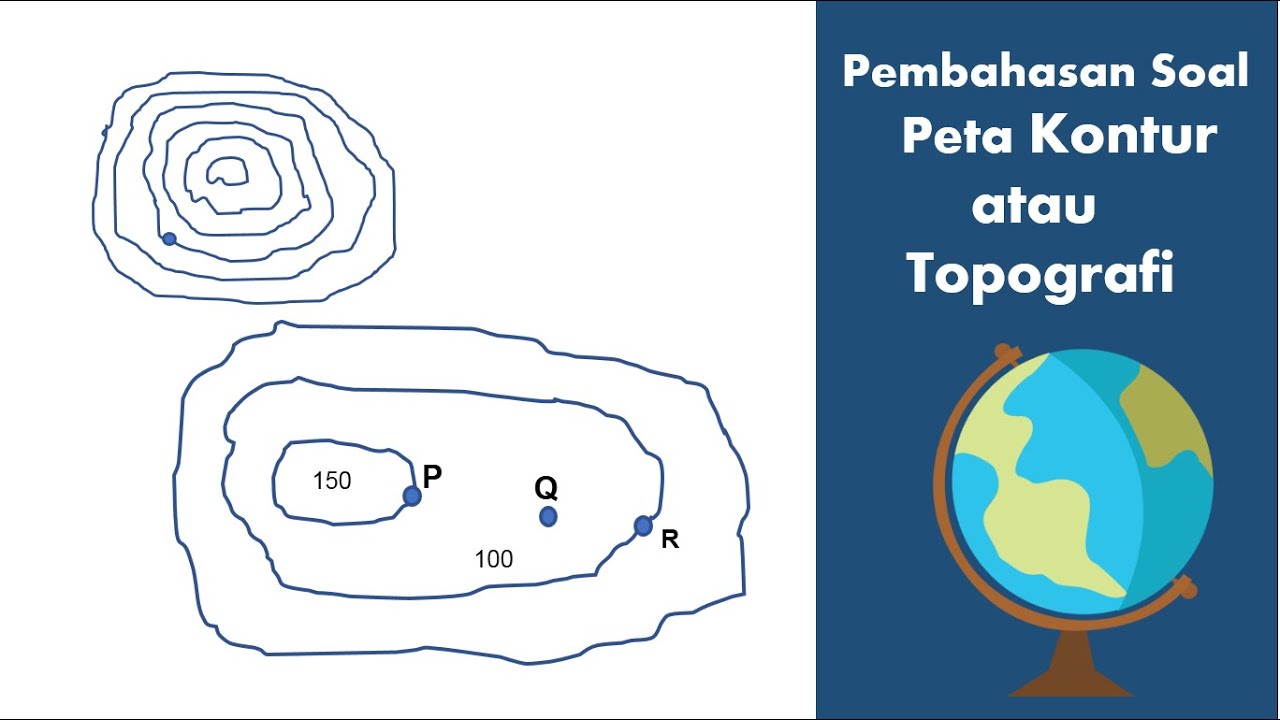
- ⛰️ Another technique is using contour lines to calculate the scale, where contour intervals are derived from known elevation points using a fixed constant (1/2000).
- 📊 The formula for contour lines: Contour Interval (C) = 1/2000 * PS (scale denominator).
- 🧭 The fourth method involves using astronomical lines, converting degree differences into kilometers (1 degree = 111 km), and then calculating the map scale.
- 📖 Example question: If the real distance between two cities is 50 km, and the map distance is 2.5 cm, the map scale would be 1:2,000,000.
Q & A
What is the first method to calculate map scale mentioned in the video?
-The first method to calculate map scale is by comparing it with another map that has a known scale. The formula used is P2 = (D1 / D2) * P1, where P2 is the unknown scale, D1 is the known map distance, D2 is the unknown map distance, and P1 is the known map scale.
How is the formula P2 = (D1 / D2) * P1 applied in a sample problem?
-In the example provided, D1 is 2 cm, D2 is 4 cm, and P1 is 1:50,000. The formula P2 = (D1 / D2) * P1 is applied as follows: (2 / 4) * 50,000 = 25,000, meaning the scale of the second map is 1:25,000.
What is the relationship between map distance and map scale?
-The relationship is inverse: as the map distance (D) increases, the map scale (P) decreases. For example, if the distance on one map is larger, its scale will be smaller than that of another map.
What is the second method for calculating map scale mentioned in the video?
-The second method involves comparing the map distance to the actual distance. The formula used is S = JP / JS, where S is the scale, JP is the map distance, and JS is the actual distance.
How is the second method applied to a specific problem?
-In an example problem, JP (map distance) is 8 cm, and JS (actual distance) is 160 km, which is converted to 16 million cm. Using the formula S = JP / JS, the scale is 1:2,000,000.
What is a contour line and how is it related to map scale calculation?
-A contour line is a line on a map connecting points of the same elevation. To calculate the scale using contour intervals, the formula C = 1 / 2000 * PS is used, where PS is the scale denominator.
What is the constant used when calculating contour intervals?
-The constant used is 1/2000. This constant is multiplied by the scale to determine the contour interval, which represents the vertical spacing between contour lines.
How is map scale calculated using astronomical lines?
-To calculate map scale using astronomical lines, the actual distance must first be determined by multiplying the degree difference by 111 km (the distance per degree). Then, the formula S = JP / JS is applied.
What is the conversion between degrees, minutes, and seconds for astronomical lines?
-One degree equals 111 km, one minute equals 1.85 km, and one second equals 30.8 meters. These conversions are used to calculate the actual distance between points on a map when working with astronomical lines.
How is map scale calculated for two maps with different distances?
-When comparing two maps, the formula P1 = (D2 / D1) * P2 is used. For example, if D1 is 8 cm and D2 is 5 cm, and the scale of the second map (P2) is 1:200,000, then P1 = (5 / 8) * 200,000 = 1:125,000.
Outlines

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنMindmap

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنKeywords

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنHighlights

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنTranscripts

هذا القسم متوفر فقط للمشتركين. يرجى الترقية للوصول إلى هذه الميزة.
قم بالترقية الآنتصفح المزيد من مقاطع الفيديو ذات الصلة
5.0 / 5 (0 votes)