Barisan dan Deret Bagian 2 - Deret Aritmetika Matematika Wajib Kelas 11

Summary

In this video, Deni Handayani explains the concept of arithmetic sequences and series, breaking down key formulas and providing detailed examples. He introduces the general definition of a series as the sum of terms in a sequence and walks through two main formulas for calculating the sum of an arithmetic series (SN). The video covers several problems, from basic calculations to more complex exam-style questions, demonstrating how to apply these formulas to find solutions. Viewers are guided through step-by-step solutions, making the video an excellent resource for mastering arithmetic sequences and series.

Takeaways

• 😀 The video focuses on arithmetic series and how to calculate the sum of the first n terms using different formulas.
• 😀 A sequence of numbers is a series when the terms of the sequence are summed together, and the sum of the first n terms is denoted by SN.
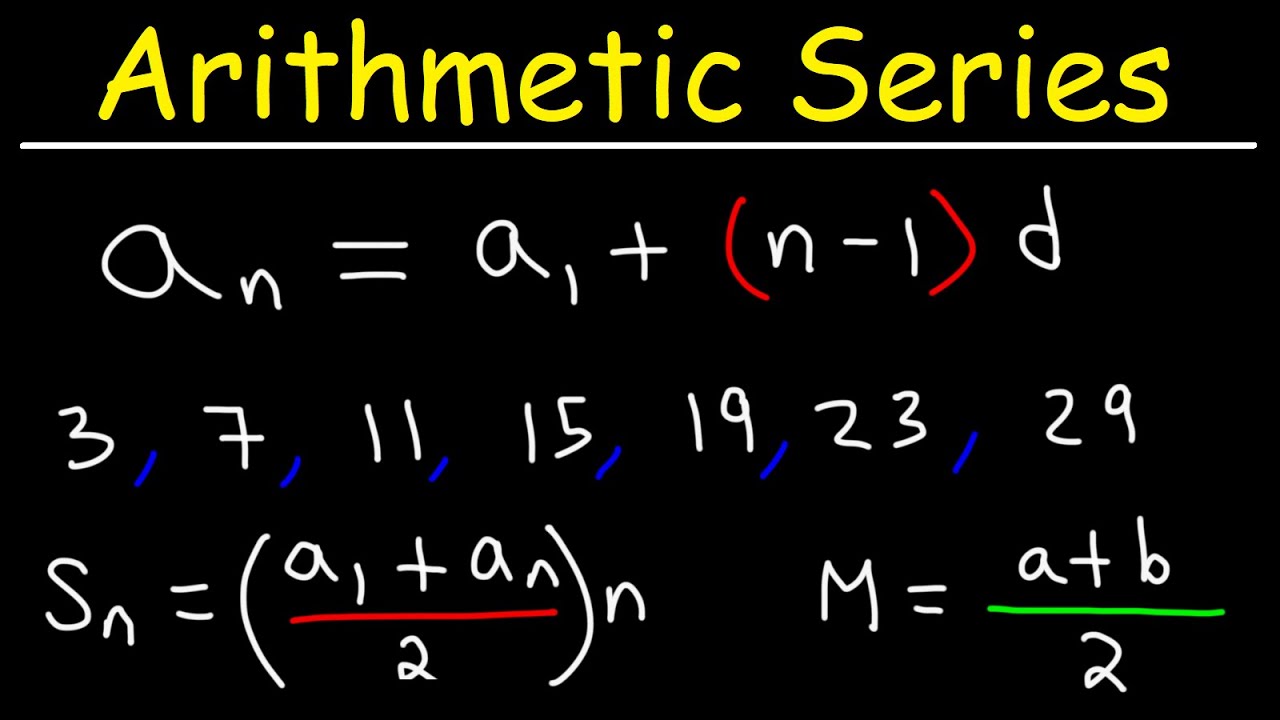
• 😀 The two primary formulas for calculating the sum of an arithmetic series (SN) are: SN = n/2 * (2A + (n-1) * B) and SN = n/2 * (A + UN), where A is the first term and B is the common difference.
• 😀 Deriving these formulas is important for understanding how to apply them in different contexts, but it’s suggested to explore the derivation in separate resources.
• 😀 In the example problem, the sum of the first 9 terms of an arithmetic series is calculated using one of these formulas, with step-by-step solutions for better understanding.
• 😀 Arithmetic sequences have the property that the difference between any two consecutive terms is constant. This property helps in solving for unknown terms.
• 😀 For example, if given the first three terms of a sequence in algebraic form, solving for the sum of terms involves determining the common difference and the first term.
• 😀 The second example highlights the use of given terms like U7 and U3 + U9 to create equations, which can be solved simultaneously to find unknowns.
• 😀 The video covers both problem-solving techniques and formula usage for practical applications such as determining the sum of specific terms in an arithmetic sequence.
• 😀 The approach is practical and geared towards real-world examples like seating arrangements or determining totals in fixed-progress sequences, such as movie theater seats or multiples of a number like 3.

Q & A

What is the general definition of a sequence in mathematics?

A sequence in mathematics is a list of numbers arranged in a specific order. In this video, the concept of a sequence is explained as a series of numbers, which could represent a pattern, such as an arithmetic sequence.

What is an arithmetic sequence?

An arithmetic sequence is a sequence of numbers where the difference between consecutive terms is constant. This constant difference is called the 'common difference.'

How is an arithmetic series related to an arithmetic sequence?

An arithmetic series is the sum of the terms of an arithmetic sequence. While a sequence lists the terms in order, a series sums them to find a total value.

What does SN represent in the context of an arithmetic series?

SN represents the sum of the first 'n' terms of an arithmetic sequence. For example, S6 would be the sum of the first six terms, and S100 would be the sum of the first 100 terms.

What are the two formulas for finding SN in an arithmetic series?

The two formulas for finding SN are: 1) SN = n/2 * (2a + (n - 1) * d), where 'a' is the first term and 'd' is the common difference; and 2) SN = n/2 * (a + l), where 'l' is the last term.

How can you derive the formula for SN from the arithmetic sequence?

To derive the formula, you start by adding the first and last terms of the series. Then, this sum is multiplied by the number of terms (n), and finally, you divide by 2 to get the average sum of the first and last terms.

In the first example, how do we find the sum of the first 9 terms of the arithmetic series?

In the first example, we are given the first three terms of the sequence and need to determine the common difference. Once the common difference is found, we use the formula SN = n/2 * (2a + (n - 1) * d) to find S9.

Why is it important to identify the common difference in an arithmetic sequence?

The common difference is crucial because it allows us to determine how each term is related to the next one in the sequence. It is essential for calculating the sum of terms using the SN formula.

In the second example, how do we solve for the sum of the first 21 terms when given certain specific terms of the sequence?

In the second example, we use the given terms (u7 = 16 and u3 + u9 = 24) to set up equations for the first term and the common difference. After solving for these variables, we apply the SN formula to find the sum of the first 21 terms.

How is the process of finding the 20th term in the sequence simplified in the third example?

In the third example, we use the formula UN = SN - SN-1 to find the 20th term. By calculating S20 and S19, we can determine U20, the 20th term of the sequence.