Ecuaciones diferenciales Homogéneas | Ejemplo 5
Summary
TLDRThis educational video script offers a detailed walkthrough of solving a homogeneous second-order differential equation. The instructor begins by recommending viewers watch introductory videos for context. The script then guides through the process of identifying the equation as homogeneous, separating variables, and transforming it into a solvable form. It covers the method of substitution, simplification, and integration, ultimately leading to the solution with a constant of integration. The instructor emphasizes the importance of practice and invites viewers to engage with more content for a deeper understanding of differential equations.
Takeaways
- 📚 The video is a tutorial on solving homogeneous differential equations, specifically for viewers who are new to the topic.
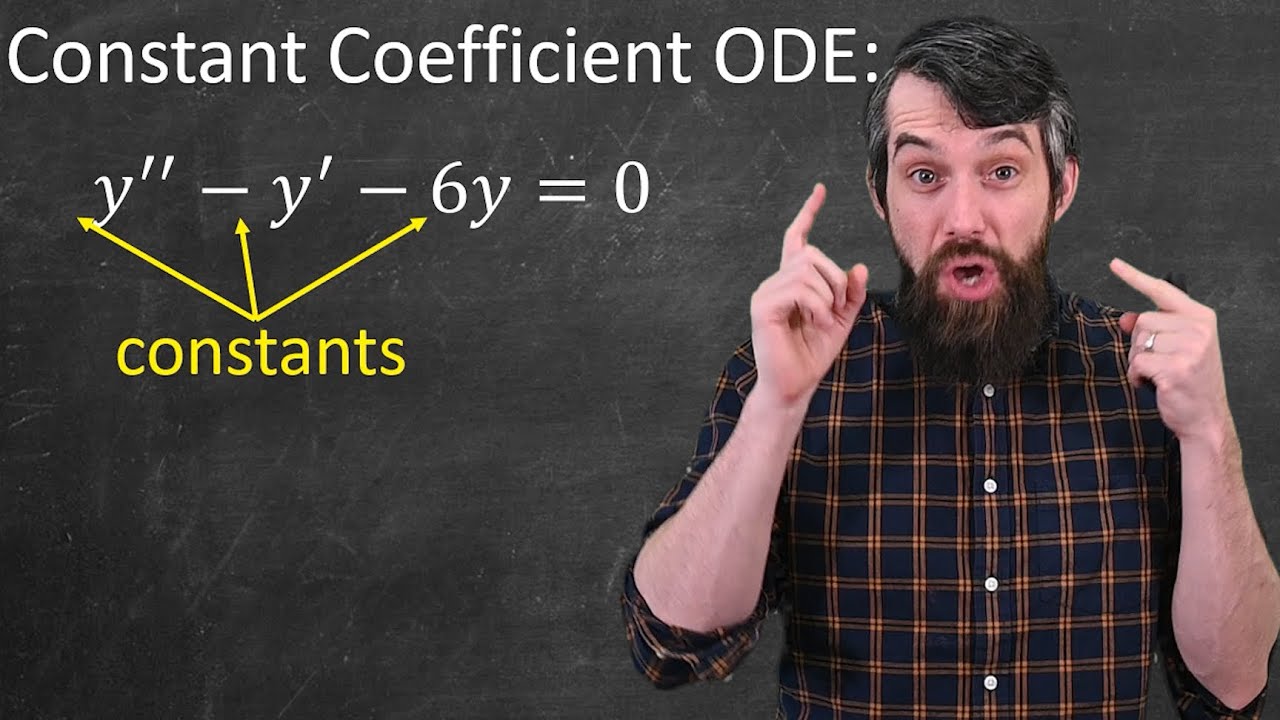
- 🔍 The script emphasizes checking if an equation is homogeneous and of the first degree before attempting to solve it.
- 📝 The first step in solving the equation is to separate the differentials, which involves moving terms involving 'dx' to one side and 'dy' to the other.
- 🔄 The process includes a substitution technique to transform the homogeneous equation into a separable variables equation.
- ✍️ The script provides a detailed walkthrough of the algebraic manipulations needed to simplify the equation, such as factoring and combining like terms.
- 📉 The video demonstrates the integration process, including handling of inverse trigonometric integrals and logarithmic integrals.
- 🔍 It highlights the importance of recognizing when an integral can be solved by substitution or parts, and when it requires a different method.
- 📐 The tutorial includes a step to eliminate fractions by multiplying through by the denominator, simplifying the equation.
- 📝 The final step involves back-substitution to convert the solution back into the original variables, x and y.
- 📚 The presenter suggests practicing with the given example and comparing it with the solution provided in the video for better understanding.
- 👍 The video concludes with an encouragement for viewers to subscribe, comment, share, and like the video for further assistance and support.
Q & A
What is the main topic of the video?
-The main topic of the video is solving homogeneous differential equations.
What does the instructor recommend for viewers who are new to the topic?
-The instructor recommends that new viewers watch the introductory video on homogeneous differential equations before proceeding with this one.
How does the instructor verify if the given equation is homogeneous?
-The instructor verifies if the equation is homogeneous by checking if both terms in the equation have the same degree and if the functions in the numerator and denominator are homogeneous of the same degree.
What is the first step in solving a homogeneous differential equation according to the script?
-The first step is to separate the differentials, which involves moving the differential of x to the other side of the equation and multiplying the terms accordingly.
What substitution does the instructor suggest to transform the homogeneous equation into a separable variables equation?
-The instructor suggests substituting the variable 'y' with 'u' (or any letter with a hat, like 'u^'), and replacing the differential 'dy' with the differential of the new variable.
How does the instructor approach the process of integrating after separating the variables?
-The instructor integrates by first simplifying the equation, then identifying direct integrations and inverse trigonometric integrals, and applying the appropriate integration techniques.
What is the significance of the instructor's observation about the terms having the same variable?
-The observation about terms having the same variable is significant for factorization, which simplifies the equation and makes it easier to integrate.
How does the instructor handle the presence of fractions in the integrated equation?
-The instructor eliminates fractions by multiplying the entire equation by the denominator, which simplifies the equation and removes the need for fractions.
What technique does the instructor use to simplify the final answer involving logarithms and arctangents?
-The instructor uses the property of logarithms that allows combining multiple logarithms into a single logarithm with the arguments multiplied together.
Why does the instructor suggest reviewing the final answer for any remaining substituted variables?
-The instructor suggests reviewing the final answer to ensure that all substituted variables have been correctly replaced back to their original form, ensuring the solution is in terms of the original variables.
What does the instructor recommend for viewers who want to practice solving homogeneous differential equations?
-The instructor recommends that viewers practice by watching the next video, pausing it to attempt the exercise, and then comparing their work with the instructor's explanation.
Outlines

此内容仅限付费用户访问。 请升级后访问。
立即升级Mindmap

此内容仅限付费用户访问。 请升级后访问。
立即升级Keywords

此内容仅限付费用户访问。 请升级后访问。
立即升级Highlights

此内容仅限付费用户访问。 请升级后访问。
立即升级Transcripts

此内容仅限付费用户访问。 请升级后访问。
立即升级5.0 / 5 (0 votes)