Contoh Perhitungan Analisa Balok Anak dgn Plastik Sempurna (Leleh Umum) | Struktur Baja | Lightboard
Summary
TLDRThis video tutorial dives into the process of designing a simple beam under uniform load, focusing on flexural strength. The presenter explains how to calculate the beam's strength, the load it can bear, and whether it is strong enough to support the given forces. Using an example of a six-meter-long beam, the tutorial covers concepts like the ultimate load, plastic section modulus, and the ultimate moment in structural analysis. The video also emphasizes how understanding these calculations can simplify designing beams that undergo plastic deformation. Viewers can learn key structural engineering principles and apply them to real-world scenarios.
Takeaways
- 😀 The video focuses on designing a bending beam (balok lentur) and calculating its strength to determine if it can carry the applied load.
- 😀 The beam in the example is a simple beam with a length of 6 meters, supported at both ends, and subjected to a uniform load of 4000 kg per meter.
- 😀 The load provided is the ultimate load, which is already the combination of dead load and live load.
- 😀 The beam is assumed to have sufficient lateral support, and local buckling is not a concern, allowing for plastic behavior.
- 😀 The formula for calculating the beam’s ultimate strength involves the plastic section modulus (Zcx) and the yield strength (Fy) of the material.
- 😀 The plastic section modulus (Zcx) for the selected WX350 beam is 6.8, and the yield strength for the steel (BJ-37) is 244 N/mm².
- 😀 The calculation involves converting units to ensure consistency, with results expressed in kN·m for practical use.
- 😀 Using the formula, the beam’s ultimate bending strength (Mbn) was calculated to be 187.5 kN·m.
- 😀 The ultimate moment (Mu) for the beam under the applied load was calculated using a simple structural analysis, resulting in 180 kN·m.
- 😀 Since the ultimate bending strength (Mbn) is greater than the applied moment (Mu), the beam is considered strong enough to carry the load.
- 😀 The example highlights the simplicity of calculating bending strength when the beam is designed to experience perfect plastic deformation, making it easier to determine its adequacy for load-bearing in structures like steel framed buildings.
Q & A
What is the focus of the video in terms of structural analysis?
-The video focuses on designing and analyzing the strength of a simple beam, specifically how to determine whether the beam can withstand a given load. It covers bending strength, ultimate load calculations, and ensuring the beam's structural safety.
What type of beam is being discussed in the example?
-The example discusses a simple beam with a length of 6 meters, supported at two points (a pin support and a roller support), subjected to a uniform load of 4000 kg per meter.
What does 'ultimate load' mean in the context of this example?
-The ultimate load refers to the maximum load a structure can carry, taking into account both dead load and live load in their most critical combined form. In this case, the 4000 kg per meter is an ultimate load.
What is the significance of the 'plastic section modulus' in the calculations?
-The plastic section modulus (Zcx) is used to calculate the plastic moment capacity of the beam. It is a measure of the beam's ability to resist bending and helps determine the beam's maximum strength under bending loads.
How is the moment of resistance (M) calculated for the beam?
-The moment of resistance (M) is calculated using the formula 0.9 * Zcx * Fb, where Zcx is the plastic section modulus and Fb is the yield strength of the material. The resulting moment is compared with the ultimate moment the beam is expected to carry.
What material is the beam made of in the example?
-The beam is made of steel, specifically the material grade BJ-37, which has a yield strength (Fb) of 244 Newton per millimeter squared.
Why is it necessary to convert the units during the calculation?
-Unit conversion is necessary to ensure consistency in the units used across all parameters. For example, the plastic section modulus is in mm³, and it needs to be converted to a consistent unit like kN·m for easier comparison with other values such as the ultimate moment.
What is the formula used to calculate the ultimate moment of the beam?
-The formula for calculating the ultimate moment (Mu) for a simply supported beam under a uniform load is Mu = 8 * Q * L² / 10, where Q is the uniform load (4000 kg/m) and L is the length of the beam (6 meters).
What does the comparison of the left and right sides of the equation determine?
-The comparison of the left side (the moment resistance, M) and the right side (the ultimate moment, Mu) determines if the beam is strong enough to withstand the applied load. If the moment resistance is greater than or equal to the ultimate moment, the beam is considered safe.
How can this analysis be applied in real-world structures?
-This analysis is useful for designing beams in real-world buildings, especially in structures where a simple beam is subjected to various loads. It helps engineers ensure that beams can safely carry the anticipated loads, such as in building frames or bridge supports.
Outlines

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowMindmap

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowKeywords

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowHighlights

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowTranscripts

This section is available to paid users only. Please upgrade to access this part.
Upgrade NowBrowse More Related Video

Expt 2. Flexural Strength Test of Concrete Beam
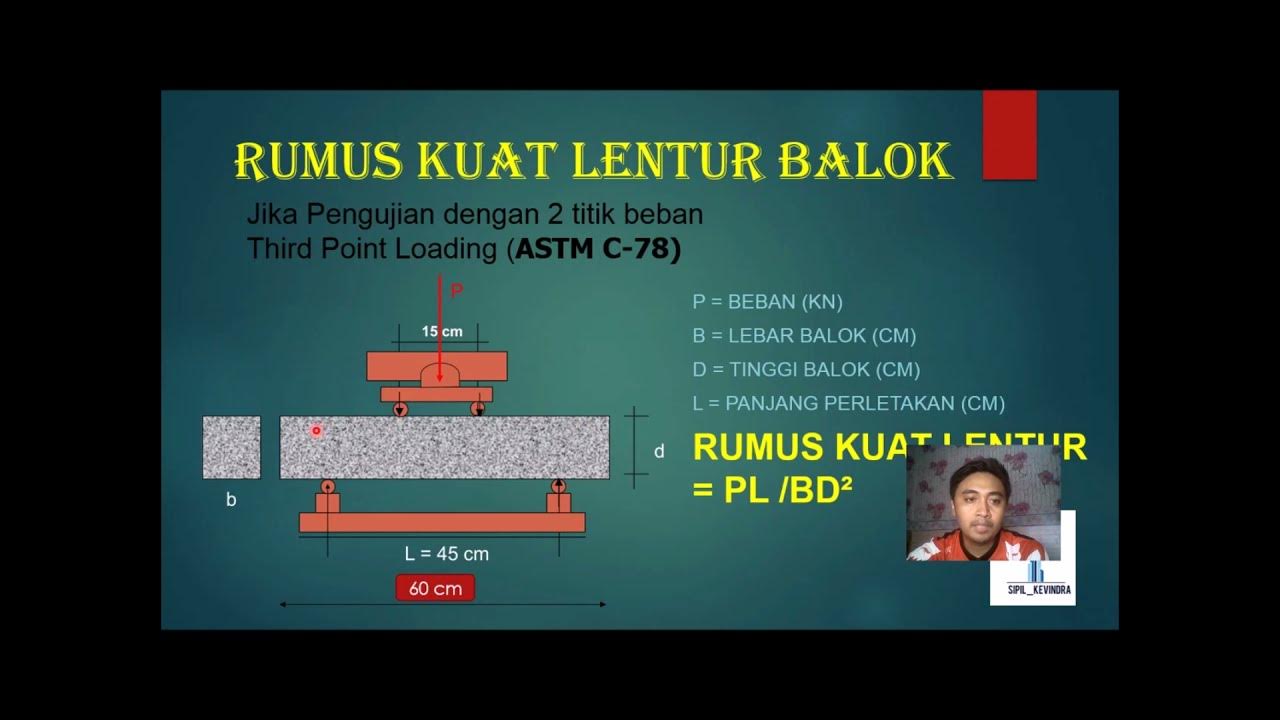
PENGUJIAN TES KUAT LENTUR BETON
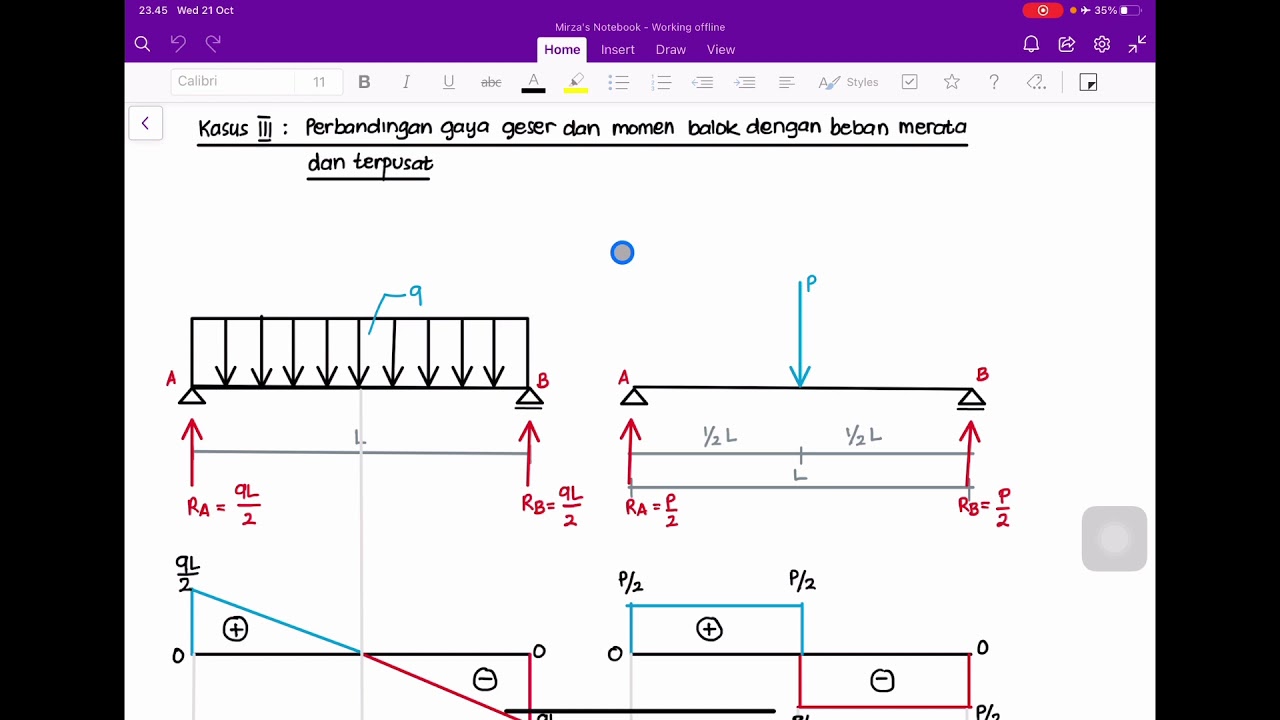
Mekanika Statis Tentu: Perbandingan Gaya Geser Dan Momen Pada Balok Dengan Beban Merata Dan Terpusat

Pemeriksaan Kuat Lentur Kayu

Steel Structures: Analysis/Design Course using MIDAS GEN - SIMPLE STEEL TRUSS SHED (Part 1)

Balok Lentur dengan Tekuk Lokal Menurut SNI 1729-2015 | Struktur Baja | Lightboard
5.0 / 5 (0 votes)