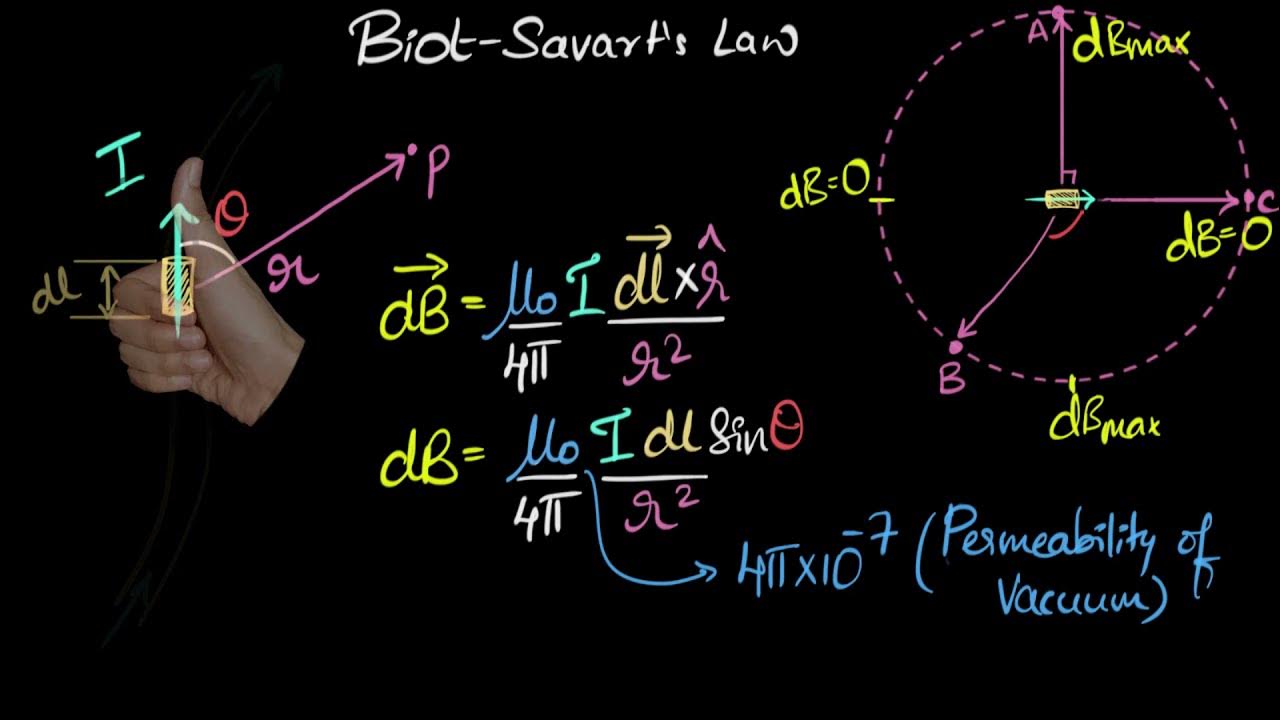
Derivation of the Biot-Savart law and calculating the magnetic field of a long straight wire.

Summary

In this video, the derivation of the Biot-Savart law for the magnetic field due to a current in a long straight wire is presented. Starting with the idea that current consists of moving charges, the magnetic field is calculated by applying the law of a moving point charge to infinitesimal segments of the wire. Through integration and trigonometric substitution, the magnetic field formula for a long straight wire is derived, showing that it is directly proportional to the current and inversely proportional to the distance from the wire. This result forms the foundation for more advanced concepts like Ampère's Law.

Takeaways

• 😀 The video builds upon a previous one where a magnetic field expression due to a moving point charge was derived.
• 😀 The goal of this video is to derive the Biot-Savart law for the magnetic field due to a current, which is essentially a collection of moving charges.
• 😀 The approach taken in the video is a standard method from introductory physics textbooks but is considered a naive approximation for the magnetic field of a moving point charge.
• 😀 The derivation involves modeling a long straight wire as a series of tiny moving charges, each with an infinitesimal charge (DQ), which move with drift velocity.
• 😀 The drift velocity (VD) of charge carriers in the wire is crucial in this derivation and relates to the current through the formula: I = n * Q * A * VD.
• 😀 The magnetic field contribution (DB) from each infinitesimal charge (DQ) is calculated using the formula for a moving point charge's magnetic field.
• 😀 The magnetic field contribution direction is determined using the right-hand rule (V cross R), which helps visualize the direction of the magnetic field at a given observation point.
• 😀 The differential form of the Biot-Savart law is derived, which allows for computing the magnetic field due to a current-carrying wire.
• 😀 The example provided for applying the Biot-Savart law focuses on a long straight wire, and the magnetic field calculation leads to a trigonometric substitution integral.
• 😀 A detailed trigonometric substitution is performed, and the final result for the magnetic field around a long straight wire at distance 's' is found to be μI / 2πs.
• 😀 This result for the magnetic field of a long straight wire is a foundational result in electromagnetism and is critical for understanding Ampère's law, which is analogous to Gauss's law for electrostatics.

Q & A

What is the goal of this video?

The goal of the video is to derive the Biot-Savart law for the magnetic field due to a current and apply it to calculate the magnetic field around a long straight wire.

What is the main disclaimer made in the video regarding the derivation?

The video mentions that the approach being used is the standard one found in introductory physics textbooks, but it also points out that the Biot-Savart law for a moving charge is a naive approximation and not the real story until advanced courses are taken.

What is the significance of the drift velocity (V_d) in the derivation?

The drift velocity represents the average velocity of charge carriers in the wire, and it's used in the derivation to calculate the magnetic field contribution due to the motion of these charges.

What does the Biot-Savart law help us compute?

The Biot-Savart law helps us compute the magnetic field contribution from a small slice of a current-carrying wire, and by integrating these contributions, we can determine the magnetic field due to the entire wire.

How is the differential element of charge (DQ) related to the current in the wire?

The differential charge element (DQ) is related to the current by the formula DQ = n * Q * A * V_d * DL, where n is the charge carrier density, Q is the charge of each carrier, A is the cross-sectional area of the wire, and V_d is the drift velocity.

Why is it important to use the differential form of the Biot-Savart law?

Using the differential form allows us to break down the current-carrying wire into infinitesimally small segments, each contributing a small magnetic field that can be summed up through integration.

What is the relationship between the position vector (R) and the angle theta in the diagram?

The position vector (R) points from the source charge to the observation point, and the angle theta is the angle between this position vector and the direction of the velocity of the moving charge.

What is the significance of the integral involving x^2 + s^2 to the 3/2 power?

This integral represents the calculation of the magnetic field due to a current in a long straight wire. The denominator, x^2 + s^2 raised to the 3/2 power, arises from the geometry of the system and requires a trigonometric substitution to simplify the calculation.

What happens when the integral is transformed using a trigonometric substitution?

The trigonometric substitution simplifies the denominator of the integral, allowing for a straightforward integration. The substitution x = s * tan(θ) leads to a more manageable form, ultimately enabling the calculation of the magnetic field.

What is the final expression for the magnetic field around a long straight wire?

The final expression for the magnetic field at a distance 's' from a long straight wire carrying current 'I' is given by B = μ₀ * I / (2π * s), where μ₀ is the permeability of free space.